Synthetic differential geometry
Geometers like S. Lie, E. Cartan and their contemporaries explicitly used infinitesimal real numbers, infinitesimal curves, etc. and Lie referred to methods based on infinitesimals as "synthetic" , as opposed to "analytic" (cf. [a1]). In present day mathematics, based on set-theoretic foundations, such infinitesimal reals do not exist, and the synthetic methods cannot be made mathematically rigorous in a direct way.
The phrase "synthetic differential geometry" usually refers to a development initiated by F.W. Lawvere's 1967 lecture (later published as [a2]). This development is based on category-theoretic rather than set-theoretic foundations, and is compatible with infinitesimals. For example, one of the basic axioms (often called the Kock–Lawvere axiom) states that for the subobject 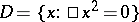 of the line
of the line 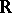 the mapping
the mapping 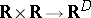 , sending
, sending 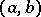 to the "infinitesimal" straight line
to the "infinitesimal" straight line 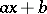 , is an isomorphism. Thus, the derivative of a function
, is an isomorphism. Thus, the derivative of a function  at a point
at a point 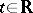 can be defined in a purely algebraic way as the unique number
can be defined in a purely algebraic way as the unique number 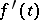 for which
for which 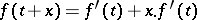 for all
for all 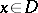 ; no limits are involved in this definition. Much of "infinitesimal" differential geometry, such as the theory of connections, curvature, etc., can be similarly developed in a purely algebraic fashion, by explicitly using infinitesimals. It is remarkable that in the context of synthetic differential geometry infinitesimal arguments such as those by Cartan literally make sense, and are mathematically rigorous.
; no limits are involved in this definition. Much of "infinitesimal" differential geometry, such as the theory of connections, curvature, etc., can be similarly developed in a purely algebraic fashion, by explicitly using infinitesimals. It is remarkable that in the context of synthetic differential geometry infinitesimal arguments such as those by Cartan literally make sense, and are mathematically rigorous.
The relation with ordinary differential geometry is established via suitable models, as presented in [a3] and [a4]. These models are Grothendieck topoi (cf. Topos), much like the ones in algebraic geometry, but based on rings of smooth functions on manifolds, and quotients of such rings by ordinary ideals.
Following Lawvere, every topos can be viewed as a universe of sets, with an intrinsic logic which is intuitionistic. Thus, it is possible to give foundations of synthetic differential geometry which are based on intuitionistic set theory rather than on category theory (this is done in [a5]).
It should be emphasized that the infinitesimals used in synthetic differential geometry are generally nilpotent, and hence cannot be accounted for in Robinson's non-standard analysis. The compatibility of non-standard analysis with synthetic differential geometry is demonstrated in [a4].
For detailed expositions of various aspects of synthetic differential geometry see [a5], [a7], [a8].
References
| [a1] | S. Lie, "Allgemeine Theorie der partiellen Differentialgleichungen erster Ordnung" Math. Ann. , 9 (1876) pp. 245–296 |
| [a2] | F.W. Lawvere, "Categorical dynamics" A. Kock (ed.) , Topos theoretic methods in geometry , Aarhus Univ. (1979) pp. 1–28 |
| [a3] | E. Dubuc, "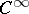 -schemes" Amer. J. Math. , 103 (1981) pp. 683–690 -schemes" Amer. J. Math. , 103 (1981) pp. 683–690 |
| [a4] | I. Moerdijk, G.E. Reyes, "A smooth version of the Zariski topos" Adv. Math. , 65 (1987) pp. 229–253 |
| [a5] | R. Lavendhomme, "Leçons de la géométrie différentielle synthétique naive" , Univ. Louvain (1987) |
| [a6] | A. Robinson, "Non-standard analysis" , North-Holland (1966) |
| [a7] | A. Kock, "Synthetic differential geometry" , Cambridge Univ. Press (1981) |
| [a8] | I. Moerdijk, G.E. Reyes, "Models for smooth infinitesimal analysis" , Springer (1991) |
Synthetic differential geometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Synthetic_differential_geometry&oldid=15789