Oscillating matrix
oscillatory matrix
A totally non-negative matrix 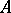 for which there exists a positive integer
for which there exists a positive integer 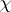 such that
such that 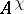 is a totally positive matrix; the matrix
is a totally positive matrix; the matrix 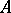 is called totally non-negative (totally positive) if all its minors, of whatever order, are non-negative (positive). The lowest exponent
is called totally non-negative (totally positive) if all its minors, of whatever order, are non-negative (positive). The lowest exponent 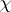 is called the exponent of the oscillating matrix. If
is called the exponent of the oscillating matrix. If 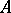 is an oscillating matrix with exponent
is an oscillating matrix with exponent 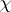 , then for any integer
, then for any integer 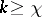 the matrix
the matrix  is totally positive; an integer positive power of an oscillating matrix and the matrix
is totally positive; an integer positive power of an oscillating matrix and the matrix 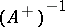 are also oscillating matrices. In order that a totally non-negative matrix
are also oscillating matrices. In order that a totally non-negative matrix 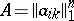 is an oscillating matrix, it is necessary and sufficient that: 1)
is an oscillating matrix, it is necessary and sufficient that: 1)  is a non-singular matrix; and 2) for
is a non-singular matrix; and 2) for 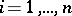 , the following are fulfilled:
, the following are fulfilled: 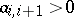 ,
, 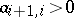 .
.
The basic theorem on oscillating matrices is: An oscillating matrix 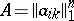 always has
always has  different positive eigen values; for the eigen vector
different positive eigen values; for the eigen vector  that corresponds to the largest eigen value
that corresponds to the largest eigen value 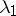 , all coordinates differ from zero and are of the same sign; for an eigen vector
, all coordinates differ from zero and are of the same sign; for an eigen vector  that corresponds to the
that corresponds to the  -th eigen value
-th eigen value  (arranged according to decreasing value) there are exactly
(arranged according to decreasing value) there are exactly 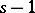 changes of sign; for any real numbers
changes of sign; for any real numbers  ,
, 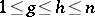 ,
,  , the number of changes of sign in the sequence of coordinates of the vector
, the number of changes of sign in the sequence of coordinates of the vector  is between
is between  and
and 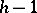 .
.
References
| [1] | F.R. Gantmakher, M.G. Krein, "Oscillation matrices and kernels and small vibrations of mechanical systems" , Dept. Commerce USA. Joint Publ. Service (1961) (Translated from Russian) |
Comments
References
| [a1] | S. Karlin, "Total positivity" , Stanford Univ. Press (1960) |
| [a2] | F.R. [F.R. Gantmakher] Gantmacher, "The theory of matrices" , 2 , Chelsea, reprint (1959) pp. Chapt. XIII, §9 (Translated from Russian) |
Oscillating matrix. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Oscillating_matrix&oldid=13884