Harmonic mapping
A smooth mapping  between Riemannian manifolds (cf. Riemannian manifold) is harmonic if it is an extremal (or critical point) of the energy functional
between Riemannian manifolds (cf. Riemannian manifold) is harmonic if it is an extremal (or critical point) of the energy functional
 |
where 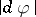 is the Hilbert–Schmidt norm of the differential, computed with respect to the metrics
is the Hilbert–Schmidt norm of the differential, computed with respect to the metrics  and
and 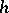 , and
, and 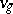 is the Riemannian volume element.
is the Riemannian volume element.
The mapping 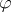 is harmonic if it satisfies the Euler–Lagrange equation
is harmonic if it satisfies the Euler–Lagrange equation 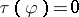 , where the tension field
, where the tension field 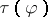 is given by
is given by  ,
,  denoting the natural connection on
denoting the natural connection on 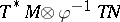 .
.
In local coordinate systems 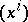 on
on  and
and 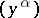 on
on 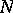 , one has
, one has
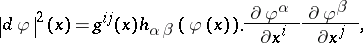 |
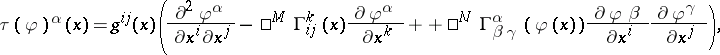 |
where the 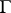 are the Christoffel symbols of the Levi–Civita connections on
are the Christoffel symbols of the Levi–Civita connections on 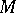 and
and 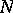 . The Euler–Lagrange equation is therefore a semi-linear elliptic system of partial differential equations.
. The Euler–Lagrange equation is therefore a semi-linear elliptic system of partial differential equations.
Harmonic mappings include as special cases the closed geodesics in a Riemannian manifold  , the minimal immersions, the totally geodesic mappings and the holomorphic mappings between Kähler manifolds. In physics, they are related to
, the minimal immersions, the totally geodesic mappings and the holomorphic mappings between Kähler manifolds. In physics, they are related to 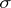 -models and to some types of liquid crystals.
-models and to some types of liquid crystals.
The systematic study of harmonic mappings was initiated in 1964 in [a7] by J. Eells and J. Sampson.
A detailed exposition of results obtained before 1988 can be found in [a5] and [a6] and includes the following four main directions:
existence theory for harmonic mappings in prescribed homotopy classes (with existence and non-existence results);
regularity and partial regularity for minimizers of the energy in appropriate Sobolev spaces (with restriction on the Hausdorff dimension of the singular set);
explicit constructions of harmonic mappings from the two-dimensional sphere to Lie groups, symmetric spaces and loop groups in terms of holomorphic mappings and twistor constructions;
applications of the existence theory of harmonic mappings to the study of the geometry of real manifolds (curvature pinching, rigidity), or of Kähler manifolds (rigidity, uniformization), applications to the study of Teichmüller spaces (cf. Discrete subgroup; Teichmüller space; Riemannian geometry in the large).
Further developments (up to 1997) include the following:
Application of harmonic mappings to (Mostow) rigidity of manifolds was pursued in [a4] and [a15], the latter unifying previous results. In a similar vein, existence of harmonic mappings bears on the structure of the fundamental group of Kähler manifolds ([a3], see also [a1]).
A new direction was opened in [a11], in which the notion of harmonic mapping was extended to more general spaces (trees, polyhedra, Tits buildings), and an existence result was proved and applied to the study of 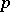 -adic superrigidity for lattices in groups of rank one.
-adic superrigidity for lattices in groups of rank one.
Further curvature pinching theorems were obtained in [a13] and [a19].
Various results on Teichmüller spaces were obtained, using classical harmonic mappings (see [a17]) or harmonic maps into trees (see [a18]).
The question of regularity or partial regularity was extended to weakly harmonic mappings (as opposed to minimizers) in the appropriate Sobolev space: when 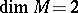 , any weakly harmonic mapping is smooth [a12], and examples show that for
, any weakly harmonic mapping is smooth [a12], and examples show that for  , the Hausdorff dimension of the singular set is not restricted [a16].
, the Hausdorff dimension of the singular set is not restricted [a16].
Examples show that harmonic mappings homotopic to homeomorphisms are not always homeomorphisms, even when the curvature of the range is negative [a8].
Explicit constructions of harmonic mappings of surfaces into symmetric spaces in terms of holomorphic constructions or totally integrable systems were further developed, e.g. in [a14] and [a2] (see [a9], [a10]).
References
| [a1] | J. Amorós, M. Burger, K. Corlette, D. Kotschick, D. Toledo, "Fundamental groups of compact Kähler manifolds" , Math. Surveys Monogr. , 44 , Amer. Math. Soc. (1996) |
| [a2] | F.E. Burstall, D. Ferus, F. Pedit, U. Pinkall, "Harmonic tori in symmetric spaces and commuting Hamiltonian systems on loop algebras" Ann. of Math. , 138 (1993) pp. 173–212 |
| [a3] | J.A. Carlson, D. Toledo, "Harmonic maps of Kähler manifolds to locally symmetric spaces" Publ. Math. IHES , 69 (1989) pp. 173–201 |
| [a4] | K. Corlette, "Archimedean superrigidity and hyperbolic geometry" Ann. of Math. , 135 (1992) pp. 165–182 |
| [a5] | J. Eells, L. Lemaire, "A report on harmonic maps" Bull. London Math. Soc. , 10 (1978) pp. 1–68 |
| [a6] | J. Eells, L. Lemaire, "Another report on harmonic maps" Bull. London Math. Soc. , 20 (1988) pp. 385–524 |
| [a7] | J. Eells, J. Sampson, "Harmonic mappings of Riemannian manifolds" Amer. J. Math. , 86 (1964) pp. 109–160 |
| [a8] | F.T. Farrell, L.E. Jones, "Some non-homeomorphic harmonic homotopy equivalences" Bull. London Math. Soc. , 28 (1996) pp. 177–180 |
| [a9] | "Harmonic maps and integrable systems" A.P. Fordy (ed.) J.C. Wood (ed.) , Aspects of Math. , 23 , Vieweg (1994) |
| [a10] | M.A. Guest, "Harmonic maps, loop groups, and integrable systems" , London Math. Soc. Student Texts , 38 , Cambridge Univ. Press (1997) |
| [a11] | M. Gromov, R. Schoen, "Harmonic maps into singular spaces and 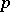 -adic superrigidity for lattices in groups of rank one" Publ. Math. IHES , 76 (1992) pp. 165–246 -adic superrigidity for lattices in groups of rank one" Publ. Math. IHES , 76 (1992) pp. 165–246 |
| [a12] | F. Hélein, "Régularité des applications faiblement harmoniques entre une surface et une variété riemannienne" C.R. Acad. Sci. Paris Ser. I , 312 (1991) pp. 591–596 |
| [a13] | L. Hernandez, "Kähler manifolds and  pinching" Duke Math. J. , 62 (1991) pp. 601–611 pinching" Duke Math. J. , 62 (1991) pp. 601–611 |
| [a14] | N. Hitchin, "Harmonic maps from a  -torus to the -torus to the  -sphere" J. Diff. Geom. , 31 (1990) pp. 627–710 -sphere" J. Diff. Geom. , 31 (1990) pp. 627–710 |
| [a15] | N. Mok, Y.-T. Siu, S.-K. Yeung, "Geometric superrigidity" Invent. Math. , 113 (1993) pp. 57–83 |
| [a16] | T. Rivière, "Applications harmoniques de 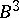 dans dans 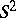 partout discontinues" C.R. Acad. Sci. Paris Ser. I , 314 (1992) pp. 719–723 partout discontinues" C.R. Acad. Sci. Paris Ser. I , 314 (1992) pp. 719–723 |
| [a17] | A.J. Tromba, "Teichmüller theory in Riemannian geometry" , ETH Lectures , Birkhäuser (1992) |
| [a18] | M. Wolf, "On realizing measured foliations via quadratic differentials of harmonic maps to 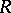 -trees" J. Anal. Math. , 68 (1996) pp. 107–120 -trees" J. Anal. Math. , 68 (1996) pp. 107–120 |
| [a19] | S.-T Yau, F. Zheng, "Negatively 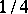 -pinched Riemannian metric on a compact Kähler manifold" Invent. Math. , 103 (1991) pp. 527–536 -pinched Riemannian metric on a compact Kähler manifold" Invent. Math. , 103 (1991) pp. 527–536 |
Harmonic mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Harmonic_mapping&oldid=14710