Extension of a Lie algebra
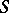 with kernel
with kernel 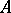
A Lie algebra 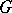 with an epimorphism
with an epimorphism  whose kernel is an ideal
whose kernel is an ideal 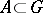 . This is equivalent to specifying an exact sequence
. This is equivalent to specifying an exact sequence
 |
The extension is said to split if there is a subalgebra 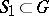 such that
such that 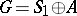 (direct sum of modules). Then
(direct sum of modules). Then  induces an isomorphism
induces an isomorphism 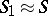 , and defines an action of the algebra
, and defines an action of the algebra 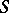 on
on 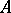 by derivations. Conversely, any homomorphism
by derivations. Conversely, any homomorphism 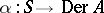 , where
, where 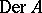 is the algebra of derivations of
is the algebra of derivations of 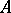 , uniquely determines a split extension
, uniquely determines a split extension 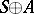 with multiplication given by
with multiplication given by
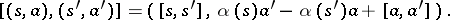 |
For finite-dimensional Lie algebras over a field of characteristic 0, Lévy's theorem holds: If  is semi-simple, then every extension of
is semi-simple, then every extension of 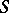 splits.
splits.
Of all non-split extensions, the Abelian ones have been studied most, i.e. the extensions with an Abelian kernel 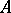 . In this case the action of
. In this case the action of 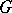 on
on  induces an action of
induces an action of 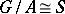 on
on 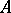 , that is,
, that is, 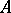 is an
is an 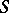 -module. For Lie algebras over a field, every Abelian extension of
-module. For Lie algebras over a field, every Abelian extension of 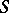 with as kernel an
with as kernel an 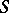 -module
-module 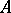 has the form
has the form 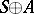 with multiplication given by
with multiplication given by
 |
where 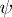 is some linear mapping
is some linear mapping 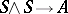 . The Jacobi identity is equivalent to the fact that
. The Jacobi identity is equivalent to the fact that 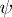 is a two-dimensional cocycle (or
is a two-dimensional cocycle (or  -cocycle, see Cohomology of Lie algebras). The extensions determined by cohomologous cocycles are equivalent in a natural sense. In particular, an extension is split if and only if
-cocycle, see Cohomology of Lie algebras). The extensions determined by cohomologous cocycles are equivalent in a natural sense. In particular, an extension is split if and only if 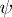 is cohomologous to zero. Thus, the Abelian extensions of an algebra
is cohomologous to zero. Thus, the Abelian extensions of an algebra 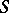 with kernel
with kernel  are described by the cohomology group
are described by the cohomology group 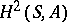 . The study of extensions with solvable kernel reduces to the case of Abelian extensions.
. The study of extensions with solvable kernel reduces to the case of Abelian extensions.
References
| [1] | N. Jacobson, "Lie algebras" , Interscience (1962) ((also: Dover, reprint, 1979)) |
Extension of a Lie algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Extension_of_a_Lie_algebra&oldid=13207