Dixmier mapping

Dixmier map

A mapping first defined for nilpotent Lie algebras by J. Dixmier in 1963 [a6], based on the orbit method of A.A. Kirillov [a12]. In 1966, Dixmier extended his definition to solvable Lie algebras [a7] (here and below, all Lie algebras are of finite dimension over an algebraically closed field of characteristic zero, cf. also Lie algebra; Lie algebra, solvable).

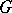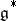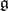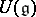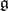The Dixmier mapping is an equivariant mapping with respect to the adjoint algebraic group from the dual space of a solvable Lie algebra into the space of primitive ideals of the enveloping algebra of (cf. also Universal enveloping algebra; the adjoint algebraic group 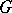of is the smallest algebraic subgroup of the group of automorphisms of the Lie algebra 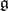whose Lie algebra in the algebra of endomorphisms of 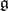contains the adjoint Lie algebra of 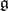). All ideals of 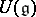are stable under the action of .

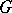The properties of the Dixmier mapping have been studied in detail. In particular, the Dixmier mapping allows one to describe (the) primitive ideals of the enveloping algebra 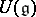and to describe the centre of .

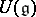The dual 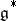of 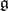is equipped with the Zariski topology and the space of primitive ideals of 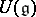with the Jacobson topology.

The Dixmier mapping (for solvable) is surjective [a9], injective (modulo the action of the adjoint algebraic group 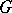) [a17], continuous [a5], and even open [a13].

Hence the Dixmier mapping induces a homeomorphism between 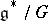and the space 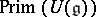of primitive ideals of 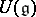and allows a complete classification of the primitive ideals of . The openness was an open question for quite a long while.

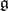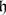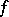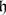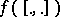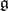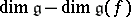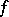The Dixmier construction goes as follows: If is a linear form on the Lie algebra , one chooses a subalgebra of which is a polarization of . This means that the subalgebra is an isotropic subspace of maximal dimension for the skew-symmetric bilinear form (on ); hence the dimension of is one half of , where is the stabilizer of in 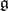with respect to the co-adjoint action of in .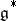

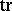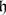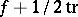For solvable Lie algebras such polarizations always exist, whereas for arbitrary Lie algebras this is, in general, not the case. Let denote the linear form on defined as the trace of the adjoint action of in . The linear form on 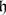defines a one-dimensional representation of the enveloping algebra 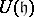. Let denote its kernel and 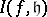the largest two-sided ideal in 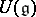contained in 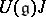. This is nothing else but the kernel of the so-called twisted induction from 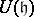to 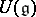of the one-dimensional representation of 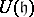given by 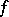. In the case of a nilpotent Lie algebra, the twist 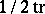is zero. The twisted induction on the level of enveloping algebras corresponds to the unitary induction on the level of Lie groups.

The ideal 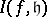obtained (in the solvable case) in this way is independent of the choice of the polarization [a7], hence this ideal may be denoted by 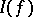. The ideal 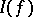is a (left) primitive ideal, i.e. the annihilator of an irreducible representation (left module) of 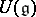. It is known that for enveloping algebras of Lie algebras, left and right primitive ideals coincide (see [a7] in the solvable case and [a14] in the general case). It should be noted that for solvable Lie algebras 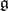all prime ideals (hence especially all primitive ideals) of 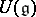are completely prime [a7].

For solvable Lie algebras 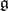, the Dixmier mapping associates to a linear form 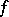of 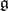this primitive ideal 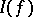. The 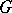-equivariance follows immediately from the fact that this construction commutes with automorphisms of 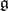. For a general description and references, see [a3] and [a8].

The definition has been extended in several directions:

1) To the Dixmier–Duflo mapping [a10], defined for all Lie algebras but only on the set of elements of having a solvable polarization. In particular, this set contains the open set of linear forms whose orbits under 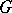have maximal dimension. For solvable Lie algebras one gets the usual definition.

2) To the -parameter Duflo mapping [a11]. This mapping is defined for algebraic Lie algebras 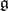(cf. also Lie algebra, algebraic). The first parameter is a so-called linear form on 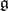of unipotent type, the second parameter is a primitive ideal in the reductive part of the stabilizer in 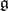of the first parameter. The mapping goes into the space of primitive ideals of 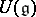. This mapping coincides with the Dixmier mapping if 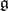is nilpotent and it can be related to the Dixmier mapping if is algebraic and solvable. For semi-simple, the mapping reduces to the identity. This -parameter Duflo mapping is surjective [a11] and it is injective modulo the operation of [a16].

3) The Dixmier mapping for 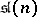. This was done by W. Borho, using the above Dixmier procedure [a1]. The problem is its being well-defined. Because of the twist in the induction, this Dixmier mapping for 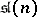(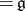) is continuous only on sheets of 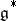but not as mapping in the whole. The sheets of 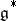are the maximal irreducible subsets of the space of linear forms whose 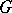-orbits have a fixed dimension. The Dixmier mapping for 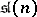is surjective on the space of primitive completely prime ideals of 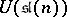[a15] and it is injective modulo [a4].

4) The Dixmier mapping on polarizable sheets (sheets in which every element has a polarization) in the semi-simple case. This was done by W. Borho. This map is well-defined [a2] and continuous, and it is conjectured to be injective modulo (the conjecture is still open, October 1999).

References

[a1]	W. Borho, "Definition einer Dixmier–Abbildung für " Invent. Math. , 40 (1977) pp. 143–169
[a2]	W. Borho, "Extended central characters and Dixmier's map" J. Algebra , 213 (1999) pp. 155–166
[a3]	W. Borho, P. Gabriel, R. Rentschler, "Primideale in Einhüllenden auflösbarer Lie–Algebren" , Lecture Notes Math. , 357 , Springer (1973)
[a4]	W. Borho, J.C. Jantzen, "Über primitive Ideale in der Einhüllenden einer halbeinfachen Lie-Algebra" Invent. Math. , 39 (1977) pp. 1–53
[a5]	N. Conze, M. Duflo, "Sur l'algèbre enveloppante d'une algèbre de Lie résoluble" Bull. Sci. Math. , 94 (1970) pp. 201–208
[a6]	J. Dixmier, "Représentations irréductibles des algèbres de Lie nilpotents" An. Acad. Brasil. Ci. , 35 (1963) pp. 491–519
[a7]	J. Dixmier, "Representations irreductibles des algebres de Lie résolubles" J. Math. Pures Appl. , 45 (1966) pp. 1–66
[a8]	J. Dixmier, "Enveloping algebras" , Amer. Math. Soc. (1996) (Translated from French)
[a9]	M. Duflo, "Sur les extensions des representations irreductibles des algèbres de Lie contenant un ideal nilpotent" C.R. Acad. Sci. Paris Ser. A , 270 (1970) pp. 504–506
[a10]	M. Duflo, "Construction of primitive ideals in enveloping algebras" I.M. Gelfand (ed.) , Lie Groups and their representations: Summer School of the Bolyai Janos Math. Soc. (1971) , Akad. Kiado (1975)
[a11]	M. Duflo, "Théorie de Mackey pour les groupes de Lie algébriques" Acta Math. , 149 (1982) pp. 153–213
[a12]	A.A. Kirillov, "Unitary representations of nilpotent Lie groups" Uspekhi Mat. Nauk , 17 (1962) pp. 57–110 (In Russian)
[a13]	O. Mathieu, "Bicontinuity of the Dixmier map" J. Amer. Math. Soc. , 4 (1991) pp. 837–863
[a14]	C. Moeglin, "Ideaux primitifs des algèbres enveloppantes" J. Math. Pures Appl. , 59 (1980) pp. 265–336
[a15]	C. Moeglin, "Ideaux primitifs completement premiers de l'algèbre enveloppante de 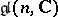" J. Algebra , 106 (1987) pp. 287–366
[a16]	C. Moeglin, R. Rentschler, "Sur la classification des ideaux primitifs des algèbres enveloppantes" Bull. Soc. Math. France , 112 (1984) pp. 3–40
[a17]	R. Rentschler, "L'injectivite de l'application de Dixmier pour les algèebres de Lie résolubles" Invent. Math. , 23 (1974) pp. 49–71