Differential operator on a module

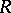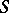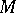A mapping of modules over a commutative ring which is an analogue of the concept of a differential operator. Let be a commutative ring, let be a subring of and let and be two 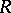-modules. A homomorphism of 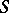-modules 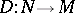is said to be a differential operator of order 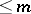, where 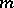is a non-negative integer, if for any the mapping 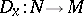defined by the formula

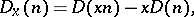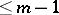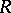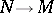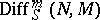is a differential operator of order . A differential operator of order zero is a homomorphism of -modules . The set of all differential operators of order forms a submodule of the 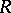-module of all homomorphisms of 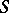-modules 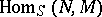. In particular,

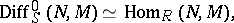and the quotient module

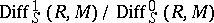is isomorphic to the module of 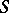-derivations 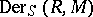of 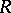with values in 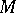. The union 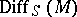of the increasing family of submodules

is a filtered associative ring with respect to the operation of composition of mappings. This ring is known as the ring of differential operators of the -module 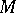over the subring 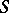, while the corresponding graded ring

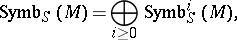where

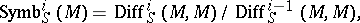is said to be the module of symbols. The image of a differential operator 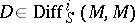in the ring is said to be the symbol of the differential operator.

If 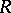is an algebra over the field of rational numbers and the module of differentials 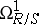is projective, then there exists an isomorphism between the 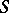-algebra 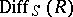and the enveloping algebra of the Lie algebra of -derivations 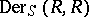. In this case the ring 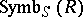is isomorphic to the symmetric algebra of the 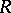-module .

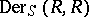For example, let 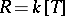be the ring of polynomials over a field 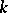; the mappings 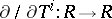, defined by the formula

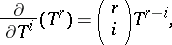are differential operators of 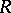over 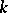of order 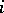. The ring of differential operators 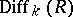is a free module over 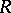with basis 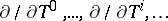. Multiplication is given by the formula

In particular,

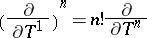(Taylor's formula) which, if the characteristic of 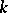is equal to zero, yields

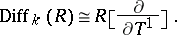If 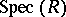is an affine group 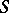-scheme, invariant differential operators of 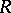may also be considered [2].

References

[1]	A.M. Vinogradov, I.S. Krasil'shchikov, "What is the Hamilton formalism?" Russian Math. Surveys , 30 : 1 (1975) pp. 177–202 Uspekhi Mat. Nauk. , 30 : 1 (1975) pp. 173–198
[2]	A. Grothendieck, "Eléments de géométrie algébrique. IV Etude locale des schémas et des morphisms des schémas I" Publ. Math. IHES , 20 (1960)
[3]	M. Demazure, P. Gabriel, "Groupes algébriques" , 1 , Masson (1970)
[4]	J.E. Björk, "The global homological dimension of some algebras of differential operators" Invent. Math. , 17 : 1 (1972) pp. 67–78