Cohomology group
of a cochain complex 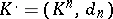 of Abelian groups
of Abelian groups
The graded group 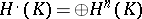 , where
, where 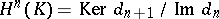 (see Complex). The group
(see Complex). The group 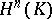 is called the
is called the  -dimensional, or the
-dimensional, or the  -th, cohomology group of the complex
-th, cohomology group of the complex  . This concept is dual to that of homology group of a chain complex (see Homology of a complex).
. This concept is dual to that of homology group of a chain complex (see Homology of a complex).
In the category of modules, the cohomology module of a cochain complex is also called a cohomology group.
The cohomology group of a chain complex  of
of 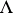 -modules with coefficients, or values, in
-modules with coefficients, or values, in 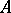 , where
, where 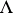 is an associative ring with identity and
is an associative ring with identity and 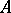 is a
is a  -module, is the cohomology group
-module, is the cohomology group
 |
of the cochain complex
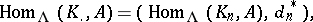 |
where 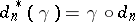 ,
, 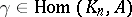 . A special case of this construction is the cohomology group of a polyhedron, the singular cohomology group of a topological space, and the cohomology groups of groups, algebras, etc.
. A special case of this construction is the cohomology group of a polyhedron, the singular cohomology group of a topological space, and the cohomology groups of groups, algebras, etc.
If 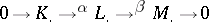 is an exact sequence of complexes of
is an exact sequence of complexes of 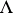 -modules, where the images of the
-modules, where the images of the 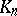 are direct factors in
are direct factors in 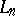 , the following exact sequence arises in a natural way:
, the following exact sequence arises in a natural way:
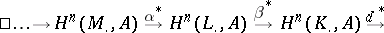 |
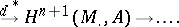 |
On the other hand, if 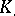 is a complex of
is a complex of 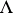 -modules, and all
-modules, and all 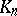 are projective, then with every exact sequence
are projective, then with every exact sequence 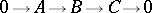 of
of 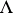 -modules is associated an exact sequence of cohomology groups:
-modules is associated an exact sequence of cohomology groups:
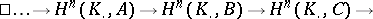 |
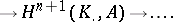 |
See Homology group; Cohomology (for the cohomology group of a topological space).
References
| [1] | H. Cartan, S. Eilenberg, "Homological algebra" , Princeton Univ. Press (1956) |
| [2] | R. Godement, "Topologie algébrique et théorie des faisceaux" , Hermann (1958) |
| [3] | S. MacLane, "Homology" , Springer (1963) |
Comments
The exact sequence of cohomology groups given above is often referred to as a long exact sequence of cohomology groups associated to a short exact sequence of complexes.
Cohomology group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cohomology_group&oldid=18382