Einstein-Smoluchowski equation
An integral equation for the probability density of the transition function  from a state
from a state 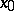 at a moment
at a moment  to a point
to a point  at a moment
at a moment  :
:
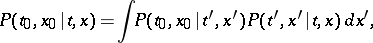 |
 |
The function  describes a stochastic process without after-effects (a Markov process), one characteristic feature of which is the independence of the evolution of the system from
describes a stochastic process without after-effects (a Markov process), one characteristic feature of which is the independence of the evolution of the system from  to
to 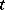 of its possible states preceding the moment
of its possible states preceding the moment  . The equation was formulated by M. von Smoluchowski (1906) in connection with the representation of Brownian motion as a stochastic process, and was developed simultaneously by him and A. Einstein. In the literature the Einstein–Smoluchowski equation is called the Kolmogorov–Chapman equation.
. The equation was formulated by M. von Smoluchowski (1906) in connection with the representation of Brownian motion as a stochastic process, and was developed simultaneously by him and A. Einstein. In the literature the Einstein–Smoluchowski equation is called the Kolmogorov–Chapman equation.
The physical analysis of a process of Brownian-motion type shows that it can be described by means of the function 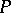 on intervals
on intervals 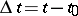 considerably larger than the correlation time of the stochastic process (even if
considerably larger than the correlation time of the stochastic process (even if 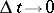 formally), and that the moments
formally), and that the moments
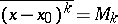 |
computed by means of this function must satisfy
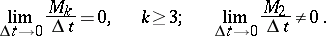 |
In this case the Einstein–Smoluchowski equation reduces to a linear differential equation of parabolic type, called the Fokker–Planck equation (see Kolmogorov equation; Diffusion process), for which the initial and boundary conditions are chosen in accordance with the specific problem considered.
References
| [1] | A. Einstein, M. von Smoluchowski, "Brownian motion" , Moscow-Leningrad (1936) (In Russian; translated from German) |
| [2] | S. Chandrasekhar, "Stochastic problems in physics and astronomy" Rev. Modern Physics , 15 (1943) pp. 1–89 |
| [3] | M. Kac, "Probability and related topics in physical sciences" , Proc. summer sem. Boulder, Col., 1957 , 1 , Interscience (1959) pp. Chapt. 4 |
Comments
The chain equation for the transition density of a Markov process is usually called the Chapman–Kolmogorov equation in the English literature. It was already introduced in 1900 by L. Bachelier, see [a1]. For references and discussion of the original work by Einstein and (von) Smoluchowski see the collection of papers reproduced in [a2]. The Fokker–Planck equation corresponds to Kolmogorov's forward differential equation [a3], Sect. 5.26. There exist non-Markovian processes satisfying the Chapman–Kolmogorov equation [a4], Chapt. XV.13.
References
| [a1] | P. Lévy, "Processus stochastiques et mouvement Brownien" , Gauthier-Villars (1965) |
| [a2] | N. Wax (ed.) , Selected papers on noise and stochastic processes , Dover, reprint (1954) |
| [a3] | E.B. Dynkin, "Markov processes" , 1 , Springer (1965) pp. Sect. 5.26 (Translated from Russian) |
| [a4] | W. Feller, "An introduction to probability theory and its applications", 1 , Wiley (1966) pp. Chapt. XV.13 |
| [a5] | I.I. [I.I. Gikhman] Gihman, A.V. [A.V. Skorokhod] Skorohod, "The theory of stochastic processes" , II , Springer (1975) (Translated from Russian) |
Einstein-Smoluchowski equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Einstein-Smoluchowski_equation&oldid=25526