Covariant derivative

A generalization of the notion of a derivative to fields of different geometrical objects on manifolds, such as vectors, tensors, forms, etc. It is a linear operator acting on the module of tensor fields of given valency and defined with respect to a vector field on a manifold and satisfying the following properties:

1) ,

2) , where and are differentiable functions on 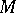. This mapping is trivially extended by linearity to the algebra of tensor fields and one additionally requires for the action on tensors of different valency:

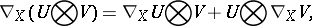where 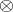denotes the tensor product. Thus 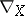is a derivation on the algebra of tensor fields (cf. Derivation in a ring); it has the additional properties of commuting with operations of contraction (cf. Contraction of a tensor), skew-symmetrization (cf. Alternation) and symmetrization of tensors (cf. Symmetrization (of tensors)).

Properties 1) and 2) of 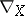(for vector fields) allow one to introduce on a linear connection (and the corresponding parallel displacement) and on the basis of this, to give a local definition of a covariant derivative which, when extended to the whole manifold, coincides with the operator defined above; see also Covariant differentiation.

Comments

There is not much of a difference between the notions of a covariant derivative and covariant differentiation and both are used in the same context.