Discrepancy
of a sequence 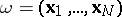 of points from the unit
of points from the unit  -dimensional cube
-dimensional cube 
The norm of the functional
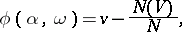 | (1) |
calculated in some metric. Here,  and
and 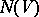 are, respectively, the volume of the domain
are, respectively, the volume of the domain 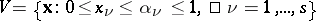 and the number of the points of
and the number of the points of 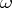 belonging to
belonging to 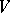 . If one considers the distribution of the points of
. If one considers the distribution of the points of 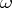 over domains of the type
over domains of the type 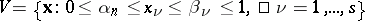 , then, in formula (1),
, then, in formula (1), 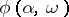 is usually replaced by
is usually replaced by 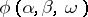 .
.
The following norms of the functional (1) are most often used:
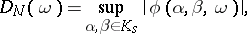 |
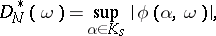 |
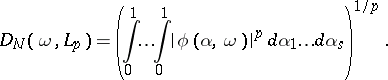 |
A sequence 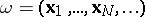 of points from the
of points from the  -dimensional unit cube
-dimensional unit cube 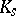 is uniformly distributed if and only if [1]
is uniformly distributed if and only if [1]
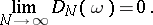 |
For any infinite sequence 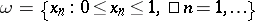 of one-dimensional points the following theorem [3] is valid:
of one-dimensional points the following theorem [3] is valid:
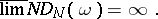 |
For any sequence 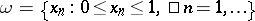 it is possible to find a sequence
it is possible to find a sequence 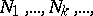 such that for
such that for  one has [4],
one has [4],
 |
The final result [5] for infinite sequences of one-dimensional points is that for 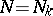 :
:
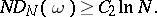 |
Studies were made of the discrepancies of various concrete sequences [6]–[8], and the estimates from above
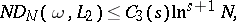 |
 |
were obtained, respectively, for finite and infinite sequences, as well as an estimate from below [4]: For any sequence of 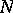 points, the following inequality is valid:
points, the following inequality is valid:
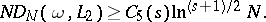 |
For any infinite sequence 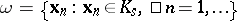 it is possible to find a sequence of numbers
it is possible to find a sequence of numbers 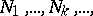 such that for
such that for 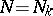 one has
one has
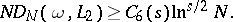 |
Also,
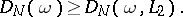 |
References
| [1] | H. Weyl, "Ueber die Gleichverteilung von Zahlen mod Eins" Math. Ann. , 77 (1916) pp. 313–352 |
| [2] | J.G. van der Corput, "Verteilungsfunktionen" Proc. Koninkl. Ned. Akad. Wet. A , 38 : 8 (1935) pp. 813–821; 1058–1066 |
| [3] | T. van Aardenne-Ehrenfest, "On the impossibility of a just distribution" Indag. Math. , 11 (1949) pp. 264–269 |
| [4] | K.F. Roth, "On irregularities of distribution" Mathematika , 1 (1954) pp. 73–79 |
| [5] | W.M. Schmidt, "Irregularities of distribution VII" Acta Arithm. , 21 (1972) pp. 45–50 |
| [6] | J.H. Halton, "On the efficiency of certain quasi-random sequences of points in evaluating multi-dimensional integrals" Numer. Math. , 2 : 2 (1960) pp. 84–90 |
| [7] | I.M. Sobol', "The distribution of points in a cube and the approximate evaluation of integrals" USSR Comp. Math. and Math. Phys. , 7 : 4 (1967) pp. 86–112 Zh. Vychisl. Mat. i Mat. Fiz. , 7 : 4 (1967) pp. 784–802 |
| [8] | N.M. Korobov, "Number-theoretical methods in approximate analysis" , Moscow (1963) (In Russian) |
| [9] | L. Kuipers, H. Niederreiter, "Uniform distribution of sequences" , Wiley (1974) |
Comments
See also Distribution modulo one; Distribution modulo one, higher-dimensional; Uniform distribution.
References
| [a1] | J. Beck, W.L. Chen, "Irregularities of distribution" , Cambridge Univ. Press (1987) |
Discrepancy. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Discrepancy&oldid=14906