Ordered sum
of partially ordered sets
An operation which associates with a system of disjoint partially ordered sets  , where the index set
, where the index set  is also partially ordered, a new partially ordered set
is also partially ordered, a new partially ordered set
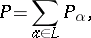 |
the elements of which are the elements of the set-theoretical union of the sets  , with order defined as follows. On the set
, with order defined as follows. On the set  one has
one has  if and only if either
if and only if either  and
and  in
in  or
or 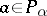 ,
,  and
and 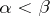 . Important particular cases of ordered sums are the cardinal and ordinal sums. The first of these is obtained when
. Important particular cases of ordered sums are the cardinal and ordinal sums. The first of these is obtained when 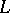 is trivially ordered, i.e. each of its elements is comparable only to itself, and the second when
is trivially ordered, i.e. each of its elements is comparable only to itself, and the second when 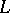 is a totally ordered set. Thus, in the cardinal sum of two disjoint partially ordered sets
is a totally ordered set. Thus, in the cardinal sum of two disjoint partially ordered sets  and
and 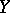 the relation
the relation 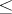 retains its meaning in the components
retains its meaning in the components 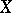 and
and 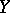 , while
, while 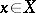 and
and  are incomparable; in the ordinal sum of
are incomparable; in the ordinal sum of 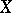 and
and 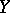 the order relation is again preserved in the components and
the order relation is again preserved in the components and 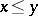 for all
for all 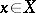 ,
, 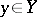 .
.
References
| [1] | G. Birkhoff, "Lattice theory" , Colloq. Publ. , 25 , Amer. Math. Soc. (1973) |
| [2] | L.A. Skornyakov, "Elements of lattice theory" , Hindushtan Publ. Comp. (1977) (Translated from Russian) |
Ordered sum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ordered_sum&oldid=14587