Kernel of a morphism in a category
A concept generalizing that of the kernel of a linear transformation of vector spaces, the kernel of a homomorphism of groups, rings, etc. Let 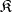 be a category with null (or zero) morphisms. A morphism
be a category with null (or zero) morphisms. A morphism 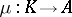 is called a kernel of a morphism
is called a kernel of a morphism 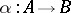 if
if 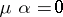 and if every morphism
and if every morphism  for which
for which 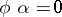 can be uniquely represented as
can be uniquely represented as 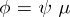 . A kernel of a morphism
. A kernel of a morphism 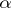 is denoted by
is denoted by 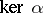 .
.
If 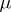 and
and 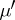 are both kernels of
are both kernels of 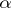 , then
, then 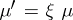 for a unique isomorphism
for a unique isomorphism 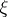 . Conversely, if
. Conversely, if 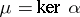 and if
and if 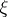 is an isomorphism, then
is an isomorphism, then  is a kernel of
is a kernel of 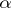 . Thus, the kernels of a morphism
. Thus, the kernels of a morphism 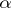 form a subobject of
form a subobject of 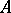 , denoted by
, denoted by 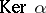 .
.
If 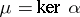 , then
, then 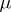 is a monomorphism. In general, the converse is not true; a monomorphism which occurs as a kernel is called a normal monomorphism. The kernel of the null morphism
is a monomorphism. In general, the converse is not true; a monomorphism which occurs as a kernel is called a normal monomorphism. The kernel of the null morphism  is the identity morphism
is the identity morphism 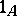 . The kernel of
. The kernel of 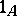 exists if and only if
exists if and only if 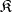 contains a null object (cf. Null object of a category).
contains a null object (cf. Null object of a category).
Kernels do not always exist in a category with null morphisms. On the other hand, in a category  with a null object a morphism
with a null object a morphism 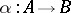 has a kernel if and only if a pullback of
has a kernel if and only if a pullback of 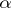 and
and  exists in
exists in  .
.
The concept of the "kernel of a morphism" is the dual to that of the "cokernel of a morphism" .
Comments
The concept "kernel of a pair of morphismskernel of a pair of morphisms" (not to be confused with "kernel pair of a morphism" ) is also frequently used. In English, the usual name for this concept is an equalizer. An equalizer of a parallel pair of morphism 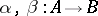 is a morphism
is a morphism 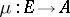 such that
such that 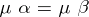 and such that every
and such that every 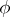 satisfying
satisfying  factors uniquely through
factors uniquely through 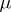 . Kernels are a special case of equalizers:
. Kernels are a special case of equalizers: 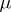 is a kernel of
is a kernel of 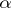 if and only if it is an equalizer of
if and only if it is an equalizer of  and
and  . Conversely, in an additive category an equalizer of
. Conversely, in an additive category an equalizer of 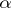 and
and  is the same thing as a kernel of
is the same thing as a kernel of  ; but in general the notion of equalizer is more widely applicable, since it does not require the existence of null morphisms. A monomorphism which occurs as an equalizer is called a regular monomorphism.
; but in general the notion of equalizer is more widely applicable, since it does not require the existence of null morphisms. A monomorphism which occurs as an equalizer is called a regular monomorphism.
References
| [a1] | B. Mitchell, "Theory of categories" , Acad. Press (1965) |
| [a2] | J. Adámek, "Theory of mathematical structures" , Reidel (1983) |
Kernel of a morphism in a category. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kernel_of_a_morphism_in_a_category&oldid=14382