Morphism
of a category
A term used to denote the elements of an arbitrary category which play the role of mappings of one set into another, homomorphisms of groups, rings, algebras, continuous mappings of topological spaces, etc. A morphism of a category is an undefined concept. Each category consists of elements of two classes, called the class of objects and the class of morphisms, respectively. The class of morphisms of a category  is usually denoted by
is usually denoted by  .
.
Any morphism  of a category
of a category  has a uniquely defined domain (source)
has a uniquely defined domain (source)  and a uniquely defined codomain (target)
and a uniquely defined codomain (target)  . All morphisms with common domain
. All morphisms with common domain  and codomain
and codomain  form a subset
form a subset  of
of  . The fact that
. The fact that 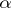 has domain
has domain  and codomain
and codomain  can be written in the usual way:
can be written in the usual way:  or, using arrows,
or, using arrows,  ,
,  , etc.
, etc.
The division of the elements of a category into morphisms and objects is meaningful only within the context of a fixed category, since the morphisms of one category may be the objects of another and conversely. The morphisms of any category form a system that is closed under a partial binary operation — multiplication. Depending on the properties of morphisms relative to this operation, special classes of morphisms can be distinguished, for example, the classes of monomorphisms, epimorphisms, bimorphisms, isomorphisms, null (zero) morphisms, normal monomorphisms, normal epimorphisms, etc. (cf. Monomorphism; Epimorphism; Bimorphism; Isomorphism; Normal monomorphism; Normal epimorphism).
Comments
References
| [a1] | B. Mitchell, "Theory of categories" , Acad. Press (1965) |
Morphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Morphism&oldid=13586