Algebraic operation

-ary operation, on a set

A mapping

of the -th Cartesian power of the set into the set itself. The number is known as the arity of the algebraic operation. Historically, the concepts of binary and unary (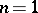) operations were the first to be considered. Nullary 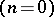operations are fixed elements of the set 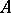; they are also known as distinguished elements or constants. In the 20th century the concept of an infinitary operation appeared, i.e. a mapping , where 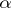is an arbitrary cardinal number. A set with a system of algebraic operations defined on it is called a universal algebra.

Comments

The study of infinitary operations actually started in the late 1950s [a1]. A nullary operation is also called a noughtary operation [a2].

References

[a1]	J. Stominski, "The theory of abstract algebras with infinitary operations" Rozprawy Mat. , 18 (1959)
[a2]	P.M. Cohn, "Universal algebra" , Reidel (1981) pp. 13–14