Dendritic manifold
A smooth odd-dimensional manifold of a special type which is the boundary of an even-dimensional manifold constructed from fibrations over spheres by a glueing scheme specified by some graph (tree).
Let 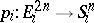 ,
, 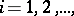 be a fibration over
be a fibration over  -spheres with as fibre the
-spheres with as fibre the  -ball
-ball 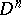 and as structure group the group
and as structure group the group 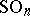 , and let
, and let 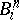 be the closed standard
be the closed standard  -ball in the
-ball in the  -sphere
-sphere 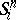 ; then
; then
 |
where 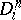 is the fibre
is the fibre 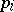 . Let
. Let
 |
be a homeomorphism realizing the glueing of two fibrations 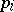 ,
, 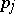 and mapping each
and mapping each  -ball
-ball 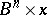 from
from 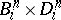 into some ball
into some ball 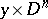 from
from 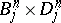 (the glueing alters the factors of the direct product
(the glueing alters the factors of the direct product  ). The result of glueing two fibrations
). The result of glueing two fibrations 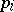 ,
, 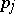 is the
is the  -dimensional manifold
-dimensional manifold 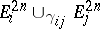 which, as a result of "angle smoothing" , is converted to a smooth manifold.
which, as a result of "angle smoothing" , is converted to a smooth manifold.
The fibrations 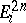 are considered as "structural blocks" from which it is possible to construct, by pairwise glueing, the resulting smooth manifold as follows. Let
are considered as "structural blocks" from which it is possible to construct, by pairwise glueing, the resulting smooth manifold as follows. Let 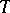 be a one-dimensional finite complex (a graph). Each vertex of
be a one-dimensional finite complex (a graph). Each vertex of  is brought into correspondence with a block
is brought into correspondence with a block 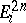 ; next,
; next, 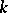 ,
, 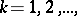 non-intersecting
non-intersecting  -balls
-balls 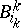 are selected in
are selected in 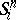 , where
, where 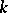 is equal to the branching index of the respective vertex, and the glueing is performed according to the scheme indicated by
is equal to the branching index of the respective vertex, and the glueing is performed according to the scheme indicated by  . The manifold with boundary thus obtained is denoted by
. The manifold with boundary thus obtained is denoted by 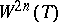 (neglecting the dependence on the choice of
(neglecting the dependence on the choice of 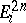 ). If
). If 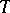 is a tree, and therefore the graph is without cycles, the boundary
is a tree, and therefore the graph is without cycles, the boundary 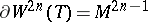 is said to be a dendritic manifold.
is said to be a dendritic manifold.
If 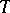 is a tree,
is a tree, 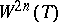 has the homotopy type of a bouquet of
has the homotopy type of a bouquet of 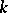 spheres, where
spheres, where 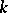 is the number of vertices of
is the number of vertices of 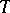 .
.
The dendritic manifold 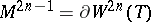 is an integral homology
is an integral homology 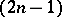 -sphere if and only if the determinant of the matrix of the integral bilinear intersection
-sphere if and only if the determinant of the matrix of the integral bilinear intersection 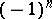 -form defined on the lattice of
-form defined on the lattice of  -dimensional homology groups
-dimensional homology groups 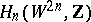 equals
equals 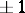 . If this condition is met, the manifold
. If this condition is met, the manifold  is called a plumbing.
is called a plumbing.
If 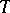 is a tree and
is a tree and 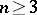 ,
, 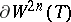 is simply connected; if
is simply connected; if 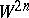 is a plumbing, the boundary
is a plumbing, the boundary 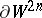 is a homotopy sphere if
is a homotopy sphere if 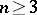 .
.
If the plumbing 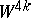 is parallelizable, the diagonal of the intersection matrix of
is parallelizable, the diagonal of the intersection matrix of  -dimensional cycles is occupied by even numbers; in such a case the signature of the intersection matrix is divisible by 8. The plumbing
-dimensional cycles is occupied by even numbers; in such a case the signature of the intersection matrix is divisible by 8. The plumbing 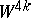 is parallelizable if and only if all the fibrations over
is parallelizable if and only if all the fibrations over 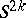 used in constructing
used in constructing 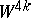 are stably trivial; e.g., if all fibrations used in constructing
are stably trivial; e.g., if all fibrations used in constructing 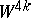 are tangent bundles on discs over
are tangent bundles on discs over 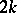 -dimensional spheres, the plumbing
-dimensional spheres, the plumbing 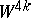 is parallelizable. The plumbing
is parallelizable. The plumbing 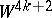 will be parallelizable if and only if any fibration
will be parallelizable if and only if any fibration 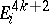 used as a block in the construction of
used as a block in the construction of 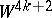 is either trivial or is a tubular neighbourhood of the diagonal in the product
is either trivial or is a tubular neighbourhood of the diagonal in the product  , i.e. is a tangent bundle on discs over
, i.e. is a tangent bundle on discs over 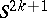 . If the plumbing
. If the plumbing 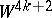 is parallelizable, its intersection matrix can be reduced to the symplectic form consisting of blocks
is parallelizable, its intersection matrix can be reduced to the symplectic form consisting of blocks
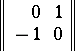 |
situated along the main diagonal.
Especially important plumbings are the Milnor manifolds of dimension 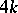 ,
, 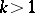 , and the Kervaire manifolds of dimension
, and the Kervaire manifolds of dimension 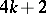 ,
, 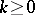 . The Milnor manifolds are constructed as follows: A few copies of the tubular neighbourhood
. The Milnor manifolds are constructed as follows: A few copies of the tubular neighbourhood 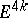 of the diagonal in the product
of the diagonal in the product 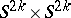 are taken as blocks, while the graph
are taken as blocks, while the graph 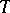 is of the form
is of the form
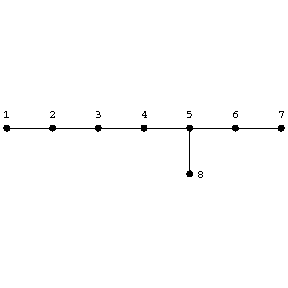
Figure: d031010a
Under these conditions the manifold 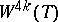 realizes a quadratic form of order eight, in which every element on the main diagonal equals 2, while the signature equals 8.
realizes a quadratic form of order eight, in which every element on the main diagonal equals 2, while the signature equals 8.
In constructing the Kervaire manifolds 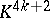 one takes two copies of the block obtained as the tubular neighbourhood
one takes two copies of the block obtained as the tubular neighbourhood 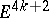 of the diagonal in the product
of the diagonal in the product 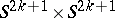 . They are glued together so that the intersection matrix has the form
. They are glued together so that the intersection matrix has the form
 |
The boundary of a Milnor manifold 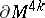 (a Milnor sphere) is never diffeomorphic to the standard sphere
(a Milnor sphere) is never diffeomorphic to the standard sphere 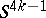 . As regards Kervaire manifolds, this problem has not yet (1987) been conclusively solved. If
. As regards Kervaire manifolds, this problem has not yet (1987) been conclusively solved. If  , then the boundary of a Kervaire manifold
, then the boundary of a Kervaire manifold 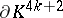 (a Kervaire sphere) is always non-standard; if
(a Kervaire sphere) is always non-standard; if  , one obtains the standard sphere
, one obtains the standard sphere 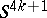 for
for 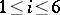 , while for other
, while for other 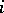 it remains unsolved (cf. Kervaire invariant).
it remains unsolved (cf. Kervaire invariant).
The Kervaire manifolds 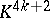 of dimension 2, 6 or 14 are products of spheres
of dimension 2, 6 or 14 are products of spheres 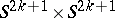 ,
, 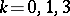 respectively, after an open cell has been discarded, while all other Kervaire manifolds are not homeomorphic to the products of spheres with a discarded cell.
respectively, after an open cell has been discarded, while all other Kervaire manifolds are not homeomorphic to the products of spheres with a discarded cell.
The PL-manifolds  and
and 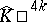 are often used in the topology of manifolds. These manifolds are obtained by adding a cone over the boundary of, respectively, the Milnor manifolds
are often used in the topology of manifolds. These manifolds are obtained by adding a cone over the boundary of, respectively, the Milnor manifolds  and the Kervaire manifolds
and the Kervaire manifolds 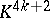 . In the theory of four-dimensional manifolds a certain simply-connected almost-parallelizable manifold
. In the theory of four-dimensional manifolds a certain simply-connected almost-parallelizable manifold 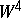 (usually called a Rokhlin manifold) plays an especially important role; its signature is 16, cf. [6]. In the known examples of Rokhlin manifolds, the minimum value of the two-dimensional Betti number is 22. The second manifold is
(usually called a Rokhlin manifold) plays an especially important role; its signature is 16, cf. [6]. In the known examples of Rokhlin manifolds, the minimum value of the two-dimensional Betti number is 22. The second manifold is 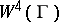 , where
, where 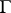 is the graph indicated above, and the tubular neighbourhood of the diagonal in the product
is the graph indicated above, and the tubular neighbourhood of the diagonal in the product 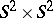 is taken as the block. The boundary of the manifold
is taken as the block. The boundary of the manifold 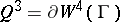 thus obtained is a dodecahedral space which is not simply connected.
thus obtained is a dodecahedral space which is not simply connected.
The three-dimensional dendritic manifolds 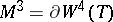 belong to the class of so-called Seifert manifolds. Not all three-dimensional manifolds are dendritic manifolds; the Poincaré conjecture holds for dendritic manifolds. In particular, three-dimensional lens spaces (cf. Lens space) are obtained by glueing two blocks only.
belong to the class of so-called Seifert manifolds. Not all three-dimensional manifolds are dendritic manifolds; the Poincaré conjecture holds for dendritic manifolds. In particular, three-dimensional lens spaces (cf. Lens space) are obtained by glueing two blocks only.
References
| [1] | M. Kervaire, "A manifold which does not admit any differentiable structure" Comment. Math. Helv. , 34 (1960) pp. 257–270 |
| [2] | M. Kervaire, J. Milnor, "Groups of homotopy spheres. I" Ann. of Math. , 77 : 3 (1963) pp. 504–537 |
| [3] | J.W. Milnor, "Differential topology" , Lectures on modern mathematics , II , Wiley (1964) pp. 165–183 |
| [4] | F. Hirzebruch, W.D. Neumann, S.S. Koh, "Differentiable manifolds and quadratic forms" , M. Dekker (1971) |
| [5] | W.B. Browder, "Surgery on simply-connected manifolds" , Springer (1972) |
| [6] | R. Mandelbaum, "Four-dimensional topology: an introduction" Bull. Amer. Math. Soc. , 2 : 1 (1980) pp. 1–159 |
Comments
The technique described in the article above and leading to so-called "dendritic manifolds" (a phrase not often used in the West) is known as surgery, plumbing or as the technique of spherical modification.
Dendritic manifold. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dendritic_manifold&oldid=12613