Ellipse
(real)
A planar curve obtained by the intersection of a circular cone with a plane not passing through the vertex of the cone and intersecting all its generators in points of one of its sheets. An ellipse also turns out to be the set of points 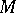 of a plane (see Fig.) for each of which the sum of the distances from two given points
of a plane (see Fig.) for each of which the sum of the distances from two given points  and
and 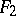 (the foci) is constant and equal to
(the foci) is constant and equal to 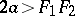 . The distance between the foci is called the focal distance and is usually denoted by
. The distance between the foci is called the focal distance and is usually denoted by 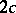 . The mid-point of the segment
. The mid-point of the segment  is called the centre of the ellipse.
is called the centre of the ellipse.
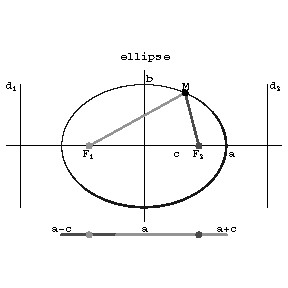
Figure: e035390a
The line on which the foci of the ellipse lie is called the first (or focal) axis. The line through the centre of the ellipse and perpendicular to the first axis is called the second axis. The axes of the ellipse are its axes of symmetry. The points of intersection of the ellipse with the axes of symmetry are called its vertices. The major axis of the ellipse is the segment (and also its length 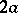 ) of the first axis between the vertices. The minor axis is the segment (and also its length
) of the first axis between the vertices. The minor axis is the segment (and also its length 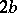 ) of the second axis between the vertices. The number
) of the second axis between the vertices. The number 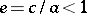 is called the eccentricity of the ellipse. A diameter of the ellipse is any straight line passing through the centre; a diameter can also be defined as a straight line passing through the mid-points of parallel chords. The directrix of the ellipse corresponding to a given focus
is called the eccentricity of the ellipse. A diameter of the ellipse is any straight line passing through the centre; a diameter can also be defined as a straight line passing through the mid-points of parallel chords. The directrix of the ellipse corresponding to a given focus 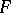 (the associated directrix) is the line
(the associated directrix) is the line  perpendicular to the first axis at distance
perpendicular to the first axis at distance 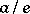 from the centre. An ellipse has in general two directrices. An ellipse is a central second-order curve with canonical equation
from the centre. An ellipse has in general two directrices. An ellipse is a central second-order curve with canonical equation
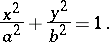 |
The equation of the tangent to an ellipse at a point 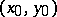 is
is
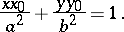 |
The focal parameter 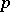 of an ellipse (half the length of the chord passing through a focus and perpendicular to the first axis) is
of an ellipse (half the length of the chord passing through a focus and perpendicular to the first axis) is 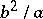 . By means of the focal parameter one can write the equation of an ellipse in the form
. By means of the focal parameter one can write the equation of an ellipse in the form
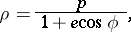 |
where 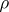 and
and  are polar coordinates,
are polar coordinates, 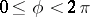 .
.
If 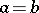 , the ellipse becomes a circle,
, the ellipse becomes a circle,  is its centre,
is its centre,  its radius,
its radius, 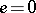 , and there is no directrix.
, and there is no directrix.
An ellipse has the following optical property: A light ray emanating from one focus passes through the other focus after mirror reflection in the ellipse.
A second-order curve with canonical equation
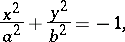 |
where  and
and 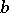 are real numbers, is called an imaginary ellipse.
are real numbers, is called an imaginary ellipse.
Comments
As the name suggests, an imaginary ellipse does not have real points.
Other characterizations of an ellipse are as follows.
An ellipse is the affine image of a circle; an ellipse is a non-degenerate conic (i.e. a second-order curve) which does not meet the line at infinity; an ellipse is the set of points whose distances to a given point (the focus) and to a given line (the associated directrix) are in constant ratio; and an ellipse is a planar compact non-singular algebraic curve of the second degree.
For a systematic exposition of the properties of ellipses see [a1], pp. 245-247 and Chapt. 17, and [a2].
References
| [a1] | M. Berger, "Geometry" , II , Springer (1987) |
| [a2] | J. Coolidge, "A history of the conic sections and quadric surfaces" , Dover, reprint (1968) |
Ellipse. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ellipse&oldid=11394