Torsion
The torsion of a curve 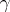 in
in  -space is a quantity characterizing the deviation of
-space is a quantity characterizing the deviation of 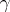 from its osculating plane. Let
from its osculating plane. Let 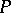 be an arbitrary point on
be an arbitrary point on 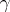 and let
and let 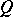 be a point near
be a point near  , let
, let 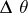 be the angle between the osculating planes to
be the angle between the osculating planes to 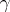 at
at 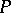 and
and 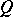 , and let
, and let 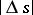 be the length of the arc
be the length of the arc 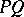 of
of  . The absolute torsion
. The absolute torsion 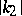 of
of  at
at  is defined as
is defined as
 |
The torsion of 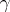 is defined as
is defined as 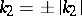 , it being considered positive (negative) if an observer at
, it being considered positive (negative) if an observer at 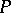 sees the osculating plane turning in the counter-clockwise (clockwise) sense as the point moves along the curve in the direction of increasing
sees the osculating plane turning in the counter-clockwise (clockwise) sense as the point moves along the curve in the direction of increasing  from the binormal vector to the principal normal vector.
from the binormal vector to the principal normal vector.
For a regular (thrice continuously differentiable) curve the torsion is defined at any point where its curvature does not vanish. If 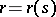 is the natural parametrization of the curve, then
is the natural parametrization of the curve, then
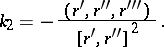 |
The torsion is sometimes called the second curvature.
The torsion and the curvature, as functions of the arc length, determine the curve up to its position in space.
A curve with vanishing torsion at each point is a planar curve.
Comments
The term "second curvature" is commonly used in higher-dimensional Frénet theory, where the curve is considered in Euclidean  -space. If the curve is sufficiently differentiable, then in this case, generically,
-space. If the curve is sufficiently differentiable, then in this case, generically, 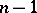 curvatures can be defined for it, and the last curvature can be equipped with a sign again.
curvatures can be defined for it, and the last curvature can be equipped with a sign again.
The torsion of a curve in  -space is connected with the angle of rotation of a parallel normal vector field along the curve. For a closed curve with positive curvature the angle of rotation of a parallel normal vector field along one period of the curve is given by its total torsion. This is also called the total twist of the curve.
-space is connected with the angle of rotation of a parallel normal vector field along the curve. For a closed curve with positive curvature the angle of rotation of a parallel normal vector field along one period of the curve is given by its total torsion. This is also called the total twist of the curve.
The geodesic torsion is a generalization of the torsion of a curve; it is an invariant of a strip in the space  and is defined by
and is defined by
 |
where 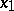 is the tangent vector to the base curve
is the tangent vector to the base curve 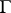 of the strip and
of the strip and 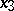 is the normal vector of the strip. The ordinary torsion
is the normal vector of the strip. The ordinary torsion  of a curve
of a curve 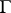 with non-vanishing curvature is expressed in terms of
with non-vanishing curvature is expressed in terms of  and the normal and geodesic curvatures
and the normal and geodesic curvatures  and
and  by the following formula:
by the following formula:
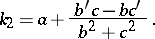 |
The vanishing of the geodesic torsion is a characteristic property of strips of curvature, in particular for strips belonging to a surface in  — see Curvature line.
— see Curvature line.
Analogous concepts can be defined for strips in a Riemannian space (see , ).
The torsion of a submanifold is a generalization of the torsion of a curve, namely the curvature of the connection (cf. Connection; Connections on a manifold) induced in the normal bundle 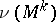 of a manifold
of a manifold  immersed in a Riemannian space
immersed in a Riemannian space  . Let
. Let 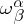 be the connection form in
be the connection form in 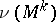 , let
, let 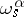 be the Eulerian curvature forms of
be the Eulerian curvature forms of 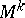 in
in  ,
, 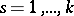 ;
; 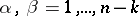 . Then the forms
. Then the forms
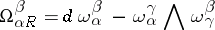 |
define the Riemannian torsion, and the forms
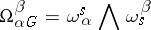 |
the Gaussian torsion of 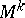 in
in 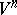 . These torsions are related by the formula
. These torsions are related by the formula
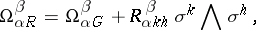 |
where 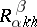 are the components of the curvature tensor of
are the components of the curvature tensor of 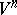 in the direction of a bivector tangent to
in the direction of a bivector tangent to 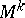 and
and  is an orthogonal cobasis of the tangent space to
is an orthogonal cobasis of the tangent space to 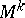 . The tensors
. The tensors 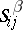 obtained by decomposing the torsion forms
obtained by decomposing the torsion forms 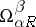 (
(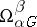 ) in terms of the forms
) in terms of the forms 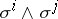 are known as the Gaussian and Riemannian torsions.
are known as the Gaussian and Riemannian torsions.
Example. Let 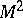 be a surface in the Euclidean space
be a surface in the Euclidean space 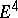 . Then the Gaussian and Riemannian torsions are equal and reduce to the single number
. Then the Gaussian and Riemannian torsions are equal and reduce to the single number
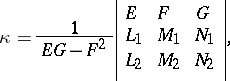 |
where 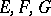 are the coefficients of the first fundamental form of
are the coefficients of the first fundamental form of 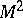 in
in 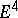 and
and 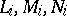 are the coefficients of the second fundamental form of
are the coefficients of the second fundamental form of 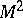 in
in 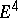 . The vanishing of
. The vanishing of 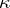 in some neighbourhood may be interpreted geometrically as the degeneration of the curvature ellipsoid to an interval on a straight line; there then exist two families of orthogonal curvature lines, the tangents to which correspond to the end-points of this interval. The equality
in some neighbourhood may be interpreted geometrically as the degeneration of the curvature ellipsoid to an interval on a straight line; there then exist two families of orthogonal curvature lines, the tangents to which correspond to the end-points of this interval. The equality 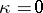 is locally a necessary and sufficient condition for
is locally a necessary and sufficient condition for 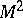 to lie in a Riemannian space
to lie in a Riemannian space  immersed in
immersed in 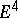 , and for the normal to
, and for the normal to 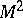 in the tangent space to
in the tangent space to 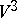 to point in the direction of a principal vector of the Ricci tensor of
to point in the direction of a principal vector of the Ricci tensor of 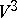 . In particular, vanishing torsion is a necessary condition for
. In particular, vanishing torsion is a necessary condition for 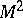 to be flat in
to be flat in 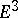 .
.
The torsion of an affine connection 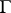 is a quantity characterizing the degree to which the covariant derivatives (cf. Covariant derivative) of some function on a manifold
is a quantity characterizing the degree to which the covariant derivatives (cf. Covariant derivative) of some function on a manifold 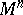 with this connection
with this connection 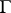 deviate from commutativity. It is defined by the transformation
deviate from commutativity. It is defined by the transformation
 |
where 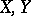 are vector fields on
are vector fields on 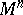 ,
,  is the covariant derivative of
is the covariant derivative of 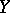 along
along  , and
, and 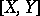 is the Lie bracket of
is the Lie bracket of 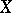 and
and 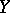 . Setting
. Setting 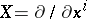 and
and 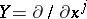 in local coordinates
in local coordinates 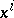 ,
, 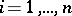 , the transformation
, the transformation 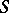 is given by
is given by
 |
the tensor 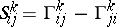 , where
, where 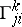 are the components of
are the components of 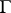 relative to the chosen basis, is known as the torsion tensor.
relative to the chosen basis, is known as the torsion tensor.
An equivalent definition of the torsion utilizes the covariant differential vector-valued  -form
-form  of the displacement of the connection,
of the displacement of the connection,
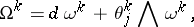 |
which is called the torsion form; here 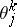 are the connection forms for
are the connection forms for 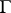 . In terms of the local cobasis
. In terms of the local cobasis 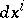 (the dual of the basis
(the dual of the basis 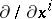 ), the form is:
), the form is:
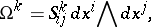 |
where 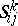 has the same meaning as before.
has the same meaning as before.
The torsion of an affine connection  admits the following geometrical interpretation. The evolvent of every infinitesimal contour
admits the following geometrical interpretation. The evolvent of every infinitesimal contour 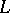 issuing from a point
issuing from a point 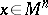 and returning to that point on the tangent space to
and returning to that point on the tangent space to  at
at  is no longer a closed curve. The vector difference between the end-points of the evolvent, evaluated up to second-order terms, has the components
is no longer a closed curve. The vector difference between the end-points of the evolvent, evaluated up to second-order terms, has the components 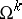 ,
, 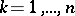 . In other words, this vector is proportional to the bounded contour
. In other words, this vector is proportional to the bounded contour 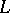 of the two-dimensional area element with bivector
of the two-dimensional area element with bivector 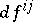 :
:  . These ideas form the basis for the interpretation of an elastic medium with continuously distributed sources of internal stress in the form of displacements; the vector
. These ideas form the basis for the interpretation of an elastic medium with continuously distributed sources of internal stress in the form of displacements; the vector 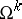 is then an analogue of the Burgers vector (see –).
is then an analogue of the Burgers vector (see –).
Example. In a two-dimensional Riemannian space 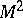 with a metric connection, the torsion tensor reduces to a vector:
with a metric connection, the torsion tensor reduces to a vector: 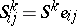 , where
, where 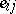 is the metric bivector. Consider a small triangle in
is the metric bivector. Consider a small triangle in 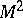 , the sides of which are geodesics of lengths
, the sides of which are geodesics of lengths 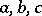 , with angles
, with angles 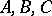 . The principal part of the projection of the vector
. The principal part of the projection of the vector 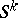 at the point
at the point 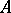 on the side
on the side  is equal to
is equal to  divided by the area
divided by the area  of the triangle, while that of the projection of the same vector on the perpendicular to
of the triangle, while that of the projection of the same vector on the perpendicular to 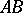 is
is  divided by
divided by 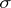 . Thus, if
. Thus, if 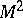 has zero torsion, the cosine and sine theorems of conventional trigonometry are valid up to quantities which are small in comparison with
has zero torsion, the cosine and sine theorems of conventional trigonometry are valid up to quantities which are small in comparison with 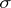 .
.
The torsion of a space 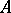 is an element
is an element  of the Whitehead group
of the Whitehead group 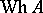 defined by the pair
defined by the pair  , where
, where 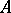 is a finite cellular space and the imbedding
is a finite cellular space and the imbedding  is a homotopy equivalence. Equivalently: The torsion is an element of the Whitehead group
is a homotopy equivalence. Equivalently: The torsion is an element of the Whitehead group  of the fundamental group
of the fundamental group 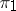 . The torsion is invariant under cellular expansions and contractions and under cellular refinements. It has been proved that the torsion is a topological invariant. If
. The torsion is invariant under cellular expansions and contractions and under cellular refinements. It has been proved that the torsion is a topological invariant. If  is simply connected, its torsion is zero (cf. Whitehead torsion).
is simply connected, its torsion is zero (cf. Whitehead torsion).
If 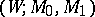 is an arbitrary
is an arbitrary 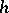 -cobordism, then
-cobordism, then  , where
, where  is the cellular space associated with a given handle decomposition of the manifold
is the cellular space associated with a given handle decomposition of the manifold 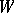 (of the manifold
(of the manifold 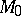 ), is called the torsion of the
), is called the torsion of the 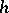 -cobordism.
-cobordism.
Let 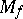 be the cylinder of a cellular mapping
be the cylinder of a cellular mapping 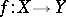 which is a homotopy equivalence (cf. Mapping cylinder). Then
which is a homotopy equivalence (cf. Mapping cylinder). Then 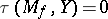 , but
, but 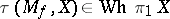 does not vanish everywhere. It is defined by the formula
does not vanish everywhere. It is defined by the formula
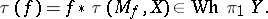 |
This element is called the torsion of the mapping 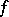 (sometimes
(sometimes 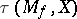 itself is called the torsion). If
itself is called the torsion). If  , the mapping is called a simple homotopy equivalence (see ).
, the mapping is called a simple homotopy equivalence (see ).
The torsion of a finitely-generated Abelian group 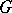 is the group
is the group 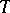 of all elements of finite order
of all elements of finite order  in
in 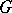 . The numbers
. The numbers 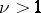 may be chosen uniquely, up to permutations, as powers of prime numbers, and they are then called the torsion coefficients of
may be chosen uniquely, up to permutations, as powers of prime numbers, and they are then called the torsion coefficients of 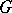 (see [9]).
(see [9]).
References
| [1] | E. Cartan, "Leçons sur la géométrie des espaces de Riemann" , Gauthier-Villars (1946) |
| [2] | W. Blaschke, "Vorlesungen über Differentialgeometrie und geometrische Grundlagen von Einsteins Relativitätstheorie. Elementare Differentialgeometrie" , 1 , Springer (1921) |
| [3] | Itogi Nauk. Algebra. Topol. Geom. 1969 (1971) pp. 123–168 |
| [4] | R. Sulanke, P. Wintgen, "Differentialgeometrie und Faserbündel" , Birkhäuser (1972) |
| [5] | A.P. Norden, "Spaces with an affine connection" , Nauka , Moscow-Leningrad (1976) (In Russian) |
| [6a] | E. Cartan, "Sur les variétés à connexion affine et la théorie de la rélativité généralisée" Ann. Sci. Ecole Norm. Sup. (3) , 40 (1923) pp. 325–412 |
| [6b] | E. Cartan, "Sur les variétés à connexion affine et la théorie de la rélativité généralisée" Ann. Sci. Ecole Norm. Sup. (3) , 41 (1924) pp. 1–25 |
| [6c] | E. Cartan, "Sur les variétés à connexion affine et la théorie de la rélativité généralisée" Ann. Sci. Ecole Norm. Sup. (3) , 42 (1925) pp. 17–88 |
| [6d] | E. Cartan, "Sur les variétés à connexion projective" Bull. Soc. Math. France , 52 (1924) pp. 205–241 |
| [6e] | E. Cartan, "Sur les variétés à connexion conforme" Ann. Soc. Polon. Math. , 2 (1924) pp. 171–221 |
| [7] | J.A. Schouten, "Tensor analysis for physicists" , Cambridge Univ. Press (1951) |
| [8] | C.P. Rourke, B.J. Sanderson, "Introduction to piecewise-linear topology" , Springer (1972) |
| [9] | A.G. Kurosh, "The theory of groups" , 1–2 , Chelsea (1955–1956) (Translated from Russian) |
M.I. Voitsekhovskii
Comments
The torsion subgroup 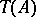 of an Abelian group,
of an Abelian group, 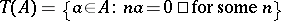 , defines a functor of the category of Abelian groups into itself. For torsion in the case of a left module over an associative ring cf. Torsion submodule.
, defines a functor of the category of Abelian groups into itself. For torsion in the case of a left module over an associative ring cf. Torsion submodule.
References
| [a1] | M. Berger, B. Gostiaux, "Differential geometry: manifolds, curves, and surfaces" , Springer (1988) (Translated from French) |
| [a2] | H.S.M. Coxeter, "Introduction to geometry" , Wiley (1961) |
| [a3] | M.P. Do Carmo, "Differential geometry of curves and surfaces" , Prentice-Hall (1976) pp. 145 |
| [a4] | G. Darboux, "Leçons sur la théorie générale des surfaces et ses applications géométriques du calcul infinitésimal" , 1–4 , Chelsea, reprint (1972) |
| [a5] | H.W. Guggenheimer, "Differential geometry" , McGraw-Hill (1963) pp. 25; 60 |
| [a6] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1–2 , Interscience (1963–1969) |
| [a7] | B. O'Neill, "Elementary differential geometry" , Acad. Press (1966) |
| [a8] | M. Spivak, "A comprehensive introduction to differential geometry" , 1979 , Publish or Perish pp. 1–5 |
| [a9] | W. Klingenberg, "Riemannian geometry" , de Gruyter (1982) (Translated from German) |
| [a10] | E. Cartan, "Oeuvres complètes" , Gauthier-Villars (1952) |
Torsion. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Torsion&oldid=11314