Schoenflies conjecture
From Encyclopedia of Mathematics
The conjecture stating that the common boundary of two domains in the plane is always decomposable. A space 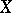 is called decomposable if it is connected and can be represented as the union of two closed connected subsets different from
is called decomposable if it is connected and can be represented as the union of two closed connected subsets different from 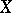 . This conjecture was stated by A. Schoenflies in 1908 and disproved by L.E.J. Brouwer in 1910, who discovered indecomposable continua, i.e. connected compact sets that cannot be represented as the union of two closed proper connected subsets. For each finite or countable
. This conjecture was stated by A. Schoenflies in 1908 and disproved by L.E.J. Brouwer in 1910, who discovered indecomposable continua, i.e. connected compact sets that cannot be represented as the union of two closed proper connected subsets. For each finite or countable 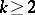 ,
, 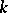 domains in the plane have been constructed with an indecomposable continuum as common boundary.
domains in the plane have been constructed with an indecomposable continuum as common boundary.
References
| [1] | K. Kuratowski, "Topology" , 2 , Acad. Press (1968) (Translated from French) |
How to Cite This Entry:
Schoenflies conjecture. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Schoenflies_conjecture&oldid=11230
Schoenflies conjecture. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Schoenflies_conjecture&oldid=11230
This article was adapted from an original article by B.A. Efimov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article