Simplicial complex
simplicial scheme, abstract simplicial complex
A set, whose elements are called vertices, in which a family of finite non-empty subsets, called simplexes or simplices, is distinguished, such that every non-empty subset of a simplex  is a simplex, called a face of
is a simplex, called a face of  , and every one-element subset is a simplex.
, and every one-element subset is a simplex.
A simplex is called  -dimensional if it consists of
-dimensional if it consists of 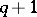 vertices. The maximal dimension of its simplices (which may be infinite) is called the dimension
vertices. The maximal dimension of its simplices (which may be infinite) is called the dimension 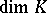 of a simplicial complex
of a simplicial complex 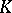 . A simplicial complex is called locally finite if each of its vertices belongs to only finitely many simplices. A simplicial complex is called ordered if its vertices admit a partial ordering that is linear on every simplex.
. A simplicial complex is called locally finite if each of its vertices belongs to only finitely many simplices. A simplicial complex is called ordered if its vertices admit a partial ordering that is linear on every simplex.
Example. Let 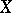 be a set and let
be a set and let 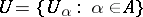 be a family of non-empty subsets of
be a family of non-empty subsets of 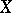 . A non-empty finite subset
. A non-empty finite subset 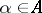 is called a simplex if the set
is called a simplex if the set 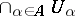 is non-empty. The resulting simplicial complex
is non-empty. The resulting simplicial complex 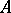 is called the nerve of the family
is called the nerve of the family  (cf. Nerve of a family of sets).
(cf. Nerve of a family of sets).
A simplicial mapping of a simplicial complex 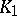 into a simplicial complex
into a simplicial complex 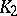 is a mapping
is a mapping  such that for every simplex
such that for every simplex  in
in 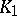 , its image
, its image  is a simplex in
is a simplex in 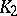 . Simplicial complexes and their simplicial mappings form a category.
. Simplicial complexes and their simplicial mappings form a category.
If a simplicial mapping 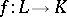 is an inclusion, then
is an inclusion, then 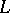 is called a simplicial subcomplex of
is called a simplicial subcomplex of  . All simplices of a simplicial complex
. All simplices of a simplicial complex 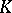 of dimension at most
of dimension at most  form a simplicial subcomplex of
form a simplicial subcomplex of 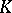 , which is written
, which is written  and is called the
and is called the  -dimensional (or
-dimensional (or  -) skeleton of
-) skeleton of  . A simplicial subcomplex
. A simplicial subcomplex 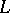 of a simplicial complex
of a simplicial complex 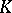 is called full if every simplex in
is called full if every simplex in 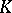 whose vertices all belong to
whose vertices all belong to  is itself in
is itself in 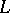 .
.
Every simplicial complex 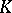 canonically determines a simplicial set
canonically determines a simplicial set 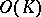 , whose simplices of dimension
, whose simplices of dimension  are all
are all 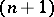 -tuples
-tuples 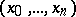 of vertices of
of vertices of 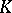 with the property that there is a simplex
with the property that there is a simplex  in
in 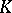 such that
such that  for each
for each 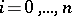 . The boundary operators
. The boundary operators  and the degeneracy operators
and the degeneracy operators 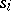 of
of  are given by the formulas
are given by the formulas
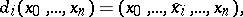 |
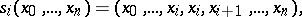 |
where  denotes the omission of the symbol beneath it. When
denotes the omission of the symbol beneath it. When 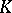 is ordered one can define a simplicial subset
is ordered one can define a simplicial subset 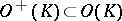 , consisting of those simplices
, consisting of those simplices 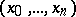 for which
for which 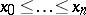 . The (co)homology groups of
. The (co)homology groups of 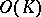 are isomorphic to the (co)homology groups of
are isomorphic to the (co)homology groups of 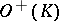 and called the (co)homology groups of
and called the (co)homology groups of 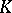 .
.
To every triangulation (simplicial space) 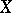 corresponds a simplicial complex, whose vertices are the vertices of
corresponds a simplicial complex, whose vertices are the vertices of 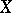 and whose simplices are those non-empty finite sets of vertices which span a simplex in
and whose simplices are those non-empty finite sets of vertices which span a simplex in  . For every simplicial complex
. For every simplicial complex  there is a triangulation, unique up to an isomorphism, whose simplicial complex is
there is a triangulation, unique up to an isomorphism, whose simplicial complex is  . It is called the geometric realization (or body, or geometric simplicial complex) of
. It is called the geometric realization (or body, or geometric simplicial complex) of 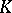 , and is denoted by
, and is denoted by 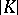 . This yields the geometric model in the sense of Giever–Hu (see Simplicial set)
. This yields the geometric model in the sense of Giever–Hu (see Simplicial set) 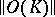 of the simplicial set
of the simplicial set 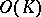 , and when
, and when 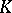 is ordered, the geometric model in the sense of Milnor
is ordered, the geometric model in the sense of Milnor 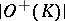 of the simplicial set
of the simplicial set  . The correspondence
. The correspondence  is a covariant functor from the category of simplicial complexes to the category of cellular spaces. A topological space
is a covariant functor from the category of simplicial complexes to the category of cellular spaces. A topological space 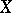 homeomorphic to the body
homeomorphic to the body 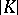 of some simplicial complex
of some simplicial complex 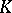 is called a polyhedron (or a triangulated space, cf. Polyhedron, abstract) and the pair
is called a polyhedron (or a triangulated space, cf. Polyhedron, abstract) and the pair 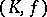 , where
, where 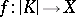 is the homeomorphism, is called a triangulation of
is the homeomorphism, is called a triangulation of 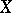 .
.
The points of the topological space 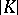 can be identified with the functions
can be identified with the functions 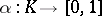 for which the set
for which the set 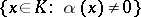 is a simplex in
is a simplex in 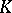 and
and
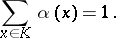 |
The number 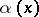 is called the
is called the  -th barycentric coordinate of
-th barycentric coordinate of 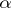 . The formula
. The formula
 |
defines a metric on 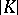 , but the corresponding metric topology is, in general, stronger than the original one. The set
, but the corresponding metric topology is, in general, stronger than the original one. The set 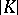 equipped with this metric topology is written as
equipped with this metric topology is written as 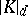 .
.
A simplicial complex  is isomorphic to the nerve of the family of stars of vertices of the space
is isomorphic to the nerve of the family of stars of vertices of the space  , that is, to the nerve of the family of open subsets
, that is, to the nerve of the family of open subsets  , where
, where  .
.
The following statements are equivalent: 1) the simplicial complex 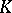 is locally finite; 2) the space
is locally finite; 2) the space 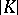 is locally compact; 3)
is locally compact; 3) 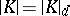 ; 4)
; 4)  is metrizable; and 5)
is metrizable; and 5) 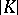 satisfies the first axiom of countability. Moreover, the space
satisfies the first axiom of countability. Moreover, the space 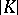 is separable (compact) if and only if
is separable (compact) if and only if 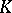 is at most countable (finite).
is at most countable (finite).
The cells of the complex 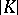 are in one-to-one correspondence with the simplices of
are in one-to-one correspondence with the simplices of  , and the closure
, and the closure 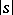 of the cell corresponding to a simplex
of the cell corresponding to a simplex  is given by
is given by
 |
It is homeomorphic to the 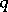 -dimensional closed ball, where
-dimensional closed ball, where 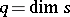 , so that the complex
, so that the complex 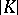 is regular. In addition, each set
is regular. In addition, each set 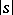 has a canonical linear (affine) structure, with respect to which it is isomorphic to the standard simplex
has a canonical linear (affine) structure, with respect to which it is isomorphic to the standard simplex 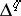 . Because of this, and the fact that
. Because of this, and the fact that 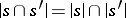 for all simplices
for all simplices 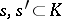 , it turns out that the space
, it turns out that the space  can be mapped homeomorphically (can be imbedded) into
can be mapped homeomorphically (can be imbedded) into  (where
(where  may be transfinite), so that all closed cells
may be transfinite), so that all closed cells 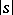 are (rectilinear) simplices. This means that the image of
are (rectilinear) simplices. This means that the image of 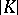 in
in 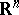 is a simplicial space (a polyhedron), i.e. a union of closed simplices intersecting only on entire faces. This simplicial space is called a realization of the simplicial complex
is a simplicial space (a polyhedron), i.e. a union of closed simplices intersecting only on entire faces. This simplicial space is called a realization of the simplicial complex 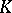 in
in 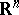 .
.
A simplicial complex 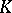 can only be realized in
can only be realized in  for finite
for finite  when
when 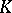 is locally finite, at most countable and of finite dimension. Moreover, if
is locally finite, at most countable and of finite dimension. Moreover, if 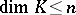 , then
, then 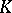 can be realized in
can be realized in 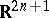 . A simplicial complex consisting of
. A simplicial complex consisting of 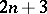 vertices every
vertices every 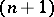 -element subset of which is a simplex cannot be realized in
-element subset of which is a simplex cannot be realized in 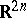 .
.
From any simplicial complex 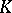 one can construct a new simplicial complex,
one can construct a new simplicial complex, 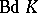 , whose vertices are the simplices of
, whose vertices are the simplices of 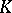 and whose simplices are families
and whose simplices are families 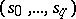 of simplices of
of simplices of 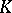 such that
such that 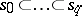 .
. 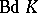 is called the barycentric refinement (or subdivision) of
is called the barycentric refinement (or subdivision) of 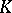 . The cellular spaces
. The cellular spaces 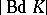 and
and 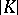 are naturally homeomorphic (but not isomorphic). Under this homeomorphism, every vertex
are naturally homeomorphic (but not isomorphic). Under this homeomorphism, every vertex 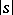 of
of  (that is, the zero-dimensional cell corresponding to the vertex
(that is, the zero-dimensional cell corresponding to the vertex  of
of 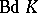 ) is mapped onto the centre of gravity (the barycentre) of the closed simplex
) is mapped onto the centre of gravity (the barycentre) of the closed simplex 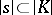 .
.
The simplicial complex 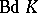 is ordered in a natural way. If
is ordered in a natural way. If 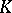 is ordered, then the correspondence
is ordered, then the correspondence 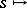 (first vertex of
(first vertex of  ) defines a simplicial mapping
) defines a simplicial mapping 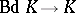 that preserves the ordering. It is called the canonical translation. Its geometric realization (which is a continuous mapping
that preserves the ordering. It is called the canonical translation. Its geometric realization (which is a continuous mapping 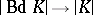 ) is homotopic to the natural homeomorphism
) is homotopic to the natural homeomorphism 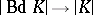 .
.
A simplicial mapping 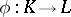 (or its geometric realization
(or its geometric realization 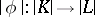 ) is called a simplicial approximation of a continuous mapping
) is called a simplicial approximation of a continuous mapping 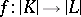 if, for every point
if, for every point 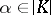 , the point
, the point 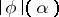 belongs to the minimal closed simplex containing the point
belongs to the minimal closed simplex containing the point  , or, equivalently, if for every vertex
, or, equivalently, if for every vertex 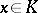 ,
, 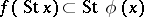 . The mappings
. The mappings 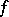 and
and  are homotopic.
are homotopic.
The simplicial approximation theorem states that if a simplicial complex 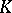 is finite, then for every continuous mapping
is finite, then for every continuous mapping 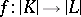 there is an integer
there is an integer 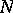 such that for all
such that for all 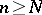 there is a simplicial approximation
there is a simplicial approximation 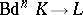 of
of 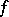 (regarded as a mapping
(regarded as a mapping  ).
).
References
| [1] | E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966) |
| [2] | P.J. Hilton, S. Wylie, "Homology theory. An introduction to algebraic topology" , Cambridge Univ. Press (1960) |
| [3] | J.H.C. Whitehead, "Simplicial spaces, nuclei and $M$-groups" Proc. London Math. Soc. , 45 (1939) pp. 243–327 |
Comments
In the West, the concept described here is usually called an (abstract) simplicial complex; the term simplicial scheme would normally be understood to mean a simplicial object in the category of schemes (cf. Simplicial object in a category).
References
| [a1] | C.R.F. Maunder, "Algebraic topology" , v. Nostrand (1972) |
| [a2] | S. Lefshetz, "Topology" , Chelsea, reprint (1956) |
| [a3] | K. Lamotke, "Semisimpliziale algebraische Topologie" , Springer (1968) |
Comments
A facet of an abstract simplicial complex is a maximal face. A complex is pure if all facets have the same dimension.
For a face $F$ of a simplicial complex $K$, we let $F^\Delta$ denote all faces contained in $F$. A shelling is a linear order $\sqsubseteq$ on the facets of $K$, such that for a facet $F$, $$ \bigcup_{G \sqsubset F} G^\Delta \cap F^\Delta $$ is a subcomplex generated by the codimension 1 faces of $F$. A complex is shellable if it is pure and possesses a shelling (some authors omit the requirement to be pure). If a complex is shellable then its face ring is Cohen–Macaulay.
References
| [b1] | Ezra Miller, Bernd Sturmfels, "Combinatorial commutative algebra" Graduate Texts in Mathematics 227 Springer (2005) ISBN 0-387-23707-0 Zbl 1090.13001 |
| [b2] | Richard P. Stanley, "Combinatorics and commutative algebra" , (2nd ed.)mBirkhäuser (1996) ISBN 0-81764-369-9 Zbl 1157.13302 Zbl 0838.13008 |
Simplicial complex. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Simplicial_complex&oldid=55462