Telegraph equation
The partial differential equation
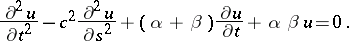 | (1) |
This equation is satisfied by the intensity of the current in a conductor, considered as a function of time 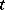 and distance
and distance  from any fixed point of the conductor. Here,
from any fixed point of the conductor. Here,  is the speed of light,
is the speed of light, 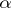 is a capacity coefficient and
is a capacity coefficient and 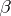 is the induction coefficient.
is the induction coefficient.
By the transformation
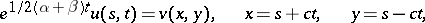 |
equation (1) is reduced to the form
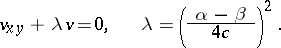 | (2) |
This equation belongs to the class of hyperbolic equations of the second order (cf. Hyperbolic partial differential equation),
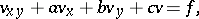 |
in the theory of which an important part is played by the Riemann function 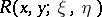 . For equation (2) this function can be written in the explicit form
. For equation (2) this function can be written in the explicit form
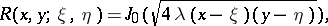 |
where  is the Bessel function (cf. Bessel functions).
is the Bessel function (cf. Bessel functions).
References
| [1] | R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) |
Comments
The special case 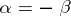 is treated in [a1].
is treated in [a1].
References
| [a1] | F. John, "Partial differential equations" , Springer (1978) |
Telegraph equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Telegraph_equation&oldid=48953