Conjugate elements
in a group 
Elements  and
and  of
of  for which
for which
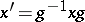 |
for some  in
in  . One also says that
. One also says that  is the result of conjugating
is the result of conjugating  by
by 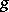 . The power notation
. The power notation 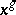 is frequently used for the conjugate of
is frequently used for the conjugate of  under
under  .
.
Let 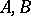 be two subsets of a group
be two subsets of a group  , then
, then 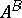 denotes the set
denotes the set
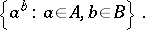 |
For some fixed 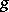 in
in 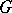 and some subset
and some subset 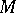 of
of 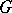 the set
the set  is said to be conjugate to the set
is said to be conjugate to the set  in
in 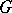 . In particular, two subgroups
. In particular, two subgroups 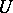 and
and  are called conjugate subgroups if
are called conjugate subgroups if 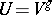 for some
for some 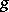 in
in 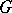 . If a subgroup
. If a subgroup  coincides with
coincides with 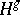 for every
for every  (that is,
(that is,  consists of all conjugates of all its elements), then
consists of all conjugates of all its elements), then  is called a normal subgroup of
is called a normal subgroup of  (or an invariant subgroup, or, rarely, a self-conjugate subgroup).
(or an invariant subgroup, or, rarely, a self-conjugate subgroup).
Comments
The map $x \mapsto g^{-1} x g$ for given $g$ is conjugation by $g$: it is an inter automorphism of $G$.
References
| [a1] | B. Huppert, "Endliche Gruppen" , 1 , Springer (1967) |
| [a2] | D. Gorenstein, "Finite groups" , Chelsea, reprint (1980) |
Conjugate elements. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Conjugate_elements&oldid=35117