Poincaré space
A Poincaré space of formal dimension  is a topological space
is a topological space  in which is given an element
in which is given an element 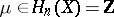 such that the homomorphism
such that the homomorphism 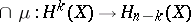 given by
given by  is an isomorphism for each
is an isomorphism for each 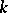 (here
(here 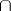 is Whitney's product operation, the cap product). Moreover,
is Whitney's product operation, the cap product). Moreover, 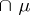 is called the Poincaré duality isomorphism and the element
is called the Poincaré duality isomorphism and the element 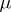 generates the group
generates the group 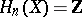 . Any closed orientable
. Any closed orientable  -dimensional connected topological manifold is a Poincaré space of formal dimension
-dimensional connected topological manifold is a Poincaré space of formal dimension  ;
; 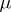 is taken to be an orientation (the fundamental class) of the manifold.
is taken to be an orientation (the fundamental class) of the manifold.
Let  be a finite cellular space imbedded in a Euclidean space
be a finite cellular space imbedded in a Euclidean space 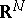 of large dimension
of large dimension 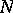 , let
, let 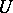 be a closed regular neighbourhood of this imbedding and let
be a closed regular neighbourhood of this imbedding and let 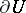 be its boundary. The standard mapping
be its boundary. The standard mapping 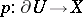 turns out to be a (Serre) fibration.
turns out to be a (Serre) fibration.  :
: 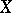 is a Poincaré space of formal dimension
is a Poincaré space of formal dimension  if and only if the fibre of this fibration is homotopy equivalent to the sphere
if and only if the fibre of this fibration is homotopy equivalent to the sphere 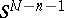 . The described fibration which arises when
. The described fibration which arises when 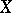 is a Poincaré space (the fibre of which is a sphere) is unique up to the standard equivalence and is called the normal spherical fibration, or the Spivak fibration, of the Poincaré space
is a Poincaré space (the fibre of which is a sphere) is unique up to the standard equivalence and is called the normal spherical fibration, or the Spivak fibration, of the Poincaré space 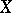 . Moreover, the cone of the projection
. Moreover, the cone of the projection 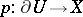 is the Thom space of the normal spherical fibration over
is the Thom space of the normal spherical fibration over  .
.
If one restricts just to homology with coefficients in a certain field  , then a so-called Poincaré space over
, then a so-called Poincaré space over 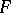 is obtained.
is obtained.
One also considers Poincaré pairs 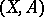 (generalizations of the concept of a manifold with boundary), where for a certain generator
(generalizations of the concept of a manifold with boundary), where for a certain generator 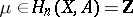 and any
and any 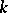 there is a Poincaré duality isomorphism:
there is a Poincaré duality isomorphism:
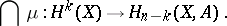 |
Poincaré spaces naturally arise in problems on the existence and the classification of structures on manifolds. The problem of smoothing (triangulation) of a Poincaré space is also interesting, that is, to find a smooth (piecewise-linear), closed manifold that is homotopy equivalent to a given Poincaré space.
Sometimes, by  -dimensional Poincaré space one means a closed
-dimensional Poincaré space one means a closed  -dimensional manifold
-dimensional manifold 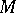 with homology groups (cf. Homology group)
with homology groups (cf. Homology group) 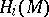 isomorphic to the homology groups
isomorphic to the homology groups 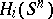 of the
of the  -dimensional sphere
-dimensional sphere 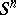 ; these are also called homology spheres.
; these are also called homology spheres.
A simply-connected Poincaré space is homotopy equivalent to a sphere. For a group 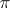 that is realizable as the fundamental group of a certain Poincaré space one has
that is realizable as the fundamental group of a certain Poincaré space one has 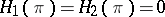 , where
, where 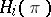 are the homology groups of the group
are the homology groups of the group 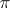 . Conversely, for any
. Conversely, for any 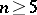 and any finitely-presented group
and any finitely-presented group  with
with 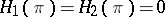 there exist an
there exist an  -dimensional Poincaré space
-dimensional Poincaré space 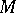 with
with 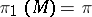 .
.
For  these conditions are insufficient to realize the group
these conditions are insufficient to realize the group 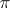 in the form
in the form 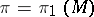 . So, for example, the fundamental group of any three-dimensional Poincaré space admits a presentation with the same number of generators and relations. The only finite group which is realizable as the fundamental group of a three-dimensional Poincaré space is the binary icosahedral group
. So, for example, the fundamental group of any three-dimensional Poincaré space admits a presentation with the same number of generators and relations. The only finite group which is realizable as the fundamental group of a three-dimensional Poincaré space is the binary icosahedral group 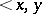 :
: 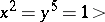 , which is the fundamental group of the dodecahedral space — historically the first example of a Poincaré space.
, which is the fundamental group of the dodecahedral space — historically the first example of a Poincaré space.
References
| [1] | W.B. Browder, "Surgery on simply connected manifolds" , Springer (1972) |
Poincaré space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Poincar%C3%A9_space&oldid=22943