Component of a space
From Encyclopedia of Mathematics
A connected subset C of a topological space X with the following property: If  is a connected subset such that
is a connected subset such that  , then
, then  . The components of a space are disjoint. Every non-empty connected subset is contained in exactly one component. If
. The components of a space are disjoint. Every non-empty connected subset is contained in exactly one component. If 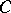 is a component of a space
is a component of a space 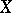 and
and 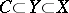 , then
, then 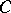 is a component of
is a component of 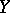 . If
. If  is a monotone continuous mapping onto, then
is a monotone continuous mapping onto, then 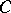 is a component of
is a component of 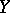 if and only if
if and only if 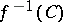 is a component of
is a component of 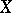 .
.
References
| [1] | K. Kuratowski, "Topology" , 2 , Acad. Press (1968) (Translated from French) |
How to Cite This Entry:
Component of a space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Component_of_a_space&oldid=19324
Component of a space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Component_of_a_space&oldid=19324
This article was adapted from an original article by B.A. Efimov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article