Gaussian curvature
of a surface
The product of the principal curvatures (cf. Principal curvature) of a regular surface at a given point.
If
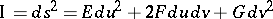 |
is the first fundamental form of the surface and
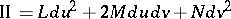 |
is the second fundamental form of the surface, then the Gaussian curvature can be computed by the formula
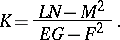 |
The Gaussian curvature is identical with the Jacobi determinant of the spherical map:
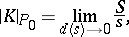 |
where 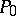 is a point on the surface,
is a point on the surface,  is the area of a domain
is the area of a domain 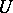 which contains
which contains 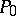 ,
, 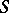 is the area of the spherical image of
is the area of the spherical image of  , and
, and 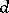 is the diameter of the domain. The Gaussian curvature is positive at an elliptic point, negative at a hyperbolic point, and is zero at a parabolic point or a flat point. It may be expressed in terms of the coefficients of the first fundamental form and their derivatives alone (the Gauss theorem), viz.
is the diameter of the domain. The Gaussian curvature is positive at an elliptic point, negative at a hyperbolic point, and is zero at a parabolic point or a flat point. It may be expressed in terms of the coefficients of the first fundamental form and their derivatives alone (the Gauss theorem), viz.
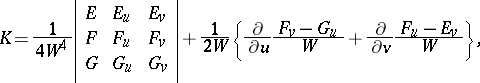 |
where
 |
Since the Gaussian curvature depends on the metric only, i.e. on the coefficients of the first fundamental form, the Gaussian curvature is invariant under isometric deformation (cf. Deformation, isometric). The Gaussian curvature plays a special role in the theory of surfaces, and many formulas are available for its computation, [2].
The concept was introduced by C.F. Gauss [1], and was named after him.
References
| [1] | C.F. Gauss, "Allgemeine Flächentheorie" , W. Engelmann , Leipzig (1900) (Translated from Latin) Zbl 31.0599.11 |
| [2] | H. Reichardt, "Einführung in die Differentialgeometrie" , Springer (1960) MR0116267 Zbl 0091.34001 |
Comments
The total Gaussian curvature (often also abbreviated to total curvature) is the quantity
 |
(See also Gauss–Bonnet theorem.)
For a smooth space curve 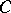 given by
given by 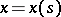 , the total curvature
, the total curvature 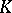 of
of 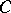 is defined as the length of the spherical image of
is defined as the length of the spherical image of 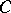 (cf. also Spherical indicatrix) and can be expressed using the Frénet formulas
(cf. also Spherical indicatrix) and can be expressed using the Frénet formulas 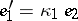 ,
, 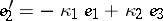 ,
, 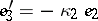 for a Frénet frame (cf. Frénet trihedron)
for a Frénet frame (cf. Frénet trihedron) 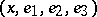 along
along 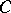 by
by
 |
Gaussian curvature. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gaussian_curvature&oldid=19268