Difference between revisions of "Group algebra of a locally compact group"
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
m (fixing superscript) |
||
| Line 17: | Line 17: | ||
be defined as the convolution $ ( f _ {1} , f _ {2} ) \rightarrow f _ {1} \star f _ {2} $; | be defined as the convolution $ ( f _ {1} , f _ {2} ) \rightarrow f _ {1} \star f _ {2} $; | ||
also, let the involution $ f \rightarrow f ^ { * } $ | also, let the involution $ f \rightarrow f ^ { * } $ | ||
| − | be given by the formula $ f ^ { * } ( g) = \overline{ {f ( g ^ {-} | + | be given by the formula $ f ^ { * } ( g) = \overline{ {f ( g ^ {- 1} ) }}\; \Delta ( g) $, |
where $ \Delta $ | where $ \Delta $ | ||
is the modular function of $ G $. | is the modular function of $ G $. | ||
| Line 69: | Line 69: | ||
is an algebra under the usual [[pointwise multiplication]] (see [[#References|[a1]]], (32.10)). For all non-discrete locally compact Abelian groups $ G $ | is an algebra under the usual [[pointwise multiplication]] (see [[#References|[a1]]], (32.10)). For all non-discrete locally compact Abelian groups $ G $ | ||
there exist continuous compactly-supported functions on $ G $ | there exist continuous compactly-supported functions on $ G $ | ||
| − | that are not in $ B ( G) $( | + | that are not in $ B ( G) $ (see [[#References|[a1]]], (33.3) and (41.19)). Thus $ K ( G) $ |
| − | see [[#References|[a1]]], (33.3) and (41.19)). Thus $ K ( G) $ | ||
is not a subset of $ B ( G) $. | is not a subset of $ B ( G) $. | ||
Latest revision as of 10:16, 8 May 2022
A topological algebra with involution formed by certain functions on the group with multiplication in it defined as convolution. Let the Banach space $ L _ {1} ( G) $
be constructed using a left-invariant Haar measure $ d g $
on a locally compact topological group $ G $
and let the multiplication in $ L _ {1} ( G) $
be defined as the convolution $ ( f _ {1} , f _ {2} ) \rightarrow f _ {1} \star f _ {2} $;
also, let the involution $ f \rightarrow f ^ { * } $
be given by the formula $ f ^ { * } ( g) = \overline{ {f ( g ^ {- 1} ) }}\; \Delta ( g) $,
where $ \Delta $
is the modular function of $ G $.
The resulting Banach algebra with involution is said to be the group algebra of $ G $
and is also denoted by $ L _ {1} ( G) $.
If $ G $
is a finite group, then the definition of the group algebra coincides with the ordinary algebraic definition of the group algebra over the field of complex numbers.
The concept of a group algebra makes it possible to use the general methods of the theory of Banach algebras in problems of group theory and, in particular, in abstract harmonic analysis. The properties of a group algebra, as a Banach algebra, reflect the properties of topological groups; thus, a group algebra contains a unit element if and only if the group is discrete; the group algebra is the direct (topological) sum of its finite-dimensional minimal two-sided ideals if and only if the group is compact. Of special importance is the concept of a group algebra in the theory of unitary representations (cf. Unitary representation) of groups: Between the continuous unitary representations of a topological group $ G $ and the non-degenerate symmetric representations (cf. Involution representation) of the group algebra $ L _ {1} ( G) $ there exists a one-to-one correspondence. This correspondence puts a continuous unitary representation $ \pi $ of $ G $ in a Hilbert space $ H $ into correspondence with the representation of $ L _ {1} ( G) $ defined by
$$ f \rightarrow \int\limits _ { G } f ( g) \pi ( g) dg,\ \ f \in L _ {1} ( G). $$
Group algebras of locally compact groups have a number of properties in common. In fact, any group algebra contains an approximate unit element (cf. Banach algebra), formed by the family of characteristic functions on a neighbourhood of the unit element ordered by inclusion (in decreasing order). For this reason it is possible to establish for a group algebra a correspondence between the positive functionals on the group algebra and its symmetric representations. Any group algebra is a semi-simple algebra, and has a symmetric faithful representation. In particular, the representation of a group algebra determined by the regular representation of the group is faithful. The closed left ideals of $ L _ {1} ( G) $ are the closed vector subspaces of $ L _ {1} ( G) $ that are invariant with respect to left translation.
The name group algebra is also sometimes given to the Banach algebra with involution obtained from the group algebra $ L _ {1} ( G) $ by the adjunction of a unit. There exist several other algebras with involutions, which are sometimes referred to as group algebras. These include, in particular: the algebra of measures $ M ( G) $ with respect to convolution, algebras with respect to ordinary multiplication, such as the algebra $ L _ \infty ( G) $ of essentially-bounded functions that are measurable by the Haar measure, and the algebra $ P ( G) $ spanned by the set of complex positive-definite functions. The set $ P _ {1} ( G) = P ( G) \cap L _ {1} ( G) $ and the set $ K ( G) $ of continuous functions with compact support form algebras both with respect to convolution and with respect to ordinary multiplication. One obtains the following table, in which arrows denote inclusions:
$$ \begin{array}{rcl} {} &P _ {1} & \rightarrow P \rightarrow L _ \infty \\ {} &\downarrow &{} \\ K \rightarrow &L _ {1} & \rightarrow M \\ \end{array} . $$
References
| [1] | M.A. Naimark, "Normed rings" , Reidel (1984) (Translated from Russian) |
| [2] | A. Guichardet, "Analyse harmonique commutative" , Dunod (1968) |
Comments
The set of all complex continuous positive-definite functions on $ G $ is not an algebra since 1 is in it but $ - 1 $ is not. However, the set of all complex linear combinations of its members, which is usually denoted $ B ( G) $, is an algebra under the usual pointwise multiplication (see [a1], (32.10)). For all non-discrete locally compact Abelian groups $ G $ there exist continuous compactly-supported functions on $ G $ that are not in $ B ( G) $ (see [a1], (33.3) and (41.19)). Thus $ K ( G) $ is not a subset of $ B ( G) $.
For the notion of the modular function $ \Delta $ of $ G $, cf. Haar measure. A symmetric representation $ \pi $ of an algebra with involution in a Hilbert space over $ \mathbf C $ is one which satisfies $ \pi ( a ^ {*} ) = \pi ( a) ^ {*} $ for all $ a \in A $. Here the $ * $ on the right denotes taking adjoints and the $ * $ on the left refers to the involution on $ A $.
References
| [a1] | E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , 1–2 , Springer (1979) |
| [a2] | J. Dixmier, "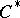 algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
Group algebra of a locally compact group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Group_algebra_of_a_locally_compact_group&oldid=52329