Difference between revisions of "Hille-Tamarkin operator"
From Encyclopedia of Mathematics
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Hille–Tamarkin operator to Hille-Tamarkin operator: ascii title) |
(No difference)
| |
Revision as of 18:52, 24 March 2012
Let  be an integral operator from
be an integral operator from  into
into 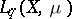 , i.e., there exists a
, i.e., there exists a  -measurable function
-measurable function 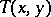 on
on  such that
such that 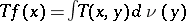 a.e. on
a.e. on 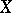 . Then
. Then  is called a Hille–Tamarkin operator if
is called a Hille–Tamarkin operator if
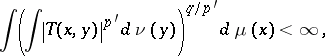 |
where 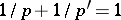 . By taking
. By taking 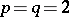 one obtains the class of Hilbert–Schmidt operators (cf. Hilbert–Schmidt operator). Replacing
one obtains the class of Hilbert–Schmidt operators (cf. Hilbert–Schmidt operator). Replacing 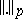 , respectively
, respectively 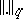 , by arbitrary Banach function norms
, by arbitrary Banach function norms 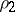 , respectively
, respectively 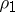 , one obtains the class of Hille–Tamarkin operators between Banach function spaces (sometimes called integral operators of finite double norm, see [a1]). Under some mild hypotheses on the norms, one can show that Hille–Tamarkin operators have rather strong compactness properties (see [a2]).
, one obtains the class of Hille–Tamarkin operators between Banach function spaces (sometimes called integral operators of finite double norm, see [a1]). Under some mild hypotheses on the norms, one can show that Hille–Tamarkin operators have rather strong compactness properties (see [a2]).
References
| [a1] | A.C. Zaanen, "Riesz spaces" , II , North-Holland (1983) |
| [a2] | A.R. Schep, "Compactness properties of Carleman and Hille–Tamarkin operators" Canad. J. Math. , 37 (1985) pp. 921–933 |
How to Cite This Entry:
Hille-Tamarkin operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hille-Tamarkin_operator&oldid=22579
Hille-Tamarkin operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hille-Tamarkin_operator&oldid=22579
This article was adapted from an original article by A.R. Schep (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article