White noise

A generalized stationary stochastic process with constant spectral density. The generalized correlation function of white noise has the form , where is a positive constant and is the delta-function. The white noise process is extensively applied in describing random disturbances with a very small correlation period (e.g. "thermal noisethermal noise" — pulsations of the current intensity in a conductor, generated by the thermal motion of the electrons). In the spectral decomposition of white noise,

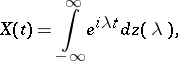the "elementary vibrations" have, on the average, the same intensity at all frequencies ; more accurately, their average squared amplitude is

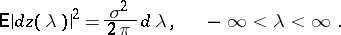This spectral decomposition means that, for each square-integrable function ,

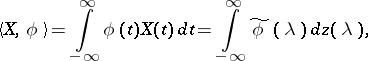where is the Fourier transform of ; a more explicit dependence of the generalized process on the function may be described by a corresponding stochastic measure 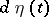of the same type as ( is the Fourier transform of the stochastic measure 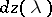), viz.

Gaussian white noise , which is the generalized derivative of Brownian motion (), is the basis for constructing stochastic diffusion processes 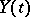(cf. Diffusion process), "controllable" by a stochastic differential equation:

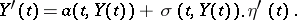These equations are often written in the form of differentials:

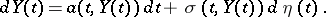Another important model involving the use of white noise is the stochastic process which describes the behaviour of a stable vibrating system acted upon by stationary random perturbations , when , , 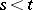do not depend on , . A very simple example of this is the system

where is a polynomial with roots in the left half-plane; after the damping of the "transient processes" , the process is given by

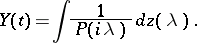In practical applications, in the description of the so-called shot effect process, white noise of the form

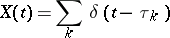plays an important role ( varies between and 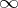and the 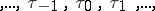form a Poisson process); more accurately, 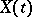is the generalized derivative of a Poisson process . The shot effect process itself has the form

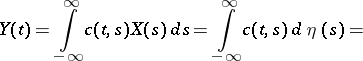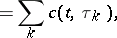where is some weight function satisfying the condition

in addition, the average value of the generalized proces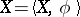s is

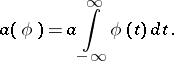Here, is the parameter of the Poisson law (see above), and the stochastic measure 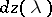in the spectral representation

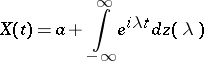of this process is such that

References

[1]	Yu.V. [Yu.V. Prokhorov] Prohorov, Yu.A. Rozanov, "Probability theory, basic concepts. Limit theorems, random processes" , Springer (1969) (Translated from Russian)

Comments

See [a1] for applications of white noise as the limit of "wide bandwidth" noise in physical systems and [a2] for the relationship between differential equations with white noise inputs and the stochastic differential equations of Itô calculus (cf. also Itô formula; Stochastic differential equation). See also Stratonovich integral for further information on this topic. Further important topics are the analysis of white noise regarded as a generalized random function [a3], i.e. a probability on the space of tempered distributions on (cf. White noise analysis), and application of white noise theory in non-linear filtering [a4], where "white noise" is interpreted in terms of finitely-additive Gaussian measures on cylinder sets of a separable Hilbert space.

References

[a1]	H.J. Kushner, "Approximation and weak convergence methods for random processes, with applications to stochastic systems theory" , M.I.T. (1984)
[a2]	N. Ikeda, S. Watanabe, "Stochastic differential equations and diffusion processes" , North-Holland & Kodansha (1988)
[a3]	T. Hida, "Brownian motion" , Springer (1980)
[a4]	G. Kallianpur, R.L. Karandikar, "White noise theory of prediction, filtering and smoothing" , Gordon & Breach (1988)
[a5]	I.M. Gel'fand, N.Ya. Vilenkin, "Generalized functions. Applications of harmonic analysis" , 4 , Acad. Press (1968) pp. Chapt. III (Translated from Russian)
[a6]	T. Hida (ed.) H.-H. Kuo (ed.) J. Potthoff (ed.) L. Streid (ed.) , White noise analysis - mathematics and applications , World Sci. (1990)