Walsh system
of functions  on the interval
on the interval 
The functions  and
and  for
for  , where
, where  ,
,  are the Rademacher functions (cf. Rademacher system) and
are the Rademacher functions (cf. Rademacher system) and  ,
,  , is the binary representation of the number
, is the binary representation of the number  . This system was defined and studied by J.L. Walsh [1], but already in 1900 J.A. Barrett studied functions of this system in questions connected with the distribution of electrons on open conducting curves. In connection with this theory another definition of Walsh functions is preferred. Namely, if
. This system was defined and studied by J.L. Walsh [1], but already in 1900 J.A. Barrett studied functions of this system in questions connected with the distribution of electrons on open conducting curves. In connection with this theory another definition of Walsh functions is preferred. Namely, if
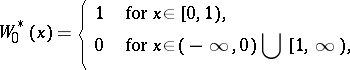 |
then the functions 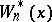 are defined by the following recurrence formulas:
are defined by the following recurrence formulas:
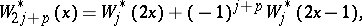 |
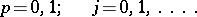 |
The systems  and
and 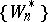 differ only in their ordering in the ranges
differ only in their ordering in the ranges 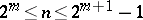 ,
, 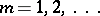 . For example,
. For example,  ,
,  ,
, 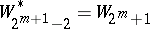 , etc. The index
, etc. The index  of the function
of the function  corresponds to the number of changes of sign of this function in the interval
corresponds to the number of changes of sign of this function in the interval 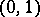 , i.e. it is the analogue to doubling the frequency of a sinusoidal function. The Walsh system is a complete orthonormal system on the interval
, i.e. it is the analogue to doubling the frequency of a sinusoidal function. The Walsh system is a complete orthonormal system on the interval 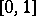 and it may be considered as a natural completion of the Rademacher system.
and it may be considered as a natural completion of the Rademacher system.
The Walsh system forms a commutative multiplicative group, with the function 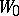 as unit element, while each
as unit element, while each 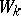 is its own inverse.
is its own inverse.
References
| [1] | J.L. Walsh, "A closed set of normal orthogonal functions" Amer. J. Math. , 45 (1923) pp. 5–24 |
| [2] | F.F. Fowle, Trans. AJEE , 23 (1905) pp. 659–687 |
| [3] | N.J. Fine, "On the Walsh functions" Trans. Amer. Math. Soc. , 65 (1949) pp. 372–414 |
| [4] | S. Kaczmarz, H. Steinhaus, "Theorie der Orthogonalreihen" , Chelsea, reprint (1951) |
| [5] | H.F. Harmut, "Transmission of information by orthogonal functions" , Springer (1972) |
Comments
References
| [a1] | B. Golubov, A. Efimov, V. Skvortsov, "Walsh series and transforms" , Kluwer (1987) (Translated from Russian) |
Walsh system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Walsh_system&oldid=14844