Virasoro algebra
A Lie algebra, denoted by 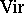 , over
, over  with basis
with basis 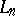 (
(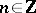 ),
),  and the following commutation relations (
and the following commutation relations (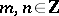 ):
):
 |
 |
Since the vector fields  (
(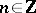 ) on
) on 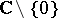 satisfy the relation
satisfy the relation 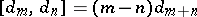 , the Lie algebra
, the Lie algebra  is a central extension (which is, in fact, universal) of the Lie algebra of holomorphic vector fields on the punctured complex plane having finite Laurent series. For this reason the Virasoro algebra plays a key role in conformal field theory.
is a central extension (which is, in fact, universal) of the Lie algebra of holomorphic vector fields on the punctured complex plane having finite Laurent series. For this reason the Virasoro algebra plays a key role in conformal field theory.
On the other hand, letting 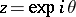 , where
, where 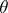 is the parameter on the unit circle
is the parameter on the unit circle 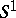 , one gets
, one gets 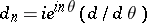 . Hence the Lie algebra of vector fields on
. Hence the Lie algebra of vector fields on 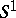 with finite Fourier series is a real form of the Lie algebra
with finite Fourier series is a real form of the Lie algebra  consisting of elements fixed under the anti-linear involution
consisting of elements fixed under the anti-linear involution 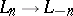 ,
, 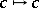 . For this reason the Virasoro algebra is intimately related to the representation theory of the group of diffeomorphisms of
. For this reason the Virasoro algebra is intimately related to the representation theory of the group of diffeomorphisms of 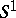 , of the loop groups and to affine Kac–Moody algebras (see Kac–Moody algebra).
, of the loop groups and to affine Kac–Moody algebras (see Kac–Moody algebra).
The representation theory of the Virasoro algebra has numerous applications in mathematics and theoretical physics. The most interesting, positive-energy representations of  in a complex vector space
in a complex vector space 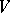 , are defined by the property that
, are defined by the property that  acts as a scalar, denoted by the same letter
acts as a scalar, denoted by the same letter  (called the central charge), and that
(called the central charge), and that 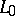 (the energy operator) is diagonalizable with finite-dimensional eigenspaces and with real spectrum bounded below:
(the energy operator) is diagonalizable with finite-dimensional eigenspaces and with real spectrum bounded below:
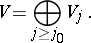 |
The character of such a representation is the (formal) series
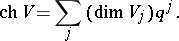 |
The first positive-energy representations of 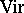 were implicitly constructed by M.A. Virasoro [a1] in 1970, using an Abelian version of the Sugawara construction (see Kac–Moody algebra) in the framework of string theory. Since that time, and especially since the proof of the no-ghost theorem [a2], the representation theory of the Virasoro algebra has become a key ingredient of string theory (see [a3]). The Virasoro central extension itself was previously discovered by mathematicians [a4], [a5]; paper [a2] is one of the earliest references in the physics literature containing a correct formula for the central term.
were implicitly constructed by M.A. Virasoro [a1] in 1970, using an Abelian version of the Sugawara construction (see Kac–Moody algebra) in the framework of string theory. Since that time, and especially since the proof of the no-ghost theorem [a2], the representation theory of the Virasoro algebra has become a key ingredient of string theory (see [a3]). The Virasoro central extension itself was previously discovered by mathematicians [a4], [a5]; paper [a2] is one of the earliest references in the physics literature containing a correct formula for the central term.
An irreducible positive-energy representation of 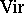 in a vector space
in a vector space 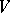 admits a non-zero vector
admits a non-zero vector 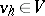 , where
, where  , such that
, such that
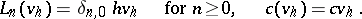 | (a1) |
Then one has:
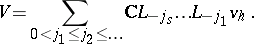 | (a2) |
This representation is determined uniquely by the two real constants  , the central charge, and
, the central charge, and 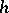 , the conformal dimension, and is denoted by
, the conformal dimension, and is denoted by 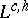 . It is called degenerate if (a2) is not a direct sum decomposition.
. It is called degenerate if (a2) is not a direct sum decomposition.
The first basic result of the representation theory of 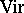 [a6] (see [a7] for a proof) states that
[a6] (see [a7] for a proof) states that  is degenerate if and only if
is degenerate if and only if 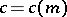 ,
,  for some
for some 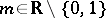 and some
and some  where
where
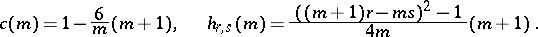 |
The basic idea of the foundational work [a8] on conformal field theory is to use degenerate representations of 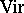 to write down differential equations for correlation functions. The most complete results have been obtained [a9] for the "most degenerate" representations, called the minimal series representations. These correspond to
to write down differential equations for correlation functions. The most complete results have been obtained [a9] for the "most degenerate" representations, called the minimal series representations. These correspond to 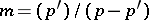 , where
, where 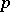 and
and  are relatively prime positive integers and
are relatively prime positive integers and 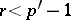 ,
,  [a8].
[a8].
The characters  were computed in [a10]. It follows that after letting
were computed in [a10]. It follows that after letting  , the function
, the function  becomes a modular function in
becomes a modular function in  on the upper half-plane for some
on the upper half-plane for some 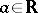 if and only if
if and only if 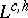 is a representation of minimal series (then
is a representation of minimal series (then 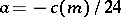 ) [a11].
) [a11].
The representation 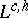 carries a unique Hermitian form such that
carries a unique Hermitian form such that 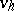 has norm
has norm 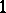 and the operators
and the operators 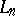 and
and 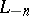 are adjoint. Another important class of representations
are adjoint. Another important class of representations 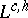 are the unitary ones, i.e. those for which the Hermitian form is positive definite. The complete list of unitary representations is (see [a12], [a13], [a7]):
are the unitary ones, i.e. those for which the Hermitian form is positive definite. The complete list of unitary representations is (see [a12], [a13], [a7]):
a) 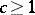 ,
,  ;
;
b) minimal series with 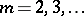 .
.
The minimal series representations (especially the unitary ones) are intimately related to statistical lattice models (see [a14]). For example, the case 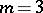 is identified with the Ising model,
is identified with the Ising model,  with the Potts model, etc.
with the Potts model, etc.
Among other areas of applications of the Virasoro algebra one should mention the theory of moduli spaces of curves (see [a15]–[a18]).
References
| [a1] | M.M. Virasoro, "Subsidary conditions and ghosts in dual-resonance models" Phys. Rev. , D1 (1970) pp. 2933–2936 |
| [a2] | P. Goddard, C.B. Thorn, "Compatibility of the dual Pomeron with unitarity and the absence of ghosts in the dual resonance model" Phys. Lett. , 4 (1972) pp. 235–238 |
| [a3] | M.B. Green, J.H. Schwarz, E. Witten, "Superstring theory" , Cambridge Univ. Press (1987) |
| [a4] | R.E. Block, "On the Mills–Seligman axioms for Lie algebras of classical type" Trans. Amer. Math. Soc. , 121 (1966) pp. 378–392 |
| [a5] | I.M. Gel'fand, D.B. Fuks, "The cohomology of the Lie algebra of vector fields in a circle" Funct. Anal. Appl. , 2 (1968) pp. 342–343 Funkts. Anal. i Prilozh. , 2 : 4 (1968) pp. 92–93 |
| [a6] | V.G. Kac, "Highest weight representations of infinite dimensional Lie algebras" , Proc. Internat. Congress Mathematicians (Helsinki, 1978) , 1 , Acad. Sci. Fennicae (1980) pp. 299–304 |
| [a7] | V.G. Kac, A.K. Raina, "Bombay lectures on highest weight representations" , World Sci. (1987) |
| [a8] | A.A. Belavin, A.M. Polyakov, A.B. Zamolodchikov, "Infinite conformal symmetry in two-dimensional quantum field theory" Nuclear Phys. , B241 (1984) pp. 333–380 |
| [a9] | G. Felder, "BRST approach to minimal models" Nuclear Phys. , B317 (1989) pp. 215–236 |
| [a10] | B.L. Feigin, D.B. [D.B. Fuks] Fuchs, "Verma models over the Virasoro algebra" L.D. Faddeev (ed.) A.A. Mal'tsev (ed.) , Topology. Proc. Internat. Topol. Conf. Leningrad 1982 , Lect. notes in math. , 1060 , Springer (1984) pp. 230–245 |
| [a11] | V.G. Kac, M. Wakimoto, "Modular invariant representations of infinite-dimensional Lie algebras and superalgebras" Proc. Nat. Acad. Sci. USA , 85 (1988) pp. 4956–4960 |
| [a12] | D. Friedan, Z. Qui, S. Shenker, "Conformal invariance, unitary and two dimensional critical exponents" Publ. MSRI , 3 (1985) pp. 419–449 |
| [a13] | P. Goddard, A. Kent, D. Olive, "Unitary representations of the Virasoro and super-Virasoro algebras" Comm. Math. Phys. , 103 (1986) pp. 105–119 |
| [a14] | C. Itzykson, J.-M. Dronfree, "Statistical field theory" , Cambridge Univ. Press (1989) |
| [a15] | E. Arbarello, C. De Concini, V.G. Kac, C. Processi, "Moduli spaces of curves and representation theory" Comm. Math. Phys. , 117 (1988) pp. 1–36 |
| [a16] | M.L. Kontzevich, "Virasoro algebra and Teichmüller spaces" Funct. Anal. Appl. , 21 : 2 (1987) pp. 156–157 Funkts. Anal. i Prilozh. , 21 : 2 (1987) pp. 78–79 |
| [a17] | A.A. Beilinson, V.V. Schechtman, "Determinant bundles and Virasoro algebras" Comm. Math. Phys. , 118 (1988) pp. 651–701 |
| [a18] | N. Kawamoto, Y. Namikawa, A. Tsuchiya, Y. Yamada, "Geometric realization of conformal field theory on Riemann surfaces" Comm. Math. Phys. , 116 (1988) pp. 247–308 |
Virasoro algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Virasoro_algebra&oldid=15975