Vekua method
in the theory of infinitesimal deformations
A method which can be applied to the case when certain quantities which characterize the deformation of surfaces with positive Gaussian curvature  are, in a conjugate-isothermal parametrization, generalized analytic functions (cf. Generalized analytic function). This makes it possible to reduce the study of the deformation of surfaces with variable
are, in a conjugate-isothermal parametrization, generalized analytic functions (cf. Generalized analytic function). This makes it possible to reduce the study of the deformation of surfaces with variable 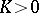 to a definite problem concerning surfaces with
to a definite problem concerning surfaces with 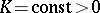 , whose infinitesimal deformations (cf. Infinitesimal deformation) are described by ordinary analytic functions, thus establishing a far-reaching analogy between the properties of deformations of surfaces with variable and constant positive Gaussian curvature.
, whose infinitesimal deformations (cf. Infinitesimal deformation) are described by ordinary analytic functions, thus establishing a far-reaching analogy between the properties of deformations of surfaces with variable and constant positive Gaussian curvature.
References
| [1] | I.N. Vekua, "Generalized analytic functions" , Pergamon (1962) (Translated from Russian) |
Vekua method. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Vekua_method&oldid=11723