Variational principles of classical mechanics
Fundamental tenets of analytical mechanics, mathematically expressed in the form of variational relations, from which the differential equations of motion and all the statements and laws of mechanics logically follow. In variational principles of classical mechanics real motions of a material system taking place under the effect of applied forces are compared with the kinematically-possible motions which are permitted by the constraints imposed on the system and which satisfy certain conditions. In most cases the criterion according to which a real motion is selected out of the class of kinematically-possible motions under consideration is the condition of extremality (stationarity) of some scalar function or functional which ensures the invariance of the description.
The variational principles of classical mechanics differ from one another both by the form and by the manners of variation, and by their generality, but each principle, within the scope of its application, forms a unique foundation of and synthesizes, as it were, the entire mechanics of the corresponding material systems. In other words, any one of the variational principles of classical mechanics potentially contains the entire subject matter of this field of science and combines all its statements in a single formulation.
Classical mechanics is based on the Newton laws of mechanics, which were established for free material points, and on constraint axioms. The validity of the variational principles of classical mechanics is based on these laws and axioms. Alternatively, any variational principle of classical mechanics may be taken as an axiom, and the laws of mechanics may be deduced from it.
In accordance with their form, one distinguishes between differential and integral variational principles. Differential principles, which describe the properties of motion for any given moment of time, comprise the principle of virtual displacements, the d'Alembert–Lagrange principle, and the principles of Gauss, Hertz, Chetaev, and Jourdain. Integral principles, which describe the properties of motion during any finite period of time, represent the principle of least action in the forms given to it by Hamilton–Ostrogradski, Lagrange, Jacobi, and others.
The first variational principle of classical mechanics is the principle of possible (virtual) displacements, which was used as early as 1665 by G. Galilei. J. Bernoulli in 1717 was the first to grasp the generality of this principle and its usefulness for the solution of problems in statics. J.L. Lagrange, in his "Mécanique analytique of LagrangeMécanique analytique" (1788), gave a justification of this principle, advanced its development and applied the principle, justly considering it to be fundamental to mechanics as a whole. The principle allows one to find an equilibrium position of a system of material points, i.e. positions 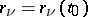 in which the system will remain for an indefinite time if it was placed there with zero initial velocities
in which the system will remain for an indefinite time if it was placed there with zero initial velocities 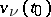 , where
, where 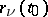 are possible positions and
are possible positions and 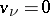 are kinematically-possible velocities at any time
are kinematically-possible velocities at any time 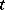 . Here,
. Here, 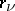 are the radius vectors of the system with respect to the origin
are the radius vectors of the system with respect to the origin 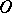 of the inertial coordinate system
of the inertial coordinate system 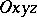 , and
, and 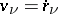 ;
; 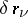 are the possible displacements permitted at the given moment of time by the constraints imposed on the system,
are the possible displacements permitted at the given moment of time by the constraints imposed on the system, 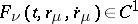 are given active forces, and
are given active forces, and 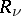 are the reactions of the constraints. Further, the constraints are assumed to be ideal (bilateral or two-sided), i.e.
are the reactions of the constraints. Further, the constraints are assumed to be ideal (bilateral or two-sided), i.e.
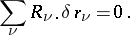 |
The principle of virtual displacements: A mechanical system is at equilibrium in a given position if and only if the sum of the elementary (infinitely small) work elements performed by the active forces on all possible displacements which would take the system out of this position is zero,
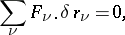 | (1) |
at any moment of time.
Equation (1) is the general equation of statics which reduces any problem in statics to a mathematical study of this equation. For the special case of potential forces
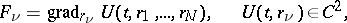 |
where 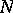 is the number of points of the system, equation (1) assumes the form
is the number of points of the system, equation (1) assumes the form
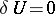 | (2) |
for an arbitrary  , i.e. a mechanical system subject to the action of potential forces is at equilibrium if and only if the force function has a stationary value.
, i.e. a mechanical system subject to the action of potential forces is at equilibrium if and only if the force function has a stationary value.
The equations of dynamics may be deduced by the methods of statics with the aid of the so-called d'Alembert principle: If the inertial forces 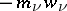 are added to the given active forces acting on the system and to the reaction forces of the constraints, such a system will be at equilibrium. Here,
are added to the given active forces acting on the system and to the reaction forces of the constraints, such a system will be at equilibrium. Here, 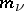 is the mass of the
is the mass of the  -th point and
-th point and 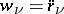 is its acceleration. Lagrange in 1788 generalized d'Alembert's principle and the principle of virtual displacements.
is its acceleration. Lagrange in 1788 generalized d'Alembert's principle and the principle of virtual displacements.
The d'Alembert–Lagrange principle: For the real motion of the system, the sum of the work elements of the active forces and the inertial forces on all possible displacements is zero,
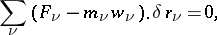 | (3) |
at any moment of time.
The d'Alembert–Lagrange principle compares the positions of the system during its actual motion with the infinitely close positions permitted by the constraints at the moment of time considered. Relation (3) defines the dependence between the active forces, the accelerations of points produced by these forces subject to the imposed constraints, and the possible displacements. Expressing as it does a necessary and sufficient condition for the correspondence of the actual motion, which is one of the kinematically-possible motions determined by the active forces, equation (3) is the general equation of dynamics. If all the accelerations 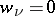 , equation (3) assumes the form of the general equation of statics (1).
, equation (3) assumes the form of the general equation of statics (1).
The equations of motion are contained in equation (3). To obtain the complete system of independent differential equations of dynamics, it is sufficient to express the possible displacements  in terms of a system of independent displacements and to substitute them in equation (3). Lagrange's equations, Appell's equations and any other system of independent differential equations of motion can be obtained in this way. If, on the other hand, some displacement is selected out of the family of possible displacements and is substituted into equation (3), the relation thus obtained will either be one of the differential equations of motion of the system or will follow from them. For instance, the general theorems (laws) of dynamics — the theorems on momentum, moment of momentum and kinetic energy — may be obtained in this way.
in terms of a system of independent displacements and to substitute them in equation (3). Lagrange's equations, Appell's equations and any other system of independent differential equations of motion can be obtained in this way. If, on the other hand, some displacement is selected out of the family of possible displacements and is substituted into equation (3), the relation thus obtained will either be one of the differential equations of motion of the system or will follow from them. For instance, the general theorems (laws) of dynamics — the theorems on momentum, moment of momentum and kinetic energy — may be obtained in this way.
The general theorems of dynamics of the system describe certain properties of motion, but unlike the variational principles of classical dynamics, none of them is in a position (at the time of writing, 1991) to replace any system of differential equations of motion or to fully describe the motion of the system.
The d'Alembert–Lagrange principle is one of the most general variational principles of classical mechanics which is valid for both holonomic and non-holonomic systems. All other variational principles constitute either this principle in a different formulation or one of its corollaries. However, the d'Alembert–Lagrange principle is not connected with the concept of the extremum of any function. It involves an infinitely small magnitude — the sum of the work elements of the given forces and of the inertial forces on an infinitely small displacement from the given configuration, which does not represent the variation of any function similarly to equation (2).
C.F. Gauss in 1829 proposed a new variational principle, which is a modification of the d'Alembert–Lagrange principle. Out of all the kinematically-possible displacements, Gauss considers those satisfying the conditions imposed on the system by the constraints, and subject to the requirement that, at the moment of time 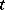 being considered,
being considered,  and
and  are constant. At the moment
are constant. At the moment 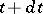 :
:
 |
where  and
and 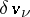 denote the changes in the velocity of motion during the period of time
denote the changes in the velocity of motion during the period of time 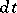 for the actual and for some virtual displacement; here, (3) is written as
for the actual and for some virtual displacement; here, (3) is written as
 | (4) |
where 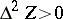 .
.
Gauss' principle of least compulsion (Gauss' principle): The motion of a system of material points which are interconnected in some way and are subject to arbitrary influences at any moment of time takes place in the best possible agreement with the motion which would be executed by these points if they were free, i.e. the compulsion on the motion is the least possible if one accepts as measure of the compulsion exerted during time  the magnitude
the magnitude 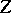 , equal to the sum of the products of the mass of each point by the square of its deviation from the point it would have occupied if it had been free. In other words, at any moment of time
, equal to the sum of the products of the mass of each point by the square of its deviation from the point it would have occupied if it had been free. In other words, at any moment of time  , out of all the accelerations caused by the forces, the actual accelerations
, out of all the accelerations caused by the forces, the actual accelerations  of the various points of the system will be those for which the function
of the various points of the system will be those for which the function 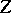 becomes minimal to the second degree with respect to the acceleration.
becomes minimal to the second degree with respect to the acceleration.
Equilibrium is a special case of the general law: It is obtained if the points have velocity zero, and if the preservation of the system at rest is closer to free motion in the absence of the constraints than to the possible motions permitted in the presence of the constraints.
If 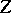 is expressed in terms of independent accelerations of the system, Appell's equations are obtained from Gauss' principle. Gauss' principle is the physical analogue of the method of least squares (cf. Least squares, method of) in the theory of errors. Gauss' principle is equivalent to the d'Alembert–Lagrange principle, but a study of non-linear differential constraints of the type
is expressed in terms of independent accelerations of the system, Appell's equations are obtained from Gauss' principle. Gauss' principle is the physical analogue of the method of least squares (cf. Least squares, method of) in the theory of errors. Gauss' principle is equivalent to the d'Alembert–Lagrange principle, but a study of non-linear differential constraints of the type 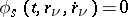 proved these principles to be incompatible, according to P. Appell and E. Delassus (1911–1913). This problem was solved by N.G. Chetaev (1932–1933), who proposed that the possible displacements of non-linear constraints be defined by conditions of the type
proved these principles to be incompatible, according to P. Appell and E. Delassus (1911–1913). This problem was solved by N.G. Chetaev (1932–1933), who proposed that the possible displacements of non-linear constraints be defined by conditions of the type
 |
The principle of least reactions, which is a consequence of Gauss' principle, states that for an actual motion the quantity
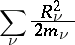 |
is minimal. Gauss' principle has been generalized to the case when some of the constraints are eliminated from the system. Since the possible displacements of the initial system are comprised among the possible displacements of the free system, the relation
 |
where 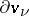 is the change in the velocity of motion during the time
is the change in the velocity of motion during the time  in the free system, will be valid. In view of equation (4), the above equation may be reduced to
in the free system, will be valid. In view of equation (4), the above equation may be reduced to
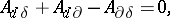 | (5) |
where
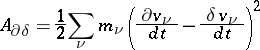 |
describes the measure of the deviation of the motion 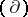 from the motion
from the motion  during the time
during the time  . The quantities
. The quantities 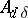 and
and 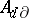 are written in a similar manner. A corollary of equation (5) are the inequalities
are written in a similar manner. A corollary of equation (5) are the inequalities
 | (6) |
which express the following theorem: The deviation of the actual motion 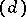 of the system from the imagined motion
of the system from the imagined motion 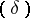 (i.e. from that of the free actual
(i.e. from that of the free actual 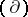 ) is smaller than the deviation between the motion
) is smaller than the deviation between the motion 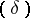 and the motion
and the motion  . This theorem was proved by Chetaev in 1932–1933. The theorem which is expressed by the second inequality in (6) was postulated by E. Mach in 1883 for the case of linear non-holonomic constraints, and was proved in 1916 by E.A. Bolotov.
. This theorem was proved by Chetaev in 1932–1933. The theorem which is expressed by the second inequality in (6) was postulated by E. Mach in 1883 for the case of linear non-holonomic constraints, and was proved in 1916 by E.A. Bolotov.
A principle which is closely related to Gauss' principle is the principle of the most direct path, postulated by H. Hertz in 1894 as the fundamental law of the mechanics he had developed. In it, unlike in the mechanics of Newton, the concept of a force is replaced by concepts of latent constraints, latent masses and latent motions.
The principle of the most direct path (the principle of least curvature, Hertz' principle): Any free system remains at rest or in a state of uniform motion along the most direct path. A "free" system according to Hertz is one not acted upon by active forces and restricted only by internal constraints which impose certain conditions on the mutual positions of the points constituting the system. The most direct part is defined as the trajectory consisting of elementary arcs with the smallest curvature as compared with any other arcs and permitted constraints, and having a common initial point and a common tangent,  being interpreted as the curvature of the trajectory of a point representing the position of the system in
being interpreted as the curvature of the trajectory of a point representing the position of the system in 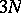 -dimensional Euclidean space with rectangular coordinates
-dimensional Euclidean space with rectangular coordinates 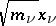 ,
, 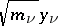 ,
, 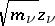 . In other words, Hertz' principle states that, out of all the trajectories compatible with the constraints, the actual trajectory has least curvature.
. In other words, Hertz' principle states that, out of all the trajectories compatible with the constraints, the actual trajectory has least curvature.
Hertz' principle is equivalent to Gauss' principle for systems confined by stationary constraints and not subjected to active forces. Chetaev (1941) proposed the following modification of Gauss' principle.
Chetaev's principle of maximum work: The work
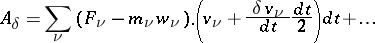 |
on an elementary cycle consisting of the direct motion in the field of given forces and of the inverse motion in the field of forces which would suffice to produce the actual motion if the mechanical system were completely free, has a (relative) maximum in the class of motions imaginable according to Gauss for the actual motion. Chetaev's principle makes it possible to extend the character of the mechanical systems ordinarily considered by using the Carnot principle in thermodynamics.
The principles outlined above may be subdivided into two groups, in accordance with their manner of variation; in the principle of virtual displacements and in the d'Alembert–Lagrange principle the variable quantity is the state 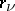 of the system at a given moment of time, while in the principles of Gauss, Hertz and Chetaev the variable quantity is the acceleration
of the system at a given moment of time, while in the principles of Gauss, Hertz and Chetaev the variable quantity is the acceleration 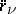 of the system for constant
of the system for constant  and
and 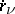 . Jourdain's principle, in which the velocities
. Jourdain's principle, in which the velocities  are varied for the moment
are varied for the moment  at constant
at constant 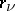 , occupies an intermediate place between the two. At the moment
, occupies an intermediate place between the two. At the moment 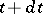 the possible displacements are
the possible displacements are 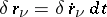 , and equation (3) assumes the form
, and equation (3) assumes the form
 |
In the integral variational principles of classical mechanics the comparison between the actual motion and the kinematically-possible motions is effected over finite intervals of time. In fact, the values of certain given integrals (the so-called actions), which can be calculated for the actual and for the kinematically-possible motions, subject to certain conditions, are compared for two states of the system. The integral variational principles of classical mechanics are less general than the differential ones and are applicable mainly to holonomic systems acted upon by potential forces. The most general such principle was established in 1834–1835 by W. Hamilton for the case of stationary holonomic constraints, and was generalized by M.V. Ostrogradski (1848) to non-stationary geometric constraints. Consider two known positions 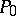 and
and  of a holonomic system at moments of time
of a holonomic system at moments of time 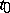 and
and 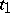 , respectively, during a motion of the system under the effect of given forces and reactions. In this motion the
, respectively, during a motion of the system under the effect of given forces and reactions. In this motion the 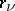 will be functions of time which satisfy the constraints and, for
will be functions of time which satisfy the constraints and, for  and
and 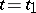 , assume values corresponding to the states
, assume values corresponding to the states 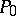 and
and 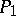 , respectively. Let
, respectively. Let 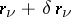 be certain functions of time of class
be certain functions of time of class  , sufficiently close to
, sufficiently close to 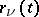 , satisfying the constraints, and assuming the same values as
, satisfying the constraints, and assuming the same values as 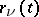 for
for 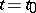 and
and 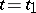 . Then the functions
. Then the functions 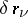 vanish for
vanish for 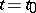 and
and 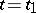 and have the meaning of possible displacements. If
and have the meaning of possible displacements. If 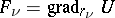 , then
, then
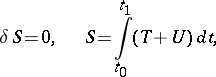 | (7) |
where the functional 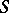 is known as the Hamilton action for the interval
is known as the Hamilton action for the interval 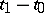 .
.
The principle of stationary action (the Hamilton–Ostrogradski principle): For an actual motion of the system, the Hamilton action has a stationary value in comparison with any infinitely close kinematically-possible motion for which the initial and final states of the system are the same as the respective states for the actual motion and the durations of motion are equal. If the forces are non-potential, the Hamilton–Ostrogradski principle is expressed by the equation
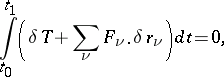 | (8) |
i.e. if 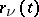 are functions of time corresponding to the actual motion of the system, the integral (8) is zero for all variations of the functions
are functions of time corresponding to the actual motion of the system, the integral (8) is zero for all variations of the functions 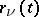 which are compatible with the constraints and vanish at both limits of the integral.
which are compatible with the constraints and vanish at both limits of the integral.
Equations (7) and (8) are necessary and sufficient conditions for the motion of the system acted upon by given forces to be actual. Equation (8) is also valid for non-holonomic systems, but for such systems the motion 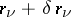 is, in general, kinematically impossible; equation (7) does not apply to non-holonomic systems.
is, in general, kinematically impossible; equation (7) does not apply to non-holonomic systems.
If the equations of the geometrical constraints are represented by
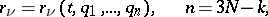 |
where 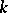 is the number of constraints, and if the Lagrange function
is the number of constraints, and if the Lagrange function
 |
is introduced, then
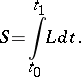 |
In the extended 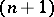 -dimensional coordinate space
-dimensional coordinate space 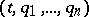 , equation (7) corresponds to the ordinary (non-parametric) problem of variational calculus with fixed ends. In the
, equation (7) corresponds to the ordinary (non-parametric) problem of variational calculus with fixed ends. In the  -dimensional extended phase space with coordinates
-dimensional extended phase space with coordinates  , equation (7) corresponds to the variational problem with free ends (
, equation (7) corresponds to the variational problem with free ends (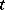 and
and 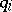 are fixed;
are fixed; 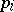 are free), and
are free), and
 |
where  is the Hamiltonian.
is the Hamiltonian.
Equation (7) yields the Lagrange equations:
 | (9) |
and the canonical Hamilton equations:
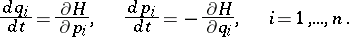 | (10) |
In order to solve the fundamental problems in dynamics it is sufficient to know the action function
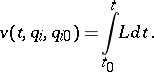 | (11) |
However, the law of motion must be known to find the action function from formula (11). In order to obviate this difficulty, Hamilton arrived at the differential equation
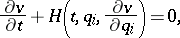 | (12) |
which is satisfied by the action function. C.G.J. Jacobi (1837) showed that if the complete integral 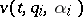 of equation (12) is known (this integral depends on
of equation (12) is known (this integral depends on  arbitrary constants
arbitrary constants 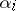 , none of which is additive), the general solution of equation (10) is given by
, none of which is additive), the general solution of equation (10) is given by
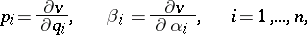 |
where 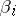 are arbitrary constants.
are arbitrary constants.
For systems constrained by stationary constraints and acted upon by potential forces which do not explicitly depend on time, there exists the energy integral
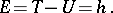 | (13) |
The existence of this integral makes it possible to restrict the set of comparable kinematically-possible motions which convert the system from state 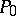 to state
to state 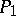 to the motions for which the complete mechanical energy
to the motions for which the complete mechanical energy 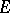 has a fixed value
has a fixed value 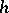 . The integral (13) may then be considered as a non-holonomic condition and the principle of mechanics may be sought in the form of a conditional variational principle. This problem was solved by Lagrange in 1760.
. The integral (13) may then be considered as a non-holonomic condition and the principle of mechanics may be sought in the form of a conditional variational principle. This problem was solved by Lagrange in 1760.
Lagrange's principle of stationary action: Given the initial moment of time  and the initial and final positions of a holonomic system for which the energy integral exists, the equation
and the initial and final positions of a holonomic system for which the energy integral exists, the equation
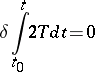 | (14) |
is valid for the actual motion in comparison with the various motions between the same initial and final states and with the same energy  as in the actual motion. The symbol
as in the actual motion. The symbol 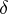 denotes the variation subject to condition (13).
denotes the variation subject to condition (13).
If relation (13) is satisfied for a constant 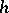 for all motions which are comparable according to Lagrange's principle, this fact imposes certain restrictions on the rates of these motions, and the time of the displacement from
for all motions which are comparable according to Lagrange's principle, this fact imposes certain restrictions on the rates of these motions, and the time of the displacement from  to
to 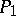 depends on the curve along which the motion is performed. Thus, the Lagrange principle (14) (taking into account (13)) is a conditional variational problem with a free upper end.
depends on the curve along which the motion is performed. Thus, the Lagrange principle (14) (taking into account (13)) is a conditional variational problem with a free upper end.
If, in these systems, the time 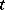 is eliminated from (14) with the aid of the energy integral (13), a new variational principle will follow; this principle was obtained in 1837 by Jacobi.
is eliminated from (14) with the aid of the energy integral (13), a new variational principle will follow; this principle was obtained in 1837 by Jacobi.
The kinetic energy of a system may be expressed in generalized coordinates 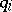 as follows:
as follows:
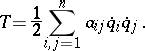 |
The metric of the coordinate space is given by the formula
 | (15) |
The initial and final positions 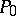 and
and 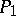 of the system in some actual motion are also given.
of the system in some actual motion are also given.
Jacobi's principle of stationary action: If the initial and final positions of a holonomic conservative system are given, then the following equation is valid for the actual motion:
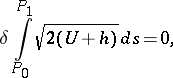 | (16) |
as compared to all other infinitely near motions between identical initial and final positions and for the same constant value of the energy 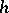 as in the actual motion.
as in the actual motion.
Jacobi's principle reduces the study of the motion of a holonomic conservative system to the geometric problem of finding the extremals of the variational problem (16) in a Riemannian space with the metric (15) which represents the real trajectories of the system. Jacobi's principle reveals the close connection between the motions of a holonomic conservative system and the geometry of Riemannian spaces. If the motion of the system takes place in the absence of applied forces, i.e. 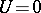 , the system moves along a geodesic line of the coordinate space
, the system moves along a geodesic line of the coordinate space  at a constant rate. This fact is a generalization of Galilei's law of inertia. If
at a constant rate. This fact is a generalization of Galilei's law of inertia. If  , determining the motion of a holonomic conservative system is also reduced to the task of determining the geodesics in a Riemannian space with the metric
, determining the motion of a holonomic conservative system is also reduced to the task of determining the geodesics in a Riemannian space with the metric
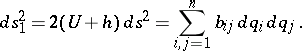 |
In the case of a single material point, when the line element 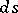 is the element of three-dimensional Euclidean space, Jacobi's principle is the mechanical analogue of Fermat's principle in optics.
is the element of three-dimensional Euclidean space, Jacobi's principle is the mechanical analogue of Fermat's principle in optics.
The Lagrange equations (9) or the equations of the extremals of the variational principles of Hamilton–Ostrogradski, Lagrange and Jacobi are necessary conditions for the extremum of the respective integral or of the action according to Hamilton, Lagrange and Jacobi. If the sufficient conditions for a minimum are met, the integrals assume their minimal values in actual motions. As a result, the integral variational principles of classical mechanics are also referred to as principles of least action.
The use of integral variational principles of classical mechanics naturally leads to the concept of generalized solutions and extended classes of function spaces in which solutions of problems of mathematical physics are to be found.
Variational principles of classical mechanics proved to be applicable not only to discrete material systems, but also to systems with distributed parameters and to continuous media; they play an important part in field theory and in mathematical physics. The optical-mechanical analogy, the theory of canonical transformations, the theory of Lie groups, and the conservation laws are closely connected with the variational principles of classical mechanics. These principles are of high heuristic value, and are also applicable to other branches of physics, in particular to the theory of relativity and to quantum mechanics and wave mechanics, in which the principle of least action and the Lagrange and Hamilton formalisms play an important part.
References
| [1] | P. Appell, "Traité de mécanique rationelle" , Gauthier-Villars (1953) |
| [2] | E.A. Bolotov, "On Gauss' principle" Izv. Fiz.-Mat. Obshch. Kazan. Univ. (2) , 21 : 3 (1916) pp. 99–152 (In Russian) |
| [3] | , Variational principles of mechanics , Moscow (1959) (In Russian) |
| [4] | C. Lanczos, "The variational principles of mechanics" , Univ. Toronto Press (1962) |
| [5] | L.A. Pars, "A treatise on analytical dynamics" , Heinemann , London (1965) |
| [6] | N.V. Roze, "Lectures on analytical mechanics" , 1 , Leningrad (1938) (In Russian) |
| [7] | N.G. Chetaev, "Stability of motion" , Works on analytical mechanics , 6 , Moscow (1962) (In Russian) |
Comments
References
| [a1] | V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) |
| [a2] | E.T. Whittaker, "Analytical dynamics of particles and rigid bodies" , Dover, reprint (1944) |
| [a3] | H. Goldstein, "Classical mechanics" , Addison-Wesley (1950) |
| [a4] | F.R. [F.R. Gantmakher] Gantmacher, "Lectures in analytical mechanics" , MIR (1975) (Translated from Russian) |
Variational principles of classical mechanics. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Variational_principles_of_classical_mechanics&oldid=17338