Difference between revisions of "Two-dimensional manifold of bounded curvature"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 30: | Line 30: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> A.D. Aleksandrov, V.A. Zalgaller, "Two-dimensional surfaces of bounded curvature" , Moscow-Leningrad (1962) (In Russian)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A.D. Aleksandrov, V.A. Zalgaller, "Two-dimensional surfaces of bounded curvature Part 2" ''Proc. Steklov Inst. Math.'' , '''76''' (1967) ''Trudy Mat. Inst. Steklov.'' , '''76''' (1965)</TD></TR></table> |
| Line 38: | Line 38: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> A.D. Aleksandrov, V.A. Zalgaller, "Intrinsic geometry of surfaces" , ''Transl. Math. Monogr.'' , Amer. Math. Soc. (1967) (Translated from Russian) {{MR|0216434}} {{ZBL|0146.44103}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> W. Rinow, "Die innere Geometrie der metrischen Räume" , Springer (1961) {{MR|0123969}} {{ZBL|0096.16302}} </TD></TR></table> |
Revision as of 17:02, 15 April 2012
A metric space which is a two-dimensional manifold with a metric, for which analogues of the concepts of two-dimensional Riemannian geometry such as the length and the total curvature of a curve, the area and the total Gaussian curvature of a set have been defined.
Special cases of two-dimensional manifolds of bounded curvature are two-dimensional Riemannian spaces and polyhedral surfaces in three-dimensional Euclidean space. In the general case the class of two-dimensional manifolds of bounded curvature may be regarded as the closure of the class of two-dimensional Riemannian manifolds with respect to an appropriate limit process.
Let 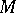 be a two-dimensional Riemannian manifold, let
be a two-dimensional Riemannian manifold, let 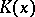 be the Gaussian curvature of
be the Gaussian curvature of  at a point
at a point  and let
and let 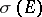 be the area of a set
be the area of a set 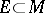 ; then the total curvature of
; then the total curvature of  will be
will be
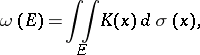 |
its total absolute curvature will be
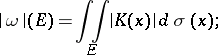 |
and the positive part of the total curvature of 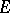 will be
will be
 |
where 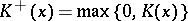 . If
. If  and
and 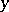 are two points in a Riemannian space
are two points in a Riemannian space 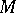 , let
, let 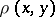 be the lower bound of the lengths of the curves on
be the lower bound of the lengths of the curves on 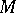 which connect the points
which connect the points  and
and 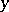 . The function
. The function 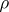 is an internal metric; it is known as the natural metric of
is an internal metric; it is known as the natural metric of 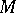 .
.
Let 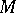 be an arbitrary two-dimensional manifold with metric
be an arbitrary two-dimensional manifold with metric 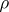 . One says that the metric
. One says that the metric 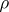 is Riemannian if the manifold
is Riemannian if the manifold 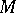 with the metric
with the metric  is isometric to some two-dimensional Riemannian space with its natural metric.
is isometric to some two-dimensional Riemannian space with its natural metric.
A two-dimensional manifold 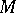 with a metric
with a metric 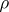 is a two-dimensional manifold of bounded curvature if the following condition is met: There exists a sequence of Riemannian metrics
is a two-dimensional manifold of bounded curvature if the following condition is met: There exists a sequence of Riemannian metrics 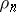 ,
, 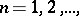 defined on
defined on 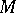 , such that for any compact set
, such that for any compact set 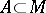 one has
one has 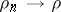 uniformly (i.e. the functions
uniformly (i.e. the functions 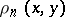 uniformly converge to the function
uniformly converge to the function 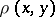 on the set
on the set 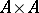 ) and the sequence
) and the sequence 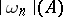 ,
, 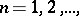 is bounded; here,
is bounded; here, 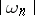 is the total absolute curvature of the Riemannian metric
is the total absolute curvature of the Riemannian metric 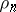 . Two-dimensional manifolds of bounded curvature can be defined axiomatically.
. Two-dimensional manifolds of bounded curvature can be defined axiomatically.
The sufficient conditions in the definition of a two-dimensional manifold of bounded curvature given above may be partially weakened. Namely, a two-dimensional manifold  with a metric
with a metric 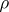 will be a two-dimensional manifold of bounded curvature if for any of its points it is possible to determine neighbourhoods
will be a two-dimensional manifold of bounded curvature if for any of its points it is possible to determine neighbourhoods 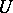 and
and  , where
, where 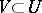 , and a sequence of Riemannian metrics
, and a sequence of Riemannian metrics  ,
, 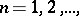 defined on
defined on  , such that
, such that 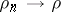 uniformly on
uniformly on 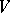 and the sequence
and the sequence  is bounded.
is bounded.
For any two-dimensional manifold of bounded curvature there are defined totally-additive set functions 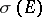 and
and 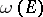 , viz., the area and the curvature of a set, respectively. In contrast to the Riemannian case,
, viz., the area and the curvature of a set, respectively. In contrast to the Riemannian case, 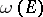 need not be absolutely continuous with respect to
need not be absolutely continuous with respect to  . For two-dimensional manifolds of bounded curvature the concept of the rotation of a curve is also defined; it is the analogue of the concept of the total geodesic curvature of a curve.
. For two-dimensional manifolds of bounded curvature the concept of the rotation of a curve is also defined; it is the analogue of the concept of the total geodesic curvature of a curve.
Any convex surface in three-dimensional Euclidean space is a two-dimensional manifold of bounded curvature. In such a case the total curvature of a set is always non-negative.
Two-dimensional manifolds of bounded curvature can have singularities like conical points 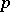 (for such points
(for such points 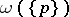 is non-zero), edges, borders with a cylindrical base, etc.
is non-zero), edges, borders with a cylindrical base, etc.
References
| [1] | A.D. Aleksandrov, V.A. Zalgaller, "Two-dimensional surfaces of bounded curvature" , Moscow-Leningrad (1962) (In Russian) |
| [2] | A.D. Aleksandrov, V.A. Zalgaller, "Two-dimensional surfaces of bounded curvature Part 2" Proc. Steklov Inst. Math. , 76 (1967) Trudy Mat. Inst. Steklov. , 76 (1965) |
Comments
Instead of natural metric the terminology induced metric and intrinsic metric is also used.
References
| [a1] | A.D. Aleksandrov, V.A. Zalgaller, "Intrinsic geometry of surfaces" , Transl. Math. Monogr. , Amer. Math. Soc. (1967) (Translated from Russian) MR0216434 Zbl 0146.44103 |
| [a2] | W. Rinow, "Die innere Geometrie der metrischen Räume" , Springer (1961) MR0123969 Zbl 0096.16302 |
Two-dimensional manifold of bounded curvature. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Two-dimensional_manifold_of_bounded_curvature&oldid=14396