Triangulation
A triangulation of a polyhedron, or rectilinear triangulation, is a representation of the polyhedron (cf. Polyhedron, abstract) as the space of a geometric simplicial complex  , that is, a decomposition of it into closed simplices such that any two simplices either do not intersect or intersect along a common face. Rectilinear triangulations of polyhedra serve as the main tool for studying them. Any polyhedron has a triangulation and any two triangulations of it have a common subdivision.
, that is, a decomposition of it into closed simplices such that any two simplices either do not intersect or intersect along a common face. Rectilinear triangulations of polyhedra serve as the main tool for studying them. Any polyhedron has a triangulation and any two triangulations of it have a common subdivision.
The closed star 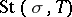 of a simplex
of a simplex 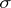 in a triangulation
in a triangulation 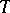 is the union of the simplices of
is the union of the simplices of 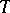 containing
containing 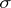 . There is a representation of the closed star of a simplex
. There is a representation of the closed star of a simplex 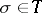 as the union (or join, cf. Union of sets) of
as the union (or join, cf. Union of sets) of 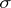 and its link:
and its link:  . In particular, the star of a vertex is a cone over its link. If a simplex
. In particular, the star of a vertex is a cone over its link. If a simplex 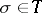 is represented as the join of two of its faces
is represented as the join of two of its faces 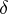 and
and 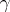 , then
, then 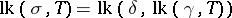 . The link of a simplex does not depend on
. The link of a simplex does not depend on 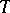 : If
: If 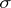 is a simplex in rectilinear triangulations
is a simplex in rectilinear triangulations 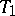 ,
, 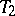 of the same polyhedron, then the polyhedra
of the same polyhedron, then the polyhedra 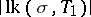 and
and 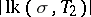 are PL-homeomorphic. The open star of a simplex
are PL-homeomorphic. The open star of a simplex  is defined as the union of the interiors of those simplices of
is defined as the union of the interiors of those simplices of 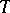 containing
containing 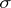 as a face. The open stars of the vertices of a triangulation of a polyhedron
as a face. The open stars of the vertices of a triangulation of a polyhedron 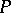 form an open covering of
form an open covering of 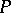 . The nerve of this covering (cf. Nerve of a family of sets) is simplicially isomorphic to the triangulation. Two triangulations
. The nerve of this covering (cf. Nerve of a family of sets) is simplicially isomorphic to the triangulation. Two triangulations  and
and 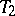 of polyhedra
of polyhedra 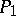 and
and 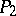 are combinatorially equivalent if certain subdivisions of them are simplicially isomorphic. In order that two triangulations
are combinatorially equivalent if certain subdivisions of them are simplicially isomorphic. In order that two triangulations 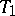 and
and 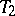 be combinatorially equivalent it is necessary and sufficient that
be combinatorially equivalent it is necessary and sufficient that  and
and 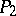 be PL-homeomorphic. A triangulation of a manifold is said to be combinatorial if the star of any of its vertices is combinatorially equivalent to a simplex. In this case the star of any simplex of the triangulation is also combinatorially equivalent to a simplex.
be PL-homeomorphic. A triangulation of a manifold is said to be combinatorial if the star of any of its vertices is combinatorially equivalent to a simplex. In this case the star of any simplex of the triangulation is also combinatorially equivalent to a simplex.
If 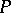 is a closed subpolyhedron of a polyhedron
is a closed subpolyhedron of a polyhedron  , then any triangulation
, then any triangulation 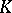 of
of 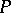 can be extended to some triangulation
can be extended to some triangulation 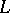 of
of 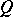 . In this case one says that the pair of geometric simplicial complexes
. In this case one says that the pair of geometric simplicial complexes 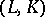 triangulates the pair
triangulates the pair 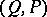 . A triangulation of the direct product
. A triangulation of the direct product 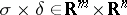 of two simplices
of two simplices 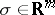 ,
,  can be constructed as follows. The vertices of the triangulation are the points
can be constructed as follows. The vertices of the triangulation are the points 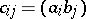 ,
, 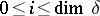 , where
, where 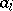 are the vertices of
are the vertices of 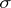 and
and  are the vertices of
are the vertices of 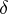 . The vertices
. The vertices 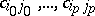 , where
, where 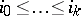 , span a
, span a 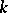 -dimensional simplex if and only if none of these coincide and
-dimensional simplex if and only if none of these coincide and 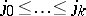 . A triangulation of the direct product of two simplicial complexes with ordered vertices can be carried out in the same way.
. A triangulation of the direct product of two simplicial complexes with ordered vertices can be carried out in the same way.
A triangulation of a topological space, or curvilinear triangulation, is a pair  , where
, where 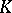 is a geometric simplicial complex and
is a geometric simplicial complex and 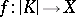 is a homeomorphism. Two triangulations
is a homeomorphism. Two triangulations 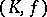 and
and 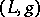 of a space
of a space 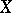 coincide if
coincide if 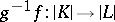 is a simplicial isomorphism. If
is a simplicial isomorphism. If 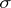 is a simplex of a complex
is a simplex of a complex 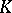 and
and 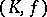 is a triangulation of
is a triangulation of 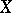 , then the space
, then the space 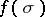 endowed with the homeomorphism
endowed with the homeomorphism 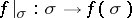 is called a topological simplex. The star and the link of a topological simplex of a triangulated topological space are defined in the same way as in the case of rectilinear triangulations. If a point
is called a topological simplex. The star and the link of a topological simplex of a triangulated topological space are defined in the same way as in the case of rectilinear triangulations. If a point 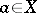 is a vertex of triangulations
is a vertex of triangulations 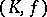 and
and 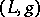 of
of  , then its links in these triangulations are homotopy equivalent.
, then its links in these triangulations are homotopy equivalent.
References
| [1] | P.S. Aleksandrov, "Combinatorial topology" , Graylock , Rochester (1956) (Translated from Russian) |
| [2] | D.B. Fuks, V.A. Rokhlin, "Beginner's course in topology. Geometric chapters" , Springer (1981) (Translated from Russian) |
Comments
References
| [a1] | E.C. Zeeman, "Seminar on combinatorial topology" , IHES (1963) |
| [a2] | H. Seifert, W. Threlfall, "Lehrbuch der Topologie" , Chelsea, reprint (1970) |
| [a3] | I.M. Singer, J.A. Thorpe, "Lecture notes on elementary topology and geometry" , Springer (1967) |
| [a4] | L.C. Glaser, "Geometrical combinatorial topology" , 1–2 , v. Nostrand (1970) |
Triangulation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Triangulation&oldid=17514