Difference between revisions of "Tight and taut immersions"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 19: | Line 19: | ||
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281062.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a3)</td></tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281062.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a3)</td></tr></table> | ||
| − | Here <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281063.png" /> is a unit vector, the gradient of the linear function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281064.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281065.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281066.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281067.png" /> is a | + | Here <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281063.png" /> is a unit vector, the gradient of the linear function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281064.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281065.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281066.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281067.png" /> is a "height function on a manifoldheight function" on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281068.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281069.png" /> is the number of non-degenerate critical points of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281070.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281071.png" /> the expectation (or mean) value for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281072.png" /> with respect to the standard invariant measure on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281073.png" />. |
For smooth immersions one has | For smooth immersions one has | ||
| Line 47: | Line 47: | ||
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281089.png" /></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281089.png" /></td> </tr></table> | ||
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281090.png" /> is a tight immersion and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281091.png" /> is a non-degenerate height function, then by Morse theory <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281092.png" /> is injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281093.png" />-homology. By continuity this injectivity then holds for every half-space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281094.png" />. For smooth immersions of closed manifolds this half-space property is equivalent to tightness. However, this half-space definition can be applied in the larger context of continuous immersions or even mappings of manifolds and other compact topological spaces into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281095.png" />. An example is the tight | + | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281090.png" /> is a tight immersion and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281091.png" /> is a non-degenerate height function, then by Morse theory <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281092.png" /> is injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281093.png" />-homology. By continuity this injectivity then holds for every half-space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281094.png" />. For smooth immersions of closed manifolds this half-space property is equivalent to tightness. However, this half-space definition can be applied in the larger context of continuous immersions or even mappings of manifolds and other compact topological spaces into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281095.png" />. An example is the tight "Swiss cheese" , an imbedded surface with boundary, see Fig. e. A tight mapping into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t09281096.png" /> is also called a perfect function. |
<img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/t092810e.gif" /> | <img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/t092810e.gif" /> | ||
| Line 79: | Line 79: | ||
In this context there is another remarkable theorem. A substantial tight continuous immersion of the real projective plane into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810143.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810144.png" />, is necessarily an imbedding into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810145.png" /> onto either the algebraic Veronese surface or onto Banchoff's six-vertex polyhedral surface [[#References|[a11]]]. Every smooth immersion of a surface with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810146.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810147.png" /> into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810148.png" /> is regularly isotopic to a tight immersion (U. Pinkall, [[#References|[a15]]]). For the other surfaces the results are not yet complete. Every orientable surface with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810149.png" /> has a smooth substantial tight imbedding in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810150.png" />, but it | In this context there is another remarkable theorem. A substantial tight continuous immersion of the real projective plane into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810143.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810144.png" />, is necessarily an imbedding into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810145.png" /> onto either the algebraic Veronese surface or onto Banchoff's six-vertex polyhedral surface [[#References|[a11]]]. Every smooth immersion of a surface with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810146.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810147.png" /> into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810148.png" /> is regularly isotopic to a tight immersion (U. Pinkall, [[#References|[a15]]]). For the other surfaces the results are not yet complete. Every orientable surface with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810149.png" /> has a smooth substantial tight imbedding in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810150.png" />, but it | ||
| − | be analytic except for the torus (G. Thorbergsson, [[#References|[a19]]]). Every smooth imbedded knotted orientable surface in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810151.png" /> has total absolute curvature <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810152.png" />, and equality cannot be attained if genus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810153.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810154.png" />. For genus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810155.png" />, however, Kuiper and W.F. Meeks [[#References|[a10]]] proved that there do exist | + | be analytic except for the torus (G. Thorbergsson, [[#References|[a19]]]). Every smooth imbedded knotted orientable surface in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810151.png" /> has total absolute curvature <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810152.png" />, and equality cannot be attained if genus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810153.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810154.png" />. For genus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810155.png" />, however, Kuiper and W.F. Meeks [[#References|[a10]]] proved that there do exist "isotopy-tight" knotted surfaces with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810156.png" />. An example of a knotted surface of genus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810157.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810158.png" /> is depicted in Fig. e. It is obtained from two linked tight tori by two connecting handles of non-positive Gaussian curvature <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810159.png" />. For this surface every non-degenerate height function has <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810160.png" /> critical points. |
Smooth immersions of surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810161.png" /> form a subclass of the smooth stable mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810162.png" />. In that class every surface has a tight stable mapping into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810163.png" />, hence with total absolute curvature equal to | Smooth immersions of surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810161.png" /> form a subclass of the smooth stable mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810162.png" />. In that class every surface has a tight stable mapping into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810163.png" />, hence with total absolute curvature equal to | ||
| Line 87: | Line 87: | ||
Tight analytic surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810165.png" /> are isometrically rigid in the class of analytic surfaces (A.D. Aleksandrov, 1938; see [[#References|[a3]]], p. 81, and [[Rigidity|Rigidity]]). Hardly anything more is known about <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810166.png" />-rigidity of non-convex smooth closed surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810167.png" />. However, by Kuiper's theorem (1955), no smooth closed surface in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810168.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810169.png" />-isometrically rigid. A surprising tight four-dimensional manifold in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810170.png" /> is Kühnel's topological imbedding of the complex projective plane <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810171.png" /> into the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810172.png" />-skeleton of a simplex in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810173.png" /> [[#References|[a9]]]. The image is a triangulation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810174.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810175.png" /> vertices. | Tight analytic surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810165.png" /> are isometrically rigid in the class of analytic surfaces (A.D. Aleksandrov, 1938; see [[#References|[a3]]], p. 81, and [[Rigidity|Rigidity]]). Hardly anything more is known about <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810166.png" />-rigidity of non-convex smooth closed surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810167.png" />. However, by Kuiper's theorem (1955), no smooth closed surface in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810168.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810169.png" />-isometrically rigid. A surprising tight four-dimensional manifold in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810170.png" /> is Kühnel's topological imbedding of the complex projective plane <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810171.png" /> into the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810172.png" />-skeleton of a simplex in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810173.png" /> [[#References|[a9]]]. The image is a triangulation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810174.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810175.png" /> vertices. | ||
| − | Taut imbeddings deserve a separate discussion. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810176.png" /> be a compact connected space. The given extrinsic definition of tautness for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810177.png" /> in the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810178.png" />-sphere <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810179.png" />, namely by the property that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810180.png" /> is tight in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810181.png" />, evidently determines (with the half-space definition of tight) the following intrinsic definition. The subspace <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810182.png" /> is taut in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810183.png" /> if the inclusion <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810184.png" /> is injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810185.png" />-homology for every (round) ball <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810186.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810187.png" />. By stereographic projection from a point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810188.png" /> into a Euclidean <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810189.png" />-space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810190.png" /> orthogonal to the vector <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810191.png" />, one obtains the following definition of tautness in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810192.png" />. A compact subspace <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810193.png" /> is taut if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810194.png" /> contains no open set of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810195.png" />, and the imbedding <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810196.png" /> is injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810197.png" />-homology, with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810198.png" /> a round ball or the complement of a round ball in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810199.png" />. Then taut implies tight. A taut subspace of the plane is either a circle or a round disc, from which an everywhere-dense union of disjoint open round discs is deleted (a | + | Taut imbeddings deserve a separate discussion. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810176.png" /> be a compact connected space. The given extrinsic definition of tautness for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810177.png" /> in the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810178.png" />-sphere <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810179.png" />, namely by the property that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810180.png" /> is tight in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810181.png" />, evidently determines (with the half-space definition of tight) the following intrinsic definition. The subspace <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810182.png" /> is taut in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810183.png" /> if the inclusion <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810184.png" /> is injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810185.png" />-homology for every (round) ball <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810186.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810187.png" />. By stereographic projection from a point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810188.png" /> into a Euclidean <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810189.png" />-space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810190.png" /> orthogonal to the vector <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810191.png" />, one obtains the following definition of tautness in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810192.png" />. A compact subspace <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810193.png" /> is taut if and only if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810194.png" /> contains no open set of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810195.png" />, and the imbedding <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810196.png" /> is injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810197.png" />-homology, with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810198.png" /> a round ball or the complement of a round ball in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810199.png" />. Then taut implies tight. A taut subspace of the plane is either a circle or a round disc, from which an everywhere-dense union of disjoint open round discs is deleted (a "limit Swiss cheese" ). Banchoff's plane Swiss cheese in Fig. eis tight but not taut, although every circle does cut it in at most two pieces, but one piece could be not injective in homology. While excluding exotic examples by an ANR-assumption, it is conjectured that every compact taut absolute neighbourhood retract in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810200.png" /> (cf. also [[Absolute retract for normal spaces|Absolute retract for normal spaces]]; [[Retract of a topological space|Retract of a topological space]]) is a smooth manifold. This is known for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810201.png" />. See [[#References|[a8]]]. |
The customary definitions of a smooth taut manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810202.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810203.png" /> are as follows: a) every non-degenerate distance function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810204.png" /> has <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810205.png" /> critical points; and b) every round ball in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810206.png" /> meets <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810207.png" /> in a subset that is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810208.png" />-homology injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810209.png" />. These definitions make sense and are also used for proper submanifolds of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810210.png" /> that are not necessarily compact (see [[#References|[a3]]]). But in that case tight is not defined and so tightness is not a consequence. | The customary definitions of a smooth taut manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810202.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810203.png" /> are as follows: a) every non-degenerate distance function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810204.png" /> has <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810205.png" /> critical points; and b) every round ball in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810206.png" /> meets <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810207.png" /> in a subset that is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810208.png" />-homology injective in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810209.png" />. These definitions make sense and are also used for proper submanifolds of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810210.png" /> that are not necessarily compact (see [[#References|[a3]]]). But in that case tight is not defined and so tightness is not a consequence. | ||
| Line 104: | Line 104: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R. Bott, H. Samelson, "Applications of the theory of Morse to symmetric spaces" ''Amer. J. Math.'' , '''80''' (1958) pp. 964–1029 {{MR|0105694}} {{ZBL|0101.39702}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> T.E. Cecil, S.S. Chern, "Tautness and Lie sphere geometry" ''Math. Ann.'' , '''278''' (1987) pp. 381–399 {{MR|0909233}} {{ZBL|0635.53029}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> T.E. Cecil, P.J. Ryan, "Tight and taut immersions of manifolds" , Pitman (1985) {{MR|0781126}} {{ZBL|0596.53002}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> S. Carter, A. West, "Isoparametric and totally focal submanifolds" ''Proc. London Math. Soc.'' , '''60''' (1990) pp. 609–624 {{MR|1044313}} {{ZBL|0663.53045}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> D. Ferus, H. Karcher, H.F. Münzer, "Clifford Algebren und neue isoparametrische Hyperflächen" ''Math. Z.'' , '''177''' (1981) pp. 479–502 {{MR|624227}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> W.Y. Hsiang, R.S. Palais, C.L. Terng, "The topology of isoparametric submanifolds" ''J. Diff. Geom.'' , '''27''' (1988) pp. 423–460 {{MR|0940113}} {{ZBL|0618.57018}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> N.H. Kuiper, "Tight embeddings and maps" W.Y. Hsiang (ed.) et al. (ed.) , ''The Chern Symposium (1979)'' , Springer (1980) pp. 97–145 {{MR|0609559}} {{ZBL|0461.53033}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> N.H. Kuiper, "Taut sets in three-space are very special" ''Topology'' , '''23''' (1984) pp. 323–336 {{MR|0770568}} {{ZBL|0578.53044}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> W. Kühnel, T. Banchoff, "The 9-vertex complex projective plane" ''The Math. Intelligencer'' , '''5''' : 3 (1983) pp. 11–22 {{MR|0737686}} {{ZBL|0534.51009}} </TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> N.H. Kuiper, W.F., III Meeks, "Total curvature for knotted surfaces" ''Invent. Math.'' , '''77''' (1984) pp. 25–69 {{MR|0751130}} {{ZBL|0553.53034}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> N.H. Kuiper, W.F. Pohl, "Tight topological embedding of the real projective plane in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092810/t092810250.png" />" ''Invent. Math.'' , '''42''' (1977) pp. 177–199 {{MR|0494122}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> H.-F. Münzner, "Isoparametrische Hyperflächen in Sphären II" ''Math. Ann.'' , '''256''' (1981) pp. 215–232 {{MR|0620709}} {{ZBL|0438.53050}} </TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> R. Miyaoka, T. Ozawa, "Construction of taut embeddings and the Cecil–Ryan conjecture" , ''Proc. 1988 Symp. Differential geometry'' , Acad. Press (1990)</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> T. Ozawa, "On critical sets of distance functions to a taut submanifold" ''Math. Ann.'' , '''276''' (1986) pp. 91–96 {{MR|0863709}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> U. Pinkall, "Tight surfaces and regular homotopy" ''Topology'' , '''25''' (1986) pp. 475–481 {{MR|0862434}} {{ZBL|0605.53027}} </TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> R.S. Palais, C.L. Terng, "Critical point theory and submanifold geometry" , ''Lect. notes in math.'' , '''1353''' , Springer (1988) {{MR|0972503}} {{ZBL|0658.49001}} </TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> U. Pinkall, G. Thorbergsson, "Taut 3-manifolds" ''Topology'' , '''28''' (1989) pp. 389–402 {{MR|1030983}} {{ZBL|0686.53050}} </TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> U. Pinkall, G. Thorbergsson, "Deformations of Dupin hypersurfaces" ''Proc. Amer. Math. Soc.'' , '''107''' (1989) pp. 1037–1043 {{MR|0975655}} {{ZBL|0682.53061}} </TD></TR><TR><TD valign="top">[a19]</TD> <TD valign="top"> G. Thorbergsson, "Tight analytic surfaces" ''Topology'' (Forthcoming) {{MR|1113686}} {{ZBL|0727.57031}} </TD></TR><TR><TD valign="top">[a20]</TD> <TD valign="top"> G. Thorbergsson, "Isoparametric foliations and their buildings" ''Ann. of Math.'' , '''31''' (1991) pp. 429–446 {{MR|1097244}} {{ZBL|0727.57028}} </TD></TR></table> |
Revision as of 17:02, 15 April 2012
The total absolute curvature  of an immersion of differentiability class
of an immersion of differentiability class  ,
,  ,
,  , of a closed connected
, of a closed connected  -manifold
-manifold  in Euclidean
in Euclidean  -space
-space  (cf. Immersion of a manifold) is expressed as an integral (a2) in terms of local invariants. It obeys
(cf. Immersion of a manifold) is expressed as an integral (a2) in terms of local invariants. It obeys
 | (a1) |
Here  is the infimum of
is the infimum of  for all immersions of
for all immersions of 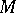 into Euclidean space or for some special class of immersions, such as a component of the space of smooth imbeddings, and
into Euclidean space or for some special class of immersions, such as a component of the space of smooth imbeddings, and  is the
is the  -th Betti number for Čech homology with
-th Betti number for Čech homology with  as coefficients (cf. Čech cohomology).
as coefficients (cf. Čech cohomology).
For a closed curve,  ,
,  is the curvature and
is the curvature and  the arc length. For a
the arc length. For a  surface
surface 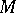 in
in  ,
, 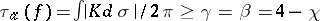 ,
, 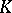 is the Gaussian curvature,
is the Gaussian curvature,  the area form, and
the area form, and  the Euler characteristic of
the Euler characteristic of 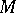 . An immersion
. An immersion  is called tight if
is called tight if  and if this
and if this  has minimal total absolute curvature
has minimal total absolute curvature  , the lowest bound being attained. If, moreover,
, the lowest bound being attained. If, moreover,  lies in the unit sphere
lies in the unit sphere  , then the immersion
, then the immersion  of
of 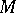 into
into  is called taut. A taut immersion is always an imbedding.
is called taut. A taut immersion is always an imbedding.
The general definition of  is as follows:
is as follows:
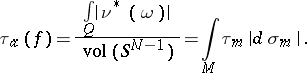 | (a2) |
Here  is the
is the 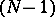 -manifold and bundle of unit normal vectors for
-manifold and bundle of unit normal vectors for  at
at  ,
,  is the natural projection by the Gauss mapping of
is the natural projection by the Gauss mapping of  into
into  ,
, 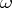 is the volume element, an
is the volume element, an  -form on
-form on  ,
, 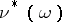 the pull-back on
the pull-back on 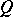 , and
, and  the volume element on
the volume element on  induced by the immersion into Euclidean space. The form
induced by the immersion into Euclidean space. The form  is called the Lipschitz–Killing form. The well-defined density
is called the Lipschitz–Killing form. The well-defined density  is the absolute Lipschitz–Killing density on
is the absolute Lipschitz–Killing density on  . For surfaces (in
. For surfaces (in  ),
),  . In general,
. In general, 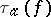 measures the area swept out by the normal vectors on the unit sphere of directions. Many homogeneous spaces, like
measures the area swept out by the normal vectors on the unit sphere of directions. Many homogeneous spaces, like  , all projective spaces and homogeneous Kähler manifolds, have tight (and even taut) imbeddings by their standard models in
, all projective spaces and homogeneous Kähler manifolds, have tight (and even taut) imbeddings by their standard models in  for some
for some 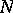 . (See below.)
. (See below.)
The main problem is existence. One is interested also in the special properties of tight immersions for a given manifold  .
.
Important is the following probabilistic definition:
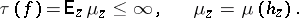 | (a3) |
Here 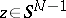 is a unit vector, the gradient of the linear function
is a unit vector, the gradient of the linear function 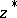 on
on  with
with 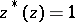 ,
,  is a "height function on a manifoldheight function" on
is a "height function on a manifoldheight function" on 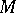 ,
,  is the number of non-degenerate critical points of
is the number of non-degenerate critical points of  , and
, and 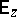 the expectation (or mean) value for
the expectation (or mean) value for 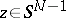 with respect to the standard invariant measure on
with respect to the standard invariant measure on  .
.
For smooth immersions one has
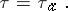 | (a4) |
The property 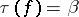 , another definition of tight, permits the application of Morse theory. The inequality (a1) is, in particular, a consequence of the Morse inequality
, another definition of tight, permits the application of Morse theory. The inequality (a1) is, in particular, a consequence of the Morse inequality  , which holds for almost-all
, which holds for almost-all  , as
, as  is non-degenerate for almost-all
is non-degenerate for almost-all  . It follows that an immersion is tight if every non-degenerate height function has
. It follows that an immersion is tight if every non-degenerate height function has 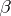 critical points. See Fig. cand Fig. d.
critical points. See Fig. cand Fig. d.

Figure: t092810a

Figure: t092810b

Figure: t092810c

Figure: t092810d
An imbedding of spaces 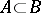 is called injective in
is called injective in  -homology if the induced homomorphism
-homology if the induced homomorphism  is injective for
is injective for  . Let
. Let  , with as boundary the hyperplane
, with as boundary the hyperplane  , be a half-space of
, be a half-space of 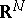 . For example,
. For example,
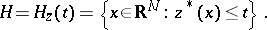 |
If  is a tight immersion and
is a tight immersion and  is a non-degenerate height function, then by Morse theory
is a non-degenerate height function, then by Morse theory 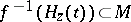 is injective in
is injective in 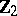 -homology. By continuity this injectivity then holds for every half-space
-homology. By continuity this injectivity then holds for every half-space 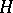 . For smooth immersions of closed manifolds this half-space property is equivalent to tightness. However, this half-space definition can be applied in the larger context of continuous immersions or even mappings of manifolds and other compact topological spaces into
. For smooth immersions of closed manifolds this half-space property is equivalent to tightness. However, this half-space definition can be applied in the larger context of continuous immersions or even mappings of manifolds and other compact topological spaces into  . An example is the tight "Swiss cheese" , an imbedded surface with boundary, see Fig. e. A tight mapping into
. An example is the tight "Swiss cheese" , an imbedded surface with boundary, see Fig. e. A tight mapping into  is also called a perfect function.
is also called a perfect function.

Figure: t092810e

Figure: t092810f
For curves and closed surfaces, the half-space property reduces to 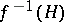 being connected for every half-space
being connected for every half-space 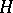 . Equivalent is Banchoff's two-piece property, which says that every hyperplane
. Equivalent is Banchoff's two-piece property, which says that every hyperplane  in
in  cuts
cuts 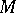 in at most two connected pieces. See the tight surfaces in Fig. c, Fig. d, and a non-tight curve in Fig. b.
in at most two connected pieces. See the tight surfaces in Fig. c, Fig. d, and a non-tight curve in Fig. b.
The half-space definition places tightness in classical geometry and convexity theory. Thus it follows that tightness is a projective property (cf. Projective geometry), as it is clearly invariant under any projective transformation in  that sends the convex hull
that sends the convex hull  into
into  . Tautness as defined above is a conformal property (cf. Conformal geometry). It is invariant under any conformal (Möbius) transformation of
. Tautness as defined above is a conformal property (cf. Conformal geometry). It is invariant under any conformal (Möbius) transformation of 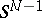 onto
onto  , which, in turn, is determined by a unique projective transformation in
, which, in turn, is determined by a unique projective transformation in  sending
sending 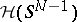 onto
onto  .
.
In proofs an important role is played by Kuiper's fundamental theorem. For imbeddings it says: Top sets of tightly imbedded spaces are tight. A top set is the intersection with a supporting half-space or hyperplane in  .
.
Miscellaneous representative theorems, mainly mentioned for surfaces, are as follows.
Curves.
A tight closed curve in  is plane and convex (W. Fenchel, 1929). The plane curve in Fig. a is not tight by every definition. A knotted curve in
is plane and convex (W. Fenchel, 1929). The plane curve in Fig. a is not tight by every definition. A knotted curve in  like the trefoil knot in Fig. bhas
like the trefoil knot in Fig. bhas 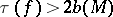 . Equality with the infimum
. Equality with the infimum  cannot be obtained (J. Milnor, 1950). Here
cannot be obtained (J. Milnor, 1950). Here  is the bridge index of the knot
is the bridge index of the knot 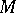 . It is the smallest number of maxima a height function can have on a knot
. It is the smallest number of maxima a height function can have on a knot  admitting isotopy of the knot (cf. Knot theory). The trefoil knot in Fig. ahas
admitting isotopy of the knot (cf. Knot theory). The trefoil knot in Fig. ahas 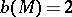 . On this knot every height function has at least two maxima but some height functions must have at least three by Milnor's bound above.
. On this knot every height function has at least two maxima but some height functions must have at least three by Milnor's bound above.
The first higher-dimensional theorem is due to S.S. Chern and J. Lashof (1957): A substantial (not in a hyper-plane) immersion of a closed  -manifold
-manifold 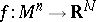 ,
,  , of differentiability class
, of differentiability class  with
with  , is a tight imbedding onto a convex hypersurface with
, is a tight imbedding onto a convex hypersurface with  . The same conclusion is known for a continuous immersion with suitably defined
. The same conclusion is known for a continuous immersion with suitably defined 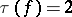 .
.
Surfaces.
If  is a non-orientable closed surface with Euler number
is a non-orientable closed surface with Euler number 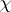 , then no tight immersion into
, then no tight immersion into  exists for
exists for 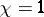 (projective plane) and
(projective plane) and  (Klein bottle, cf. Klein surface), not even a continuous immersion. The case
(Klein bottle, cf. Klein surface), not even a continuous immersion. The case  (projective plane with one handle) was an open problem since 1960. F. Haab proved (in 1990) that this surface has in fact no tight smooth immersion in Euclidean space
(projective plane with one handle) was an open problem since 1960. F. Haab proved (in 1990) that this surface has in fact no tight smooth immersion in Euclidean space 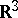 . So, for every smooth immersion there exists a plane which cuts it in at least three pieces. All other surfaces have tight immersions into
. So, for every smooth immersion there exists a plane which cuts it in at least three pieces. All other surfaces have tight immersions into 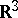 . A tight torus
. A tight torus 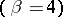 is depicted in Fig. cand a non-orientable tight surface with
is depicted in Fig. cand a non-orientable tight surface with  ,
, 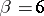 in Fig. d. The following theorems show that higher codimension and analyticity drastically restrict the possibility and nature of tight immersions. Also, differentiability is restrictive in comparison with continuous or piecewise-linear immersions.
in Fig. d. The following theorems show that higher codimension and analyticity drastically restrict the possibility and nature of tight immersions. Also, differentiability is restrictive in comparison with continuous or piecewise-linear immersions.
A smooth tight substantial closed surface in 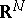 , for
, for 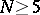 , is necessarily an (algebraic) Veronese surface (topologically a real projective plane, cf. also Veronese mapping) in
, is necessarily an (algebraic) Veronese surface (topologically a real projective plane, cf. also Veronese mapping) in  , unique up to projective transformations in
, unique up to projective transformations in  (N.H. Kuiper, 1960).
(N.H. Kuiper, 1960).
T. Banchoff (1965) suggested, however, and W. Kühnel (1980, see [a3]) proved, that except for the Klein bottle, a tight substantial polyhedral surface in 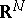 exists exactly for
exists exactly for  . This number is Heawood's chromatic number, known from the map-colour theorem. The same upper bound seems to hold for continuous tight immersions.
. This number is Heawood's chromatic number, known from the map-colour theorem. The same upper bound seems to hold for continuous tight immersions.
In this context there is another remarkable theorem. A substantial tight continuous immersion of the real projective plane into  ,
,  , is necessarily an imbedding into
, is necessarily an imbedding into  onto either the algebraic Veronese surface or onto Banchoff's six-vertex polyhedral surface [a11]. Every smooth immersion of a surface with
onto either the algebraic Veronese surface or onto Banchoff's six-vertex polyhedral surface [a11]. Every smooth immersion of a surface with  or
or  into
into  is regularly isotopic to a tight immersion (U. Pinkall, [a15]). For the other surfaces the results are not yet complete. Every orientable surface with
is regularly isotopic to a tight immersion (U. Pinkall, [a15]). For the other surfaces the results are not yet complete. Every orientable surface with  has a smooth substantial tight imbedding in
has a smooth substantial tight imbedding in 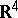 , but it
, but it
be analytic except for the torus (G. Thorbergsson, [a19]). Every smooth imbedded knotted orientable surface in  has total absolute curvature
has total absolute curvature  , and equality cannot be attained if genus
, and equality cannot be attained if genus 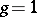 or
or  . For genus
. For genus 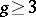 , however, Kuiper and W.F. Meeks [a10] proved that there do exist "isotopy-tight" knotted surfaces with
, however, Kuiper and W.F. Meeks [a10] proved that there do exist "isotopy-tight" knotted surfaces with  . An example of a knotted surface of genus
. An example of a knotted surface of genus  ,
,  is depicted in Fig. e. It is obtained from two linked tight tori by two connecting handles of non-positive Gaussian curvature
is depicted in Fig. e. It is obtained from two linked tight tori by two connecting handles of non-positive Gaussian curvature  . For this surface every non-degenerate height function has
. For this surface every non-degenerate height function has  critical points.
critical points.
Smooth immersions of surfaces in 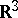 form a subclass of the smooth stable mappings
form a subclass of the smooth stable mappings 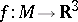 . In that class every surface has a tight stable mapping into
. In that class every surface has a tight stable mapping into  , hence with total absolute curvature equal to
, hence with total absolute curvature equal to
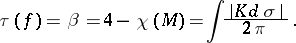 |
Tight analytic surfaces in 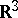 are isometrically rigid in the class of analytic surfaces (A.D. Aleksandrov, 1938; see [a3], p. 81, and Rigidity). Hardly anything more is known about
are isometrically rigid in the class of analytic surfaces (A.D. Aleksandrov, 1938; see [a3], p. 81, and Rigidity). Hardly anything more is known about  -rigidity of non-convex smooth closed surfaces in
-rigidity of non-convex smooth closed surfaces in 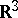 . However, by Kuiper's theorem (1955), no smooth closed surface in
. However, by Kuiper's theorem (1955), no smooth closed surface in 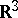 is
is  -isometrically rigid. A surprising tight four-dimensional manifold in
-isometrically rigid. A surprising tight four-dimensional manifold in  is Kühnel's topological imbedding of the complex projective plane
is Kühnel's topological imbedding of the complex projective plane  into the
into the  -skeleton of a simplex in
-skeleton of a simplex in  [a9]. The image is a triangulation of
[a9]. The image is a triangulation of 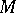 with
with 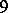 vertices.
vertices.
Taut imbeddings deserve a separate discussion. Let  be a compact connected space. The given extrinsic definition of tautness for
be a compact connected space. The given extrinsic definition of tautness for 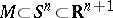 in the
in the  -sphere
-sphere  , namely by the property that
, namely by the property that  is tight in
is tight in  , evidently determines (with the half-space definition of tight) the following intrinsic definition. The subspace
, evidently determines (with the half-space definition of tight) the following intrinsic definition. The subspace 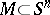 is taut in
is taut in  if the inclusion
if the inclusion  is injective in
is injective in  -homology for every (round) ball
-homology for every (round) ball 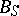 in
in 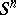 . By stereographic projection from a point
. By stereographic projection from a point 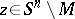 into a Euclidean
into a Euclidean  -space
-space 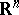 orthogonal to the vector
orthogonal to the vector  , one obtains the following definition of tautness in
, one obtains the following definition of tautness in 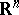 . A compact subspace
. A compact subspace 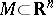 is taut if and only if
is taut if and only if  contains no open set of
contains no open set of  , and the imbedding
, and the imbedding  is injective in
is injective in  -homology, with
-homology, with 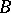 a round ball or the complement of a round ball in
a round ball or the complement of a round ball in 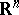 . Then taut implies tight. A taut subspace of the plane is either a circle or a round disc, from which an everywhere-dense union of disjoint open round discs is deleted (a "limit Swiss cheese" ). Banchoff's plane Swiss cheese in Fig. eis tight but not taut, although every circle does cut it in at most two pieces, but one piece could be not injective in homology. While excluding exotic examples by an ANR-assumption, it is conjectured that every compact taut absolute neighbourhood retract in
. Then taut implies tight. A taut subspace of the plane is either a circle or a round disc, from which an everywhere-dense union of disjoint open round discs is deleted (a "limit Swiss cheese" ). Banchoff's plane Swiss cheese in Fig. eis tight but not taut, although every circle does cut it in at most two pieces, but one piece could be not injective in homology. While excluding exotic examples by an ANR-assumption, it is conjectured that every compact taut absolute neighbourhood retract in  (cf. also Absolute retract for normal spaces; Retract of a topological space) is a smooth manifold. This is known for
(cf. also Absolute retract for normal spaces; Retract of a topological space) is a smooth manifold. This is known for  . See [a8].
. See [a8].
The customary definitions of a smooth taut manifold  in
in  are as follows: a) every non-degenerate distance function
are as follows: a) every non-degenerate distance function 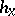 has
has 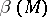 critical points; and b) every round ball in
critical points; and b) every round ball in 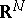 meets
meets 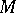 in a subset that is
in a subset that is  -homology injective in
-homology injective in  . These definitions make sense and are also used for proper submanifolds of
. These definitions make sense and are also used for proper submanifolds of 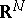 that are not necessarily compact (see [a3]). But in that case tight is not defined and so tightness is not a consequence.
that are not necessarily compact (see [a3]). But in that case tight is not defined and so tightness is not a consequence.
For a smooth proper submanifold  in
in  , the customary requirement of tautness is a very strong condition. The only taut closed surfaces in
, the customary requirement of tautness is a very strong condition. The only taut closed surfaces in  are, up to a Möbius transformation, the following: homogeneous spaces, the round
are, up to a Möbius transformation, the following: homogeneous spaces, the round  , the standard torus
, the standard torus  (
( and
and  are radii), and the standard Veronese surface (projective plane) in
are radii), and the standard Veronese surface (projective plane) in 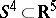 . Each of these models is a homogeneous space by motions of
. Each of these models is a homogeneous space by motions of 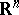 . Taut tori in
. Taut tori in  are Dupin cyclides (cf. Dupin cyclide). The diffeomorphism classes of all taut
are Dupin cyclides (cf. Dupin cyclide). The diffeomorphism classes of all taut  -manifolds in Euclidean spaces were found in [a17].
-manifolds in Euclidean spaces were found in [a17].
T. Ozawa [a14] proved that every connected set of critical points of a distance function  or
or  on a closed taut manifold
on a closed taut manifold 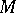 is itself a taut submanifold. The manifold
is itself a taut submanifold. The manifold  then contains many low-dimensional taut submanifolds, like circles, and tends to be special for this reason. Tautness plays an important role in differential geometry, in the study of the following kinds of spaces.
then contains many low-dimensional taut submanifolds, like circles, and tends to be special for this reason. Tautness plays an important role in differential geometry, in the study of the following kinds of spaces.
1) Orbits of isotropy representations of symmetric spaces, also called  -spaces (Kobayashi–Takeuchi), are taut submanifolds. They are, of course, homogeneous spaces and their cohomology was computed using (degenerate) tight height functions in a classic paper of R. Bott and H. Samelson [a1].
-spaces (Kobayashi–Takeuchi), are taut submanifolds. They are, of course, homogeneous spaces and their cohomology was computed using (degenerate) tight height functions in a classic paper of R. Bott and H. Samelson [a1].
2) Closed isoparametric submanifolds. A compact submanifold 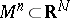 is called isoparametric if it has a flat normal bundle and the principal curvatures in the direction of any parallel normal vector field are constant. Then
is called isoparametric if it has a flat normal bundle and the principal curvatures in the direction of any parallel normal vector field are constant. Then 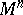 lies in a sphere
lies in a sphere  , but need not be homogeneous for codimension
, but need not be homogeneous for codimension  , [a5]. If
, [a5]. If 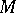 is irreducible with codimension
is irreducible with codimension  , then
, then  is an
is an 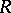 -space, [a20]. Isoparametric submanifolds are taut. They form a generalization of
-space, [a20]. Isoparametric submanifolds are taut. They form a generalization of  -spaces and their cohomology can likewise be calculated from their associated marked Dynkin diagrams ([a6]). The concepts of taut imbedding and isoparametric submanifold generalize to the Hilbert space setting [a16]. Examples are the infinite-dimensional flag manifolds. Finally, a remarkable result due to H.-F. Münzer [a12] is that for an isoparametric hypersurface
-spaces and their cohomology can likewise be calculated from their associated marked Dynkin diagrams ([a6]). The concepts of taut imbedding and isoparametric submanifold generalize to the Hilbert space setting [a16]. Examples are the infinite-dimensional flag manifolds. Finally, a remarkable result due to H.-F. Münzer [a12] is that for an isoparametric hypersurface  in a sphere
in a sphere  , the number of distinct principal curvatures must be
, the number of distinct principal curvatures must be 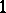 ,
,  ,
,  ,
,  , or
, or 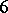 .
.
3) A submanifold  in
in 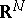 is called totally focal if every distance function
is called totally focal if every distance function  (
( ) either has on
) either has on 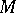 all critical points non-degenerate or all critical points degenerate. In combined efforts over several years of T.E. Cecil and P.J. Ryan, S. Carter and A. West [a4], the latter finally obtained the result that closed totally focal manifolds are the same as closed isoparametric submanifolds.
all critical points non-degenerate or all critical points degenerate. In combined efforts over several years of T.E. Cecil and P.J. Ryan, S. Carter and A. West [a4], the latter finally obtained the result that closed totally focal manifolds are the same as closed isoparametric submanifolds.
Note that any Möbius transform, or stereographic projection, or tubular  -neighbourhood boundary of a taut submanifold, like those mentioned above, is taut. Tautness is also invariant under the group of Lie sphere transformations, which contains the Möbius group as a subgroup [a2]. The product of two taut imbeddings is taut, and cylinders and surfaces of revolution built from taut imbeddings are taut (see [a3] and [a15]). All closed taut submanifolds that are now known (1990) have been obtained by these and some other new constructions (see [a18] and [a13]). Perhaps these exhaust all possibilities. For a wealth of other results and generalizations see the references.
-neighbourhood boundary of a taut submanifold, like those mentioned above, is taut. Tautness is also invariant under the group of Lie sphere transformations, which contains the Möbius group as a subgroup [a2]. The product of two taut imbeddings is taut, and cylinders and surfaces of revolution built from taut imbeddings are taut (see [a3] and [a15]). All closed taut submanifolds that are now known (1990) have been obtained by these and some other new constructions (see [a18] and [a13]). Perhaps these exhaust all possibilities. For a wealth of other results and generalizations see the references.
References
| [a1] | R. Bott, H. Samelson, "Applications of the theory of Morse to symmetric spaces" Amer. J. Math. , 80 (1958) pp. 964–1029 MR0105694 Zbl 0101.39702 |
| [a2] | T.E. Cecil, S.S. Chern, "Tautness and Lie sphere geometry" Math. Ann. , 278 (1987) pp. 381–399 MR0909233 Zbl 0635.53029 |
| [a3] | T.E. Cecil, P.J. Ryan, "Tight and taut immersions of manifolds" , Pitman (1985) MR0781126 Zbl 0596.53002 |
| [a4] | S. Carter, A. West, "Isoparametric and totally focal submanifolds" Proc. London Math. Soc. , 60 (1990) pp. 609–624 MR1044313 Zbl 0663.53045 |
| [a5] | D. Ferus, H. Karcher, H.F. Münzer, "Clifford Algebren und neue isoparametrische Hyperflächen" Math. Z. , 177 (1981) pp. 479–502 MR624227 |
| [a6] | W.Y. Hsiang, R.S. Palais, C.L. Terng, "The topology of isoparametric submanifolds" J. Diff. Geom. , 27 (1988) pp. 423–460 MR0940113 Zbl 0618.57018 |
| [a7] | N.H. Kuiper, "Tight embeddings and maps" W.Y. Hsiang (ed.) et al. (ed.) , The Chern Symposium (1979) , Springer (1980) pp. 97–145 MR0609559 Zbl 0461.53033 |
| [a8] | N.H. Kuiper, "Taut sets in three-space are very special" Topology , 23 (1984) pp. 323–336 MR0770568 Zbl 0578.53044 |
| [a9] | W. Kühnel, T. Banchoff, "The 9-vertex complex projective plane" The Math. Intelligencer , 5 : 3 (1983) pp. 11–22 MR0737686 Zbl 0534.51009 |
| [a10] | N.H. Kuiper, W.F., III Meeks, "Total curvature for knotted surfaces" Invent. Math. , 77 (1984) pp. 25–69 MR0751130 Zbl 0553.53034 |
| [a11] | N.H. Kuiper, W.F. Pohl, "Tight topological embedding of the real projective plane in 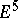 " Invent. Math. , 42 (1977) pp. 177–199 MR0494122 " Invent. Math. , 42 (1977) pp. 177–199 MR0494122 |
| [a12] | H.-F. Münzner, "Isoparametrische Hyperflächen in Sphären II" Math. Ann. , 256 (1981) pp. 215–232 MR0620709 Zbl 0438.53050 |
| [a13] | R. Miyaoka, T. Ozawa, "Construction of taut embeddings and the Cecil–Ryan conjecture" , Proc. 1988 Symp. Differential geometry , Acad. Press (1990) |
| [a14] | T. Ozawa, "On critical sets of distance functions to a taut submanifold" Math. Ann. , 276 (1986) pp. 91–96 MR0863709 |
| [a15] | U. Pinkall, "Tight surfaces and regular homotopy" Topology , 25 (1986) pp. 475–481 MR0862434 Zbl 0605.53027 |
| [a16] | R.S. Palais, C.L. Terng, "Critical point theory and submanifold geometry" , Lect. notes in math. , 1353 , Springer (1988) MR0972503 Zbl 0658.49001 |
| [a17] | U. Pinkall, G. Thorbergsson, "Taut 3-manifolds" Topology , 28 (1989) pp. 389–402 MR1030983 Zbl 0686.53050 |
| [a18] | U. Pinkall, G. Thorbergsson, "Deformations of Dupin hypersurfaces" Proc. Amer. Math. Soc. , 107 (1989) pp. 1037–1043 MR0975655 Zbl 0682.53061 |
| [a19] | G. Thorbergsson, "Tight analytic surfaces" Topology (Forthcoming) MR1113686 Zbl 0727.57031 |
| [a20] | G. Thorbergsson, "Isoparametric foliations and their buildings" Ann. of Math. , 31 (1991) pp. 429–446 MR1097244 Zbl 0727.57028 |
Tight and taut immersions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tight_and_taut_immersions&oldid=18311