Tertiary ideal
An ideal  of a ring
of a ring  which cannot be expressed as the intersection of a right fractional ideal
which cannot be expressed as the intersection of a right fractional ideal  and an ideal
and an ideal  , each strictly larger than
, each strictly larger than  . All irreducible ideals are tertiary. In Noetherian rings, tertiary ideals are the same as primary ideals (cf. Additive theory of ideals; Primary ideal; Primary decomposition).
. All irreducible ideals are tertiary. In Noetherian rings, tertiary ideals are the same as primary ideals (cf. Additive theory of ideals; Primary ideal; Primary decomposition).
Suppose that the ring  satisfies the maximum condition for left and right fractional ideals, and that every ideal decomposes as an intersection of finitely many indecomposable ideals. Then for every ideal
satisfies the maximum condition for left and right fractional ideals, and that every ideal decomposes as an intersection of finitely many indecomposable ideals. Then for every ideal  there exists a tertiary radical,
there exists a tertiary radical,  , the largest ideal
, the largest ideal  of
of 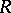 such that, for any ideal
such that, for any ideal  ,
,
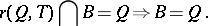 |
As for primary ideals, the intersection theorem, the existence theorem and the uniqueness theorem are true for tertiary ideals.
An analysis of the properties of left and right fractions (of ideals of a ring, of submodules of a module, and others) leads to systems with fractions in which the general notions of 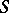 -primarity and
-primarity and  -primary radicals occur naturally. This allows one to formulate the "intersection" , "existence" and "uniqueness theorems" as axioms. In this approach, tertiarity is the unique notion of primarity for which all these three theorems hold, i.e. it is the unique "good" generalization of classical primarity (cf. [1], [2]).
-primary radicals occur naturally. This allows one to formulate the "intersection" , "existence" and "uniqueness theorems" as axioms. In this approach, tertiarity is the unique notion of primarity for which all these three theorems hold, i.e. it is the unique "good" generalization of classical primarity (cf. [1], [2]).
References
| [1] | V.A. Andrunakievich, Yu.M. Ryabukhin, "The additive theory of ideals in systems with residuals" Math. USSR Izv. , 1 : 5 (1967) pp. 1011–1040 Izv. Akad. Nauk SSSR Ser. Mat. , 31 : 5 (1967) pp. 1057–1090 |
| [2] | J.A. Riley, "Axiomatic primary and tertiary decomposition theory" Trans. Amer. Math. Soc. , 105 (1962) pp. 117–201 |
Tertiary ideal. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tertiary_ideal&oldid=13632