Symmetry on a set
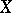
A non-negative real-valued function 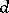 defined on the set of all pairs of elements of
defined on the set of all pairs of elements of 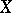 and satisfying the following axioms:
and satisfying the following axioms:
1)  if and only if
if and only if  ;
;
2)  for any
for any  .
.
In contrast to a metric and a pseudo-metric, a symmetry need not satisfy the triangle axiom. Relative to a symmetry  on a set
on a set 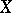 there is a topology defined on
there is a topology defined on 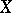 : A set
: A set 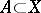 is closed (relative to
is closed (relative to 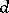 ) if and only if
) if and only if 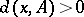 for each
for each 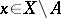 . Here
. Here
 |
The closure of a set 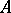 in this topological space contains the set of all
in this topological space contains the set of all 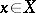 for which
for which 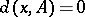 but need not be exhausted by this set. Correspondingly, the
but need not be exhausted by this set. Correspondingly, the  -ball around a point of
-ball around a point of 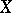 may have an empty interior. A topological space is called symmetrizable if its topology is generated by the above rule from some symmetry. The class of symmetrizable spaces is much wider than the class of metrizable spaces (cf. Metrizable space): A symmetrizable space need not be paracompact, normal or Hausdorff. In addition, a symmetrizable space need not satisfy the first axiom of countability.
may have an empty interior. A topological space is called symmetrizable if its topology is generated by the above rule from some symmetry. The class of symmetrizable spaces is much wider than the class of metrizable spaces (cf. Metrizable space): A symmetrizable space need not be paracompact, normal or Hausdorff. In addition, a symmetrizable space need not satisfy the first axiom of countability.
However, each symmetrizable space is sequential, that is, its topology is determined by convergent sequences by the rule: A set  is closed if and only if the limit of each sequence of points of
is closed if and only if the limit of each sequence of points of  that converges in
that converges in 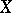 belongs to
belongs to 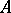 . For compact Hausdorff spaces symmetrizability is equivalent to metrizability.
. For compact Hausdorff spaces symmetrizability is equivalent to metrizability.
References
| [1] | A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) (Translated from Russian) |
| [2] | S.I. Nedev, " -metrizable spaces" Trans. Moscow Math. Soc. , 24 (1971) pp. 213–247 Trudy Moskov. Mat. Obshch. , 24 (1971) pp. 201–236 -metrizable spaces" Trans. Moscow Math. Soc. , 24 (1971) pp. 213–247 Trudy Moskov. Mat. Obshch. , 24 (1971) pp. 201–236 |
Symmetry on a set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Symmetry_on_a_set&oldid=14963