Super-group
Lie super-group
A group object in the category of super-manifolds (cf. Super-manifold). A super-group  is defined by a functor
is defined by a functor  from the category of commutative superalgebras into the category of groups. Lie's theorems (cf. Lie theorem) are transferred to super-groups, a fact that gives the correspondence between super-groups and finite-dimensional Lie superalgebras (cf. Superalgebra).
from the category of commutative superalgebras into the category of groups. Lie's theorems (cf. Lie theorem) are transferred to super-groups, a fact that gives the correspondence between super-groups and finite-dimensional Lie superalgebras (cf. Superalgebra).
Examples.
1) The super-group 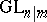 is defined by the functor
is defined by the functor  into groups of even invertible matrices from
into groups of even invertible matrices from  (see Super-space), i.e. of matrices in the form
(see Super-space), i.e. of matrices in the form
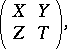 |
where  are invertible matrices of orders
are invertible matrices of orders  over
over  , while
, while 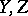 are matrices over
are matrices over  . A homomorphism
. A homomorphism 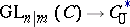 is defined by the formula
is defined by the formula
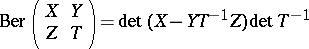 |
(the Berezinian);
2) 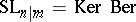 ;
;
3) 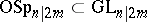 and
and 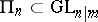 ; they leave invariant an even, or odd, non-degenerate symmetric bilinear form.
; they leave invariant an even, or odd, non-degenerate symmetric bilinear form.
To every super-group 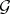 and super-subgroup
and super-subgroup  of it there is related a super-manifold
of it there is related a super-manifold 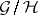 , represented by a functor
, represented by a functor  . This super-manifold is a homogeneous space of
. This super-manifold is a homogeneous space of 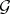 .
.
References
| [1] | Yu.I. Manin, "Gauge fields and complex geometry" , Springer (1988) (Translated from Russian) |
| [2] | F.A. Berezin, "Introduction to superanalysis" , Reidel (1987) (Translated from Russian) |
| [3] | D.A. Leites (ed.) , Seminar on supermanifolds , Kluwer (1990) |
Super-group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Super-group&oldid=17496