Subharmonic function
A function  of the points
of the points 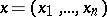 of a Euclidean space
of a Euclidean space  ,
, 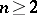 , defined in a domain
, defined in a domain 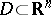 and possessing the following properties: 1)
and possessing the following properties: 1) 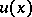 is upper semi-continuous in
is upper semi-continuous in  ; 2) for any point
; 2) for any point 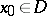 there exist values
there exist values 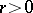 , arbitrarily small, such that
, arbitrarily small, such that
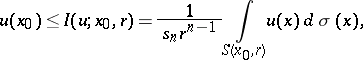 |
where  is the mean value of the function
is the mean value of the function 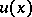 over the area of the sphere
over the area of the sphere 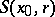 with centre
with centre  of radius
of radius  and
and 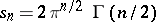 is the area of the unit sphere in
is the area of the unit sphere in 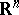 ; and 3)
; and 3) 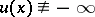 (this condition is sometimes dropped). In this definition of a subharmonic function, the mean value
(this condition is sometimes dropped). In this definition of a subharmonic function, the mean value 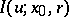 over the area of the sphere can be replaced by the mean value
over the area of the sphere can be replaced by the mean value
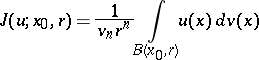 |
over the volume of the ball 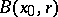 , where
, where 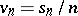 is the volume of the unit ball in
is the volume of the unit ball in 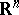 .
.
An equivalent definition of a subharmonic function, which explains the name "subharmonic function" is obtained by replacing condition 2) by 2'): If 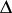 is a relatively-compact subdomain of
is a relatively-compact subdomain of  and
and 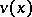 is a harmonic function in
is a harmonic function in  that is continuous on the closure
that is continuous on the closure  and is such that
and is such that
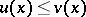 | (1) |
on the boundary  , then the inequality (1) holds everywhere in
, then the inequality (1) holds everywhere in  (
(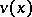 is called a harmonic majorant of the subharmonic function
is called a harmonic majorant of the subharmonic function 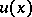 in
in 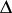 ). If the function
). If the function  belongs to the class
belongs to the class 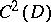 , then for it to be subharmonic in
, then for it to be subharmonic in  it is necessary and sufficient that the result of applying the Laplace operator,
it is necessary and sufficient that the result of applying the Laplace operator, 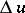 , be non-negative in
, be non-negative in 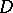 .
.
The idea of a subharmonic function was expounded in essence by H. Poincaré in the balayage method. Subharmonic functions are also found in the work of F. Hartogs [1] on the theory of analytic functions of several complex variables; the systematic study of subharmonic functions began with the work of F. Riesz . The close connection between subharmonic functions and analytic functions 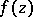 of one or several complex variables
of one or several complex variables 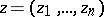 ,
, 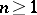 , and the consequent possible use of subharmonic functions for the study of analytic functions is related to the fact that the modulus
, and the consequent possible use of subharmonic functions for the study of analytic functions is related to the fact that the modulus 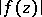 and the logarithm
and the logarithm  of the modulus of an analytic function are subharmonic functions. On the other hand, condition 2') shows that subharmonic functions can be considered as the analogue of convex functions of one real variable (cf. Convex function (of a real variable)).
of the modulus of an analytic function are subharmonic functions. On the other hand, condition 2') shows that subharmonic functions can be considered as the analogue of convex functions of one real variable (cf. Convex function (of a real variable)).
Simple properties of subharmonic functions.
1) If 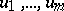 are subharmonic functions in
are subharmonic functions in 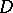 and
and  are non-negative numbers, then the linear combination
are non-negative numbers, then the linear combination 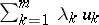 is a subharmonic function in
is a subharmonic function in  .
.
2) The upper envelope  of a finite family of subharmonic functions
of a finite family of subharmonic functions 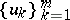 is a subharmonic function. If the upper envelope of an infinite family of subharmonic functions is upper semi-continuous, then it is also a subharmonic function.
is a subharmonic function. If the upper envelope of an infinite family of subharmonic functions is upper semi-continuous, then it is also a subharmonic function.
3) Uniformly-converging and monotone-decreasing sequences of subharmonic functions converge to subharmonic functions.
4) If  is a subharmonic function in
is a subharmonic function in 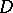 and
and 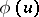 is a convex non-decreasing function on the domain of values
is a convex non-decreasing function on the domain of values 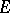 of the function
of the function  in
in 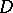 , or if
, or if  is a harmonic function in
is a harmonic function in  and
and 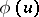 is a convex function on
is a convex function on 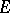 , then
, then 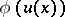 is a subharmonic function in
is a subharmonic function in  . In particular, if
. In particular, if 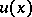 is a subharmonic function in
is a subharmonic function in 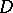 , then
, then 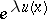 ,
, 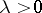 , and
, and 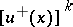 ,
, 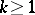 , where
, where 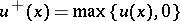 , are subharmonic functions in
, are subharmonic functions in 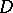 ; if
; if 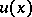 is a harmonic function in
is a harmonic function in  , then
, then 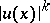 ,
,  , is a subharmonic function in
, is a subharmonic function in 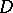 .
.
5) The maximum principle: If 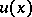 is a subharmonic function in
is a subharmonic function in 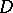 and for any boundary point
and for any boundary point 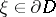 and any
and any 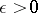 there is a neighbourhood
there is a neighbourhood 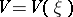 such that
such that 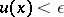 in
in 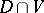 , then either
, then either 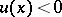 or
or  in
in 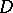 . This property also holds for unbounded domains
. This property also holds for unbounded domains  , where for
, where for 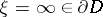 the neighbourhood
the neighbourhood 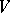 is taken to mean the exterior of a sphere,
is taken to mean the exterior of a sphere, 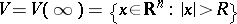 .
.
6) If 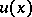 is a subharmonic function in a domain
is a subharmonic function in a domain 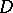 of the complex plane
of the complex plane 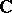 and
and  is a holomorphic mapping of a domain
is a holomorphic mapping of a domain 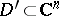 into
into  , then
, then  is a subharmonic function in
is a subharmonic function in 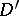 .
.
7) A function 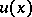 is harmonic in the domain
is harmonic in the domain 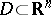 if and only if
if and only if 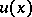 and
and 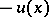 are subharmonic functions in
are subharmonic functions in 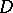 (cf. Harmonic function).
(cf. Harmonic function).
8) If 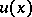 is a subharmonic function on the whole plane
is a subharmonic function on the whole plane 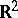 that is bounded above, then
that is bounded above, then 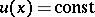 (in
(in 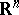 when
when 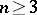 this property does not hold).
this property does not hold).
The Perron method for solving the Dirichlet problem for harmonic functions is based on the properties 2), 5) and 7).
The convexity properties of the mean values of subharmonic functions are of great importance: If  is a subharmonic function in an annulus
is a subharmonic function in an annulus  ,
, 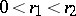 , then the mean values
, then the mean values 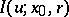 ,
,  , as well as the maximum
, as well as the maximum
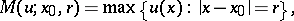 |
are convex functions in 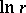 for
for 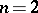 , or in
, or in 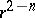 for
for 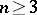 , on the interval
, on the interval 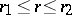 ; if
; if 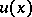 is a subharmonic function in the disc (ball)
is a subharmonic function in the disc (ball) 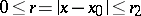 , then, moreover,
, then, moreover, 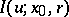 and
and 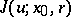 are continuous non-decreasing functions in
are continuous non-decreasing functions in  on the interval
on the interval 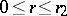 ; moreover
; moreover
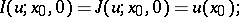 |
in the latter instance,
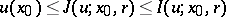 |
for 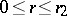 . The mean values
. The mean values  and
and 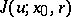 , considered as functions of the point
, considered as functions of the point 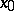 for fixed
for fixed  and
and  , are subharmonic functions in the corresponding subdomain
, are subharmonic functions in the corresponding subdomain  , and
, and  is continuous. By forming iterations of a sufficiently-high order,
is continuous. By forming iterations of a sufficiently-high order,
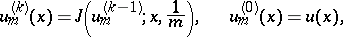 |
it is possible to obtain a monotone-decreasing sequence of subharmonic functions  of any degree of smoothness that converges, as
of any degree of smoothness that converges, as 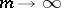 , to an arbitrarily given subharmonic function
, to an arbitrarily given subharmonic function  .
.
The Newton potential and logarithmic potential of non-negative masses, when written with a minus sign, are subharmonic functions everywhere in the space  . On the other hand, one of the basic theorems in the theory of subharmonic functions is the Riesz local representation theorem: An arbitrary subharmonic function can be represented as the sum of a harmonic function and a potential with a minus sign (see ). More precisely, if
. On the other hand, one of the basic theorems in the theory of subharmonic functions is the Riesz local representation theorem: An arbitrary subharmonic function can be represented as the sum of a harmonic function and a potential with a minus sign (see ). More precisely, if 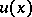 is a subharmonic function in a domain
is a subharmonic function in a domain  , then there exists a unique non-negative Borel measure
, then there exists a unique non-negative Borel measure 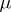 on
on 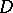 (a measure associated with
(a measure associated with 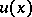 , or a Riesz measure) such that for any compact set
, or a Riesz measure) such that for any compact set  the representation
the representation
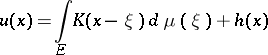 | (2) |
is valid, where  when
when 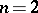 ,
, 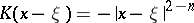 when
when 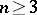 , and where
, and where 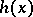 is a harmonic function in the interior of
is a harmonic function in the interior of 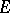 . The Riesz theorem establishes a close link between the theory of subharmonic functions and potential theory.
. The Riesz theorem establishes a close link between the theory of subharmonic functions and potential theory.
If 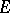 is a regular closed domain
is a regular closed domain 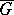 bounded, for example, by a Lyapunov surface, and having a Green function
bounded, for example, by a Lyapunov surface, and having a Green function  , then as well as (2) a representation using the Green potential is valid:
, then as well as (2) a representation using the Green potential is valid:
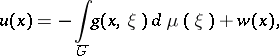 | (3) |
where 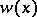 is the least harmonic majorant of the subharmonic function
is the least harmonic majorant of the subharmonic function 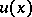 in the domain
in the domain  .
.
A representation in the form (3), generally speaking, does not hold in the whole domain of definition 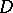 of
of 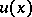 , and in the theory of subharmonic functions great importance is attached to the question of distinguishing the class of subharmonic functions
, and in the theory of subharmonic functions great importance is attached to the question of distinguishing the class of subharmonic functions 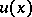 that allow a representation (3) in the whole domain
that allow a representation (3) in the whole domain 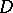 , i.e. the question of distinguishing the class
, i.e. the question of distinguishing the class 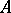 of subharmonic functions
of subharmonic functions 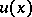 that have a harmonic majorant in the whole domain
that have a harmonic majorant in the whole domain  . For example, if
. For example, if 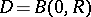 is a ball (disc) and there exists a constant
is a ball (disc) and there exists a constant 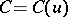 such that
such that
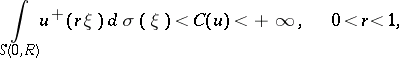 | (4) |
then 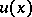 allows a representation (3) in
allows a representation (3) in 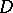 , and the least harmonic majorant
, and the least harmonic majorant 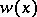 is, in turn, represented by a Poisson–Stieltjes integral:
is, in turn, represented by a Poisson–Stieltjes integral:
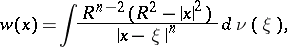 | (5) |
where  is a Borel measure of arbitrary sign concentrated on the boundary sphere (circle)
is a Borel measure of arbitrary sign concentrated on the boundary sphere (circle) 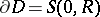 (a boundary measure) and normalized by the condition
(a boundary measure) and normalized by the condition 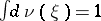 .
.
With regard to (5), it is important in practical applications to know under which conditions the boundary measure  has only a negative singular component, i.e. under which conditions the component
has only a negative singular component, i.e. under which conditions the component 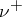 in the canonical decomposition
in the canonical decomposition 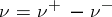 is absolutely continuous. This question is answered by introducing the class of strictly-subharmonic functions (see [11]–[13], [15], as well as [10], where generalizations are also examined). Let
is absolutely continuous. This question is answered by introducing the class of strictly-subharmonic functions (see [11]–[13], [15], as well as [10], where generalizations are also examined). Let 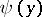 be an increasing concave function in
be an increasing concave function in 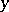 for which
for which 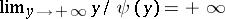 . A function
. A function 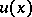 ,
, 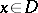 , is said to be strictly subharmonic relative to
, is said to be strictly subharmonic relative to 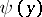 if
if 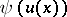 is a subharmonic function. For example, logarithmically-subharmonic functions (cf. Logarithmically-subharmonic function)
is a subharmonic function. For example, logarithmically-subharmonic functions (cf. Logarithmically-subharmonic function) 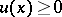 , for which
, for which 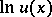 is a subharmonic function, belong to the class of strictly-subharmonic functions. If condition (4) is fulfilled for a strictly-subharmonic function
is a subharmonic function, belong to the class of strictly-subharmonic functions. If condition (4) is fulfilled for a strictly-subharmonic function 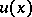 in the ball
in the ball 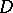 , then
, then  can be represented in the form (3) in
can be represented in the form (3) in 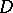 , and the boundary measure
, and the boundary measure  is characterized by the decomposition
is characterized by the decomposition
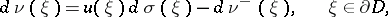 |
where 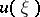 are the radial boundary values of the function
are the radial boundary values of the function 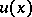 (which exist almost everywhere with respect to the Lebesgue measure on the sphere
(which exist almost everywhere with respect to the Lebesgue measure on the sphere 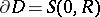 ), and
), and  is the singular component of the measure
is the singular component of the measure  .
.
Subharmonic functions of class  in the ball
in the ball 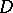 have radial boundary values almost everywhere on
have radial boundary values almost everywhere on 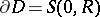 . However, examples have been constructed of bounded, continuous subharmonic functions in
. However, examples have been constructed of bounded, continuous subharmonic functions in 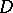 that do not have non-tangential boundary values anywhere on
that do not have non-tangential boundary values anywhere on 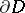 , a phenomenon that does not occur for harmonic functions. For non-tangential boundary values to exist, apart from (4) further conditions have to be imposed on the associated measure
, a phenomenon that does not occur for harmonic functions. For non-tangential boundary values to exist, apart from (4) further conditions have to be imposed on the associated measure 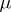 in
in 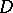 (see, for example, [14]).
(see, for example, [14]).
One of the essential questions in the theory of subharmonic functions and its applications is the characterization of the boundary properties of functions of different subclasses of the class 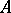 . The general method of introducing these subclasses consists of the fact that for strictly-subharmonic functions
. The general method of introducing these subclasses consists of the fact that for strictly-subharmonic functions 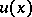 relative to a concave function
relative to a concave function 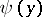 , any non-decreasing function
, any non-decreasing function  is examined that is convex relative to
is examined that is convex relative to 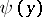 and that is such that
and that is such that 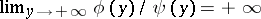 , and the class
, and the class 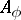 is introduced. For a sphere it is defined by the condition
is introduced. For a sphere it is defined by the condition
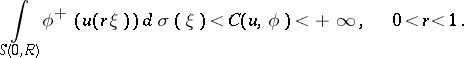 |
For the boundary properties of subharmonic functions, see [3]–[5], [10]–[16].
For functions that can be represented as the difference between two subharmonic functions, the concept of characteristic in the sense of R. Nevanlinna has been introduced, and the theory of functions of bounded characteristic (cf. Function of bounded characteristic) has been generalized (see [3], ).
A distinctive generalization of the theory of entire functions (cf. Entire function) is the theory of subharmonic functions in the whole space 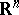 . Here, generalizations of the Weierstrass and Hadamard classical representation theorems of entire functions have been obtained, along with the theory of the growth and value distribution, the theory of asymptotic values and asymptotic paths, etc. (see ).
. Here, generalizations of the Weierstrass and Hadamard classical representation theorems of entire functions have been obtained, along with the theory of the growth and value distribution, the theory of asymptotic values and asymptotic paths, etc. (see ).
In the theory of analytic functions of several complex variables, the study of the subclasses of plurisubharmonic functions and pluriharmonic functions (cf. Plurisubharmonic function; Pluriharmonic function) is of considerable importance (see [17]). For axiomatic generalizations of subharmonic functions, see [9].
References
| [1] | F. Hartogs, "Zur Theorie der analytischen Funktionen mehrerer unabhängiger Veränderlichen, insbesondere über die Darstellung derselben durch Reihen welche nach Potenzen einer Veränderlichen fortschreiten" Math. Ann. , 62 (1906) pp. 1–88 |
| [2a] | F. Riesz, "Sur les fonctions sousharmoniques et leur rapport à la théorie du potentiel I" Acta Math. , 48 (1926) pp. 329–343 |
| [2b] | F. Riesz, "Sur les fonctions sousharmoniques et leur rapport à la théorie du potentiel II" Acta Math. , 54 (1930) pp. 321–360 |
| [3] | I.I. Privalov, "Subharmonic functions" , Moscow-Leningrad (1937) (In Russian) |
| [4] | I.I. Privalov, "Boundary properties of single-valued analytic functions" , Moscow (1941) (In Russian) |
| [5] | I.I. [I.I. Privalov] Priwalow, "Randeigenschaften analytischer Funktionen" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian) |
| [6] | T. Radó, "Subharmonic functions" , Springer (1937) |
| [7a] | W.K. Hayman, P.B. Kennedy, "Subharmonic functions" , 1 , Acad. Press (1976) |
| [7b] | W.K. Hayman, "Subharmonic functions" , 2 , Acad. Press (1989) |
| [8] | M. Brelot, "Etude des fonctions sousharmoniques au voisinage d'un point" , Hermann (1934) |
| [9] | M. Brélot, "Lectures on potential theory" , Tata Inst. (1967) |
| [10] | M. Heins, "Hardy classes on Riemann surfaces" , Springer (1969) |
| [11] | E.D. Solomentsev, "On some classes of subharmonic functions" Izv. Akad. Nauk SSSR , 5–6 (1938) pp. 571–582 (In Russian) |
| [12] | I.I. Privalov, P.I. Kuznetsov, "Sur les problèmes limites et les classes différentes de fonctions harmoniques et sousharmoniques définies dans une domaine arbitraire" Mat. Sb. , 6 : 3 (1939) pp. 345–376 (In Russian) (French abstract) |
| [13] | E.D. Solomentsev, "Classes of functions subharmonic on a half-space" Vestnik Moskov. Gos. Univ. Ser. Mat.-Mekh. Astron. : 5 (1959) pp. 73–91 (In Russian) |
| [14] | E.D. Solomentsev, "On boundary values of subharmonic functions" Czech. Math. J. , 8 : 4 (1958) pp. 520–536 (In Russian) (French abstract) |
| [15] | L. Gårding, L. Hormander, "Strongly subharmonic functions" Math. Scand. , 15 (1964) pp. 93–96 |
| [16] | E.D. Solomentsev, "Harmonic and subharmonic functions and their generalization" Itogi Nauk Mat. Anal. Teor. Veroyatnost. Regulirovanie 1962 (1964) pp. 83–100 (In Russian) |
| [17] | V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) (Translated from Russian) |
Comments
Axiomatic potential theory can be founded upon the properties 1) and 3), completed by some additional properties of the set 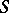 of negative subharmonic functions: a) the upper semi-continuous regularization of the supremum of a subset of
of negative subharmonic functions: a) the upper semi-continuous regularization of the supremum of a subset of 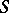 is subharmonic; b) (the Riesz decomposition property) for any
is subharmonic; b) (the Riesz decomposition property) for any 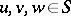 ,
, 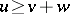 , there exist
, there exist 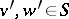 such that
such that 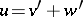 ,
, 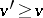 ,
, 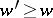 ; and c) any
; and c) any 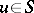 is the limit of a decreasing sequence in a sufficiently nice subcone of continuous subharmonic functions. See [a1].
is the limit of a decreasing sequence in a sufficiently nice subcone of continuous subharmonic functions. See [a1].
References
| [a1] | J. Bliedtner, W. Hansen, "Potential theory. An analytic and probabilistic approach to balayage" , Springer (1986) |
| [a2] | L.I. Ronkin, "Inroduction to the theory of entire functions of several variables" , Transl. Math. Monogr. , 44 , Amer. Math. Soc. (1974) (Translated from Russian) |
| [a3] | O.D. Kellogg, "Foundations of potential theory" , Dover, reprint (1954) pp. 315ff (Re-issue: Springer, 1967) |
Subharmonic function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Subharmonic_function&oldid=13674