Sequence of series
series scheme, serial scheme, triangular array
A double sequence of random variables 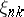 ,
, 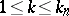 ,
, 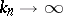 ,
, 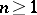 , in which
, in which 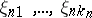 (forming the
(forming the  -th series) are mutually independent for any
-th series) are mutually independent for any  . The simplest sequence of series corresponds to the case
. The simplest sequence of series corresponds to the case 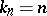 . In the limit theorems of probability theory, a fundamental problem is to determine the limiting behaviour, as
. In the limit theorems of probability theory, a fundamental problem is to determine the limiting behaviour, as 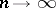 , of the distributions of the random variables
, of the distributions of the random variables
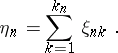 | (1) |
Under suitable conditions the class of limit distributions for such sequences coincides with the class of infinitely-divisible distributions (cf. Infinitely-divisible distribution). Suppose that the sequence of series 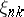 satisfies the condition of uniform asymptotic negligibility, that is,
satisfies the condition of uniform asymptotic negligibility, that is,
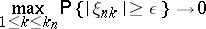 | (2) |
as 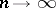 . Then
. Then 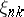 is said to form a null sequence of series. The set of distributions that are the limit distributions (in the sense of weak convergence) for the random variables (1), where
is said to form a null sequence of series. The set of distributions that are the limit distributions (in the sense of weak convergence) for the random variables (1), where 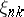 is a null sequence of series satisfying the condition of uniform asymptotic negligibility, coincides with the set of infinitely-divisible distributions.
is a null sequence of series satisfying the condition of uniform asymptotic negligibility, coincides with the set of infinitely-divisible distributions.
There are conditions for the convergence of the distributions of  to a given infinitely-divisible distribution (see [1]). In particular, the condition for convergence to a normal distribution has the following form.
to a given infinitely-divisible distribution (see [1]). In particular, the condition for convergence to a normal distribution has the following form.
Let 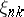 be a sequence of series, and let
be a sequence of series, and let 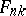 be the distribution function of
be the distribution function of  . For
. For  to satisfy (2), and for the distribution of the sums (2) to be weakly convergent to the normal distribution with parameters
to satisfy (2), and for the distribution of the sums (2) to be weakly convergent to the normal distribution with parameters  and
and 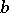 , it is necessary and sufficient that for any fixed
, it is necessary and sufficient that for any fixed 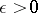 the following conditions hold:
the following conditions hold:
1) 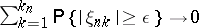 ,
,
2) 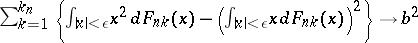 ,
,
3) 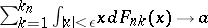 .
.
The study of limit distributions for the normalized partial sums of a sequence of independent random variables is a special case of the study of sequences of series.
As regards sequences of series see also: Infinitely-divisible distribution; Law of large numbers; and Limit theorems. For example, in classical versions of the central limit theorem and the law of large numbers, one considers special cases of sequences of series, formed by random variables
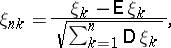 |
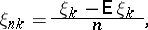 |
where the 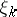 are independent random variables.
are independent random variables.
References
| [1] | B.V. Gnedenko, A.N. Kolmogorov, "Limit distributions for sums of independent random variables" , Addison-Wesley (1954) (Translated from Russian) |
| [2] | Yu.V. [Yu.V. Prokhorov] Prohorov, Yu.A. Rozanov, "Probability theory, basic concepts. Limit theorems, random processes" , Springer (1969) (Translated from Russian) |
| [3] | V.V. Petrov, "Sums of independent random variables" , Springer (1975) (Translated from Russian) |
| [4] | W. Feller, "An introduction to probability theory and its applications" , 2 , Wiley (1971) pp. Chapt. 1 |
Comments
See also Triangular array.
References
| [a1] | M. Loève, "Probability theory" , I , Springer (1977) |
Sequence of series. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Sequence_of_series&oldid=16669