Semi-group algebra

An algebra over a field with a basis 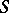that is at the same time a multiplicative semi-group. In particular, if 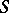is a group, one obtains a group algebra. If the semi-group contains a zero, this zero is usually identified with the zero of the algebra . The problem of describing all linear representations of a semi-group over a field (cf. Linear representation; Representation of a semi-group) is equivalent to that of describing all representations of the algebra . The importance of semi-group algebras in the theory of semi-groups is the possibility they offer of utilizing the richer tools of the theory of algebras to study linear representations of semi-groups. An example of this kind of result is: The algebra 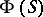of a finite semi-group 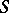is semi-simple if and only if all linear representations of the semi-group over the algebra are reducible.

References

[1]	A.H. Clifford, G.B. Preston, "Algebraic theory of semi-groups" , 1 , Amer. Math. Soc. (1961)

Comments

More precisely, let 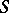be a semi-group and 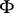a field. Consider the vector space of all formal finite sums 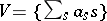, i.e. the vector space over 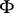with basis 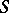. The semi-group multiplication extends linearly to define an algebra structure on 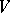. This is the semi-group algebra .

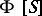If 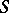is a semi-group with zero , the subspace 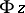is an ideal in 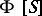and the contracted semi-group algebra 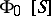is the quotient algebra .

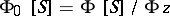For an inverse semi-group (cf. Inversion semi-group) one has the following analogue of Maschke's theorem (cf. Group algebra). The semi-group algebra 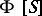of a finite inverse semi-group 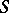is semi-simple if and only if the characteristic of is zero or is a prime that does not divided the order of any sub-semi-group of .