Semi-classical approximation
quasi-classical approximation
An asymptotic representation, that is, the asymptotics as 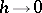 (where
(where 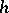 is Planck's constant), of the solutions of the equations of quantum mechanics. The Schrödinger equation
is Planck's constant), of the solutions of the equations of quantum mechanics. The Schrödinger equation
 | (1) |
describes the motion of a quantum-mechanical particle in a potential field 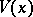 . The motion of a classical particle is described by the Hamilton–Jacobi equation (cf. Hamilton–Jacobi theory)
. The motion of a classical particle is described by the Hamilton–Jacobi equation (cf. Hamilton–Jacobi theory)
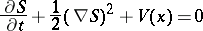 | (2) |
or by the Hamiltonian system
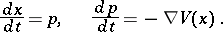 | (3) |
The Cauchy problem for the Schrödinger equation,
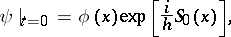 | (4) |
is compared with the Cauchy problem for the system (3):
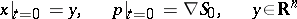 | (5) |
(here the functions 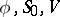 are smooth,
are smooth, 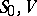 are real-valued and
are real-valued and 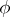 is of compact support). The asymptotics of the solution
is of compact support). The asymptotics of the solution 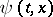 as
as 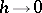 ,
, 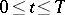 , and for small
, and for small 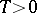 have the form:
have the form:
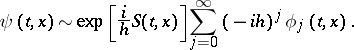 | (6) |
Here 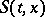 is the solution of (2) with Cauchy data
is the solution of (2) with Cauchy data 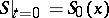 (the classical action), while
(the classical action), while
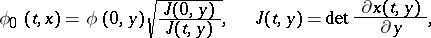 |
where 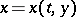 ,
, 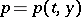 is the solution of (3), (5). The functions
is the solution of (3), (5). The functions 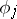 for
for 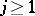 are defined from the recurrent system of transport equations (these are ordinary differential equations along the trajectories of the system (3)), so that all terms of the asymptotics are expressed in the terminology of classical mechanics. The Bohr correspondence principle asserts: If
are defined from the recurrent system of transport equations (these are ordinary differential equations along the trajectories of the system (3)), so that all terms of the asymptotics are expressed in the terminology of classical mechanics. The Bohr correspondence principle asserts: If 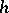 tends to zero, then the quantum laws must go over to the classical laws. The method of seeking asymptotics in the form (6) was proposed by P. Debye and has been widely applied in quantum mechanics.
tends to zero, then the quantum laws must go over to the classical laws. The method of seeking asymptotics in the form (6) was proposed by P. Debye and has been widely applied in quantum mechanics.
The asymptotic solution of the problem (1), (4) in the large (that is, for any finite time) is constructed via the canonical operator of V.P. Maslov [3]. The Cauchy data (5) fill out an  -dimensional Lagrangian manifold
-dimensional Lagrangian manifold 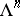 in the phase space
in the phase space 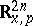 . Its translates
. Its translates  along the trajectories of the system (3) are also Lagrangian manifolds; their union
along the trajectories of the system (3) are also Lagrangian manifolds; their union 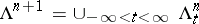 is an
is an  -dimensional Lagrangian manifold in the phase space
-dimensional Lagrangian manifold in the phase space 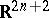 with coordinates
with coordinates 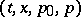 . For the canonical operator
. For the canonical operator 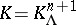 corresponding to
corresponding to 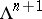 , the following commutation relation holds:
, the following commutation relation holds:
 | (7) |
where  is the derivative along the system (3) and
is the derivative along the system (3) and 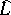 is the Schrödinger operator. The asymptotics of the solution
is the Schrödinger operator. The asymptotics of the solution 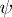 in the large are given by the formula
in the large are given by the formula
 | (8) |
where the functions 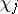 are defined from the Cauchy data (4) by means of the transport equations and can be expressed in terms of classical mechanics. At a non-focal point
are defined from the Cauchy data (4) by means of the transport equations and can be expressed in terms of classical mechanics. At a non-focal point 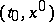 the asymptotics have the form
the asymptotics have the form
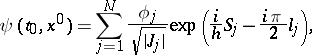 |
where the sum is taken over all rays arriving at this point, 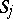 and
and 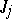 are the action and Jacobian for the
are the action and Jacobian for the 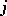 -th ray and
-th ray and 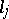 is the Morse index of the
is the Morse index of the 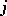 -th ray. For the stationary Schrödinger equation in the semi-classical approximation the problem of scattering has been studied, as well as the problem of the field of a point source, and the classical series (of Balmer type) of eigenvalues has been obtained.
-th ray. For the stationary Schrödinger equation in the semi-classical approximation the problem of scattering has been studied, as well as the problem of the field of a point source, and the classical series (of Balmer type) of eigenvalues has been obtained.
Semi-classical approximations in the wide sense (synonyms: high-frequency asymptotics, short-wave approximations, approximations of geometrical optics, WKB-method, eikonal method, quasi-classical approximations in the wide sense) are the asymptotics of solutions of partial differential equations with real characteristics of the form
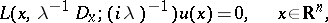 | (9) |
as well as of differential and pseudo-differential equations. Here  is a large parameter and the symbol
is a large parameter and the symbol 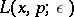 depends weakly on
depends weakly on  . Corresponding to (9) are the equations of classical mechanics, namely the Hamilton–Jacobi equation
. Corresponding to (9) are the equations of classical mechanics, namely the Hamilton–Jacobi equation
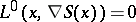 |
and the Hamiltonian system
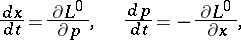 | (10) |
where 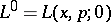 . The semi-classical approximation is constructed by means of the canonical operator corresponding to the Lagrangian manifolds that are invariant with respect to the dynamical system (10) and having a form similar to (8).
. The semi-classical approximation is constructed by means of the canonical operator corresponding to the Lagrangian manifolds that are invariant with respect to the dynamical system (10) and having a form similar to (8).
The semi-classical approximation is widely applied in modern physics in problems of the propagation of sound, elastic and electromagnetic waves, in non-relativistic and relativistic quantum mechanics and other questions.
References
| [1] | L. Brillouin, "La théorie des quanta et l'atome de Bohr" , Blanchard (1922) |
| [2] | L.D. Landau, E.M. Lifshitz, "Quantum mechanics" , Pergamon (1965) (Translated from Russian) |
| [3] | V.P. Maslov, "Théorie des perturbations et méthodes asymptotiques" , Dunod (1972) (Translated from Russian) |
| [4] | V.P. Maslov, M.V. Fedoryuk, "Quasi-classical approximation for the equations of quantum mechanics" , Reidel (1981) (Translated from Russian) |
| [5] | V.A. Fok, "Problems of diffraction and propagation of electromagnetic waves" , Moscow (1970) (In Russian) |
| [6] | V.M. Babich, V.S. Buldyrev, "Asymptotic methods in the diffraction of short waves" , Moscow (1972) (In Russian) (Translation forthcoming: Springer) |
| [7] | V.P. Maslov, "Operational methods" , MIR (1976) (Translated from Russian) |
Comments
References
| [a1] | B. Simon, "Functional integration and quantum mechanics" , Acad. Press (1979) |
Semi-classical approximation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Semi-classical_approximation&oldid=16814