Selberg sieve
Selberg method
A special, and at the same time fairly universal, sieve method created by A. Selberg [1]. The Selberg sieve enables one to obtain a good upper bound of the shifting function  , which denotes the number of elements of a set
, which denotes the number of elements of a set 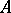 of integers that are not divisible by prime numbers
of integers that are not divisible by prime numbers 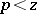 and that belong to a certain set
and that belong to a certain set 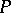 of prime numbers.
of prime numbers.
Let 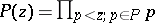 . The Selberg method is based on the obvious inequality
. The Selberg method is based on the obvious inequality
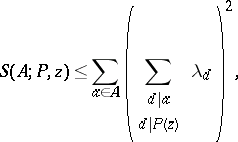 | (*) |
which holds when  for arbitrary real numbers
for arbitrary real numbers 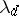 (
(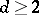 ). Selberg's idea consists of the following: Set
). Selberg's idea consists of the following: Set 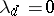 for
for 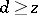 , and minimize the right-hand side of (*) by a suitable choice of the remaining numbers
, and minimize the right-hand side of (*) by a suitable choice of the remaining numbers 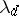 (
(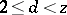 ).
).
When combined with other sieve methods, the Selberg sieve enables one to obtain lower bounds that are particularly powerful when used with weight functions.
References
| [1] | A. Selberg, "On an elementary method in the theory of primes" Norsk. Vid. Selsk. Forh. , 19 : 18 (1947) pp. 64–67 |
| [2] | K. Prachar, "Primzahlverteilung" , Springer (1957) |
| [3] | H. Halberstam, H.-E. Richert, "Sieve methods" , Acad. Press (1974) |
Selberg sieve. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Selberg_sieve&oldid=11878