Schwarz kernel
in the disc 
The function
 |
Let 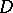 be a finite simply-connected or multiply-connected domain with boundary
be a finite simply-connected or multiply-connected domain with boundary 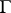 , let
, let 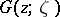 be the Green function for the Laplace operator in
be the Green function for the Laplace operator in 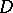 , and let the real-valued function
, and let the real-valued function 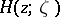 be the conjugate to
be the conjugate to  . Then the function
. Then the function 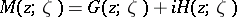 is called the complex Green function of the domain
is called the complex Green function of the domain 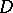 . The function
. The function 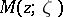 is an analytic but multiple-valued (if
is an analytic but multiple-valued (if 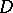 is multiply connected) function of
is multiply connected) function of  and a single-valued non-analytic function of
and a single-valued non-analytic function of  . The function
. The function
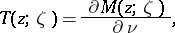 |
where  is the direction of the interior normal at
is the direction of the interior normal at 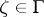 , is called the Schwarz kernel of
, is called the Schwarz kernel of  .
.
Let 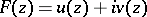 be an analytic function without singular points in
be an analytic function without singular points in 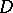 , and let
, and let  be single valued and continuous in
be single valued and continuous in 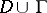 . Then the following formula holds:
. Then the following formula holds:
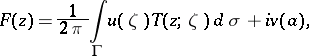 |
where 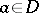 is a fixed point and
is a fixed point and  is the value at
is the value at  of one of the branches of the function
of one of the branches of the function 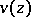 .
.
References
| [1] | P.P. Zabreiko (ed.) A.I. Koshelev (ed.) M.A. Krasnoselskii (ed.) S.G. Mikhlin (ed.) L.S. Rakovshchik (ed.) V.Ya. Stet'senko (ed.) T.O. Shaposhnikova (ed.) R.S. Anderssen (ed.) , Integral equations - a reference text , Noordhoff (1975) (Translated from Russian) |
| [2] | S.G. Mikhlin, "Linear integral equations" , Hindushtan Publ. Comp. , Delhi (1960) (Translated from Russian) |
Comments
Of course, some regularity conditions on 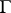 have to be assumed, so that the normal derivative
have to be assumed, so that the normal derivative 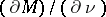 is well defined. Note that the real part of
is well defined. Note that the real part of 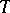 is the Poisson kernel.
is the Poisson kernel.
See also Schwarz integral.
Schwarz kernel. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Schwarz_kernel&oldid=16346