Regular function
From Encyclopedia of Mathematics
in a domain
A function 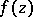 of a complex variable
of a complex variable  which is single-valued in this domain and which has a finite derivative at every point (see Analytic function). A regular function at a point
which is single-valued in this domain and which has a finite derivative at every point (see Analytic function). A regular function at a point  is a function that is regular in some neighbourhood of
is a function that is regular in some neighbourhood of  .
.
Comments
References
| [a1] | G. Springer, "Introduction to Riemann surfaces" , Addison-Wesley (1957) pp. 60; 169; 173 |
How to Cite This Entry:
Regular function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Regular_function&oldid=14473
Regular function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Regular_function&oldid=14473
This article was adapted from an original article by Yu.D. Maksimov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article