Reductio ad absurdum
From Encyclopedia of Mathematics
A logical derivation rule that allows one to conclude that if a list  of statements and a statement
of statements and a statement 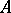 imply both a statement
imply both a statement 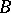 and the statement
and the statement  , then
, then  implies
implies 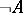 . The rule of reductio ad absurdum can, e.g., be written in the form
. The rule of reductio ad absurdum can, e.g., be written in the form
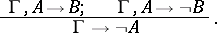 |
Reductio ad absurdum is a sound rule in the majority of logico-mathematical calculi.
Comments
Informally, the name "reductio ad absurdum" is also used for the rule that if  together with
together with  implies a contradiction, then
implies a contradiction, then  implies
implies  . This is of course equivalent to the above (and therefore sound) in classical logic, but it is not a sound rule of inference in intuitionistic logic.
. This is of course equivalent to the above (and therefore sound) in classical logic, but it is not a sound rule of inference in intuitionistic logic.
How to Cite This Entry:
Reductio ad absurdum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Reductio_ad_absurdum&oldid=16889
Reductio ad absurdum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Reductio_ad_absurdum&oldid=16889
This article was adapted from an original article by S.Yu. Maslov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article