Rationality theorems
for algebraic groups
Statements about the rationality (unirationality) or non-rationality of various algebraic group varieties (cf. Rational variety, Unirational variety). Since Abelian varieties can never be rational, the main interest is in rationality theorems for linear algebraic groups. Here, the rationality problem has two essentially different features: geometrical and arithmetical, according as whether the ground field $ K $ is algebraically closed or not. The first rationality theorems over the field $ \mathbf C $ of complex numbers were in fact proved by E. Picard and, in contemporary terminology, establish the unirationality of varieties of connected complex groups. The rationality problem for group varieties was clearly stated by C. Chevalley [1] as late as 1954. Progress in this direction is closely connected with achievements in the structure theory of algebraic groups. Thus, the Levi decomposition enables one to reduce the rationality problem to the case of reductive groups, and the Bruhat decomposition is the key to proving the rationality of varieties of reductive groups over any algebraically closed field (cf. Reductive group). Thus, in the geometrical case definitive results have been obtained.
The situation for algebraically non-closed fields $ K $ turns out to be much more complicated. Examples of non-rational $ K $- varieties are supplied by algebraic tori; for example, a three-dimensional torus $ T = R _{L/K} ^{(1)} ( G _{m} ) $, corresponding to the biquadratic extension $ L = K ( \sqrt a ,\ \sqrt b ) $ of $ K $( see [1]). This example is minimal, for tori of dimension $ \leq 2 $ are rational. Algebraic tori are always unirational. Arbitrary connected $ K $- groups are not necessarily unirational [3], but if $ K $ is perfect or $ G $ is reductive, unirationality can be proved (see [1]–[4]). Thus, the rationality problem for group varieties has the character of the Lüroth problem over an algebraically non-closed field.
Since an arbitrary reductive group is the almost-direct product of a torus and a semi-simple group, one can naturally distinguish two main cases: 1) $ G $ is a torus; or 2) $ G $ is a semi-simple group. The first case is investigated using various cohomological invariants (for semi-simple groups, these invariants turn out to be ineffective). Fairly complete results are obtained for tori which split over an Abelian extension of the ground field (see [5]). The first example of a non-rational variety in the class of semi-simple groups was a non-simply-connected group, whose construction is actually contained in [10]. The resulting conjecture, that varieties of simply-connected groups are always rational, was solved negatively by V.P. Platonov, using reduced $ K $- theory (see [6], [7]). It was found that the reduced Whitehead group $ S K _{1} (D) $ of a finite-dimensional central simple $ K $- algebra $ D $ is trivial if the variety determined by $ \mathop{\rm SL}\nolimits ( 1 ,\ D ) $ is rational over $ K $. These results carry over to unitary groups [12]. There are results on the rationality of the spinor varieties $ \mathop{\rm Spin}\nolimits ( n ,\ f \ ) $, where $ f $ is a non-degenerate quadratic form in $ n $ variables over $ K $( $ \mathop{\rm char}\nolimits \ K \neq 2 $). Spinor varieties are rational if either $ n \leq 5 $, or $ K $ is locally compact and non-discrete, or is the field of rational numbers (see [8], [9], [11]); for $ n \geq 6 $ there are spinor varieties that are not rational [8]. The last result is astonishing, in that $ \mathop{\rm Spin}\nolimits ( n ,\ f \ ) $ is a two-sheeted covering of the rational variety $ \mathop{\rm SO}\nolimits ( n ,\ f \ ) $.
The term "rationality theorem" is sometimes used in the theory of algebraic groups in a somewhat different sense, in connection with assertions about the properties of groups over a, not necessarily algebraically closed, field, such as the Rosenlicht–Grothendieck theorem, which states that any connected $ K $-
group possesses a maximal torus defined over $ K $(
see [4]).
References
| [1] | C. Chevalley, "On algebraic group varieties" J. Math. Soc. Japan , 6 : 3/4 (1954) pp. 303–324 MR0067122 Zbl 0057.26301 |
| [2] | M. Demazure, A. Grothendieck, "Schemas en groupes II" , Lect. notes in math. , 152 , Springer (1970) MR0274458 MR0274459 MR0274460 Zbl 0209.24201 |
| [3] | M. Rosenlicht, "Some rationality questions on algebraic groups" Ann. Mat. Pura Appl. , 43 (1957) pp. 25–50 MR0090101 Zbl 0079.25703 |
| [4] | A. Borel, "Linear algebraic groups" , Benjamin (1969) MR0251042 Zbl 0206.49801 Zbl 0186.33201 |
| [5] | V.E. Voskresenskii, "Algebraic tori" , Moscow (1977) (In Russian) MR0506279 MR0472845 Zbl 0379.14001 Zbl 0367.14007 |
| [6] | V.P. Platonov, "Reduced 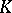 -theory and approximation in algebraic groups" Proc. Steklov Inst. Math. , 142 (1976) pp. 213–224 Trudy Mat. Inst. Steklov. , 142 (1976) pp. 198–207 MR568310 -theory and approximation in algebraic groups" Proc. Steklov Inst. Math. , 142 (1976) pp. 213–224 Trudy Mat. Inst. Steklov. , 142 (1976) pp. 198–207 MR568310 |
| [7] | V.P. Platonov, "Birational properties of the reduced Whitehead group" Dokl. Akad. Nauk BSSR , 21 : 3 (1977) pp. 197–198 (In Russian) MR0432603 Zbl 0791.20045 |
| [8] | V.P. Platonov, "On the problem of rationality of spinor varieties" Soviet Math. Dokl. , 20 : 5 (1979) pp. 1027–1031 Dokl. Akad. Nauk SSSR , 248 : 3 (1979) pp. 524–527 MR553481 Zbl 0435.20028 |
| [9] | V.P. Platonov, "Birational properties of spinor varieties" Proc. Steklov Inst. Math. , 157 (1981) pp. 173–182 Trudy Mat. Inst. Steklov. , 157 (1981) pp. 161–169 MR0651765 Zbl 0496.14008 |
| [10] | J.-P. Serre, "Cohomologie Galoisienne" , Springer (1964) MR0181643 Zbl 0143.05901 Zbl 0128.26303 |
| [11] | V.I. Chernousov, "On the rationality of spinor varieties over the rational number field" Dokl. Akad. Nauk BSSR , 25 : 4 (1981) pp. 293–296 (In Russian) (English abstract) MR0615460 |
| [12] | V.I. Yanchevskii, "Reduced  -theory. Applications to algebraic groups" Math. USSR Sb. , 38 : 4 (1981) pp. 533–548 Mat. Sb. , 110 : 4 (1979) pp. 579–596 MR1331389 MR0919253 MR0772116 MR0684770 MR0549289 MR0562210 MR0509375 MR0508832 -theory. Applications to algebraic groups" Math. USSR Sb. , 38 : 4 (1981) pp. 533–548 Mat. Sb. , 110 : 4 (1979) pp. 579–596 MR1331389 MR0919253 MR0772116 MR0684770 MR0549289 MR0562210 MR0509375 MR0508832 |
Comments
Unirationality results imply density results for rational points. For example, if $ G $ is a connected reductive group over an infinite field $ K $, the group $ G _{K} $ of $ K $- rational points is Zariski-dense in $ G $.
The Rosenlicht–Grothendieck theorem is often simply called the Grothendieck theorem.
Rationality theorems. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Rationality_theorems&oldid=44315