Rational homotopy theory
The natural setting of algebraic topology is the homotopy category. Restricting attention to simply-connected homotopy types and mappings between them allows the algebraic operation of localization (cf. Localization in categories). Inverting all the primes yields rational homotopy theory.
This theory was described algebraically by D. Quillen using differential Lie algebras modelling the loop space [a1]. It can also be described by differential algebras starting from a rational de Rham theory [a2]. A third discussion using the de Rham theory on the loop space and employing both of the first two descriptions was done by K.-T. Chen [a3].
One simple statement from all this is the following. Given a simply-connected compact manifold 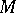 , let
, let 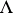 be a differential graded algebra mapping into the forms on
be a differential graded algebra mapping into the forms on 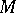 satisfying: i)
satisfying: i)  consists of the real field
consists of the real field 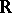 in degree zero and is free graded commutative in positive degrees; and ii) the mapping induces an isomorphism of real cohomology. Then: a) the homotopy groups of
in degree zero and is free graded commutative in positive degrees; and ii) the mapping induces an isomorphism of real cohomology. Then: a) the homotopy groups of 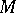 tensor
tensor  are naturally isomorphic to the dual of the indecomposable spaces of
are naturally isomorphic to the dual of the indecomposable spaces of 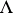 ; b) the differential yields the real form of the
; b) the differential yields the real form of the 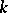 -invariants of the Postnikov system of
-invariants of the Postnikov system of  ; and c)
; and c)  is well defined up to an isomorphism of differential graded algebras.
is well defined up to an isomorphism of differential graded algebras.
The existence of 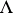 , mapping to the forms, the minimal model, can be shown by a simple inductive process. The above theory works almost verbatim for nilpotent spaces — those for which the fundamental group is nilpotent and the action of the fundamental group on the higher homotopy is nilpotent. The theory extends somehow to more general fundamental groups using representations coming from flat connections and minimal algebras more complicated than nilpotent ones. There are applications to Kähler manifolds [a2] and [a4].
, mapping to the forms, the minimal model, can be shown by a simple inductive process. The above theory works almost verbatim for nilpotent spaces — those for which the fundamental group is nilpotent and the action of the fundamental group on the higher homotopy is nilpotent. The theory extends somehow to more general fundamental groups using representations coming from flat connections and minimal algebras more complicated than nilpotent ones. There are applications to Kähler manifolds [a2] and [a4].
References
| [a1] | D. Quillen, "Rational homotopy theory" Ann. of Math. , 90 (1969) pp. 209–295 |
| [a2] | D. Sullivan, "Infinitesimal computations in topology" Publ. Math. IHES , 47 (1977) pp. 269–332 |
| [a3] | K.-T. Chen, "Iterated path integrals" Bulletin Amer. Math. Soc. , 83 (1977) pp. 831–879 |
| [a4] | J.W. Morgan, P.A. Griffiths, "Rational homotopy theory and differential forms" , Birkhäuser (1981) |
Rational homotopy theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Rational_homotopy_theory&oldid=16044