Ramanujan function
The function  , where
, where 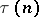 is the coefficient of
is the coefficient of 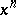 (
(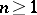 ) in the expansion of the product
) in the expansion of the product
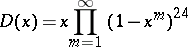 |
as a power series:
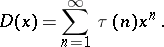 |
If one puts
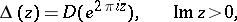 |
then the Ramanujan function is the  -th Fourier coefficient of the cusp form
-th Fourier coefficient of the cusp form 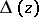 , which was first investigated by S. Ramanujan [1]. Certain values of the Ramanujan function:
, which was first investigated by S. Ramanujan [1]. Certain values of the Ramanujan function: 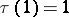 ,
, 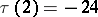 ,
, 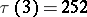 ,
, 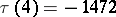 ,
, 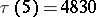 ,
,  ,
,  ,
, 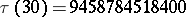 . Ramanujan conjectured (and L.J. Mordell proved) that the following properties of the Ramanujan function are true:
. Ramanujan conjectured (and L.J. Mordell proved) that the following properties of the Ramanujan function are true:
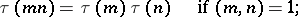 |
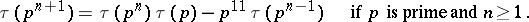 |
Consequently, the calculation of 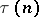 reduces to calculating
reduces to calculating 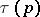 when
when 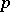 is prime. It is known that
is prime. It is known that 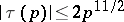 (see Ramanujan hypothesis). It is known that the Ramanujan function satisfies many congruence relations. For example, Ramanujan knew the congruence
(see Ramanujan hypothesis). It is known that the Ramanujan function satisfies many congruence relations. For example, Ramanujan knew the congruence
 |
Examples of congruence relations discovered later are:
 |
 |
etc.
References
| [1] | S. Ramanujan, "On certain arithmetical functions" Trans. Cambridge Philos. Soc. , 22 (1916) pp. 159–184 |
| [2] | J.-P. Serre, "Une interpretation des congruences relatives à la function  de Ramanujan" Sém. Delange–Pisot–Poitou (Théorie des nombres) , 9 : 14 (1967/68) pp. 1–17 de Ramanujan" Sém. Delange–Pisot–Poitou (Théorie des nombres) , 9 : 14 (1967/68) pp. 1–17 |
| [3] | O.M. Fomenko, "Applications of the theory of modular forms to number theory" J. Soviet Math. , 14 : 4 (1980) pp. 1307–1362 Itogi Nauk. i Tekhn. Algebra Topol. Geom. , 15 (1977) pp. 5–91 |
Comments
It is still (1990) not known whether there exists an 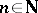 such that
such that 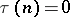 . One believes that the answer is "no" . For an elementary introduction to the background of
. One believes that the answer is "no" . For an elementary introduction to the background of 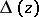 , see [a1].
, see [a1].
References
| [a1] | T.M. Apostol, "Modular functions and Dirichlet series in number theory" , Springer (1976) |
Ramanujan function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ramanujan_function&oldid=15321