Quadratic field
An extension of degree 2 of the field of rational numbers 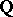 (cf. Extension of a field). Any quadratic field has the form
(cf. Extension of a field). Any quadratic field has the form 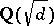 , where
, where 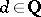 ,
,  , that is, it is obtained by adjoining
, that is, it is obtained by adjoining 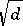 to
to 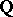 .
. 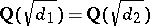 if and only if
if and only if 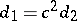 , where
, where 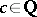 . Therefore any quadratic field has the form
. Therefore any quadratic field has the form 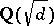 , where
, where 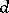 is a square-free integer that is uniquely determined by the field. In what follows,
is a square-free integer that is uniquely determined by the field. In what follows, 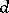 will always be taken to be this integer.
will always be taken to be this integer.
When 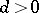 ,
, 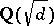 is called a real, and when
is called a real, and when  an imaginary, quadratic field.
an imaginary, quadratic field.
As a fundamental basis of 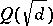 , that is, a basis of the ring of integers of the field
, that is, a basis of the ring of integers of the field 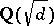 over the ring of rational integers
over the ring of rational integers 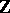 , one can take
, one can take
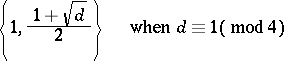 |
and
 |
The discriminant 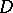 of
of  is equal to
is equal to 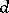 when
when 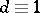 (
(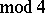 ) and to
) and to 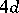 when
when 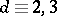 (
(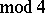 ).
).
Imaginary quadratic fields are the only type (apart from  ) with a finite unit group. This group has order 4 for
) with a finite unit group. This group has order 4 for 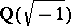 (and generator
(and generator 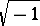 ), order 6 for
), order 6 for 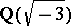 (and generator
(and generator 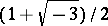 ), and order 2 (and generator
), and order 2 (and generator 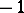 ) for all other imaginary quadratic fields.
) for all other imaginary quadratic fields.
For real quadratic fields the unit group is isomorphic to the direct product 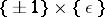 , where
, where 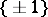 is the group of order 2 generated by
is the group of order 2 generated by  and
and  is the infinite cyclic group generated by a fundamental unit
is the infinite cyclic group generated by a fundamental unit  . For example, for
. For example, for  ,
, 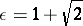 .
.
The factorization rule for rational prime divisors in a quadratic field has a simple formulation: Associated with 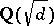 is a quadratic character
is a quadratic character 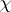 on
on 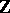 modulo
modulo 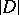 . If
. If 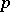 is a prime number and
is a prime number and 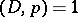 , then the divisor
, then the divisor 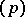 is prime in
is prime in  when
when 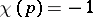 , and has two prime divisors when
, and has two prime divisors when 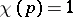 .
.
The divisor class group of quadratic fields has been studied more extensively than that of any other class of fields. For imaginary quadratic fields, the Brauer–Siegel theorem (stating that for algebraic number fields of fixed degree the following asymptotic formula holds:
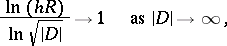 |
where 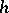 ,
, 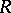 and
and 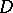 are, respectively, the class number, the regulator (cf. Regulator of an algebraic number field) and the discriminant of the field) shows that the class number tends to infinity as
are, respectively, the class number, the regulator (cf. Regulator of an algebraic number field) and the discriminant of the field) shows that the class number tends to infinity as  . There are exactly 9 imaginary quadratic fields of class number 1 (for
. There are exactly 9 imaginary quadratic fields of class number 1 (for 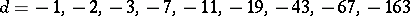 , see [2]). For real quadratic fields it is not known (1990) whether there is an infinite number of fields of class number 1. There are an infinite number of (both real and imaginary) quadratic fields whose class number is divisible by a given natural number (see [3], [4]). The analogous property for the
, see [2]). For real quadratic fields it is not known (1990) whether there is an infinite number of fields of class number 1. There are an infinite number of (both real and imaginary) quadratic fields whose class number is divisible by a given natural number (see [3], [4]). The analogous property for the  -primary component of the class group follows from Gauss' theory of genera.
-primary component of the class group follows from Gauss' theory of genera.
The theory of complex multiplication (see [5]) enables one to construct Abelian extensions of imaginary quadratic fields in an explicit form.
Many of the arithmetic properties of quadratic fields can be reformulated in terms of the theory of binary quadratic forms (cf. Binary quadratic form).
References
| [1] | Z.I. Borevich, I.R. Shafarevich, "Number theory" , Acad. Press (1987) (Translated from Russian) (German translation: Birkhäuser, 1966) |
| [2] | H.M. Stark, "A complete determination of the complex quadratic fields with class-number one" Michigan Math. J. , 14 (1967) pp. 1–27 |
| [3] | N.C. Ankeny, S. Chowla, "On the divisiblity of the class number of quadratic fields" Pacific J. Math. , 5 (1955) pp. 321–324 |
| [4] | Y. Yamamoto, "On umramified Galois extensions of quadratic number fields" Osaka J. Math. , 7 (1970) pp. 57–76 |
| [5] | J.W.S. Cassels (ed.) A. Fröhlich (ed.) , Algebraic number theory , Acad. Press (1967) pp. Chapt. 13 |
Comments
An effective version of the result 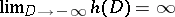 has recently been proved by B.H. Gross and D.B. Zagier [a1].
has recently been proved by B.H. Gross and D.B. Zagier [a1].
References
| [a1] | B.H. Gross, D.B. Zagier, "Heegner points and derivatives of 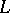 -series" Invent. Math. , 84 (1986) pp. 225–320 -series" Invent. Math. , 84 (1986) pp. 225–320 |
Quadratic field. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Quadratic_field&oldid=15229