Polynomial function

A generalization of the concept of an entire rational function (see Polynomial). Let be a unitary module over an associative-commutative ring with a unit. A mapping 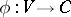is called a polynomial function if , where is a form of degree on , 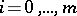(see Multilinear form). Most frequently, polynomial functions are considered when 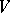is a free 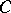-module (for example, a vector space over a field 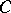) having a finite basis . 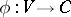Then the mapping is a polynomial function if and only if , where 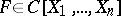is a polynomial over 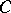and are the coordinates of an element 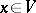in the basis . If here 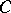is an infinite integral domain, the polynomial 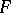is defined uniquely.

The polynomial functions on a module 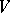form an associative-commutative 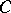-algebra 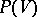with a unit with respect to the natural operations. If 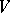is a free module with a finite basis over an infinite integral domain 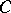, the algebra 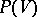is canonically isomorphic to the symmetric algebra 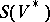of the adjoint (or dual) module , while if 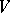is a finite-dimensional vector space over a field of characteristic 0, 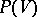is the algebra of symmetric multilinear forms on .

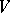Comments

E.g., polynomial functions on a Banach space naturally arise when one considers Taylor approximations to a differentiable function on such a space.